Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 116267 by bemath last updated on 02/Oct/20

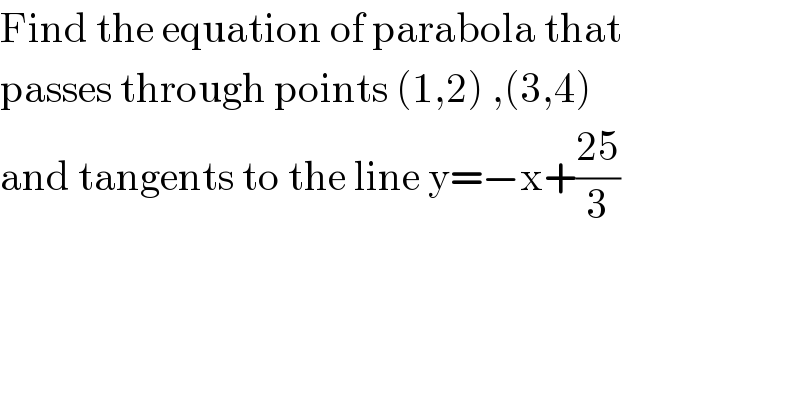
Find the equation of parabola that passes through points (1,2) ,(3,4) and tangents to the line y=−x+((25)/3)

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{parabola}\:\mathrm{that} \\ $$$$\mathrm{passes}\:\mathrm{through}\:\mathrm{points}\:\left(\mathrm{1},\mathrm{2}\right)\:,\left(\mathrm{3},\mathrm{4}\right)\: \\ $$$$\mathrm{and}\:\mathrm{tangents}\:\mathrm{to}\:\mathrm{the}\:\mathrm{line}\:\mathrm{y}=−\mathrm{x}+\frac{\mathrm{25}}{\mathrm{3}} \\ $$

Answered by bobhans last updated on 02/Oct/20

let the equation of parabola is y=ax^2 +bx+c →passes throught points { (((1,2)→2=a+b+c ...(i))),(((3,4)→4=9a+3b+c ...(ii))) :} substract eq (i) by (ii) ⇒2 = 8a+2b ; 1 = 4a+b ; b=1−4a since parabola tangent to the line y = −x+((25)/3), we get ax^2 +(1−4a)x+c=−x+((25)/3) ax^2 +(2−4a)x+c−((25)/3)= 0. Taking Δ = 4(1−2a)^2 −4a(c−((25)/3))=0 ⇒(1−2a)^2 = a(c−((25)/3)) ⇒ c = ((25)/3)+(((1−2a)^2 )/a); inserting to (i) ⇒2 = a+1−4a+((25)/3)+(((1−2a)^2 )/a) ⇒1 = ((25)/3)−3a+((1−4a+4a^2 )/a) ⇒−22=−9a+((3−12a+12a^2 )/a) ⇒−22a=−9a^2 +12a^2 −12a+3 ⇒3a^2 +10a+3 = 0 ⇒(3a+1)(a+3) = 0 for a=−(1/3)→ { ((b=1+(4/3)=(7/3))),((c=((25)/3)−3(1+(2/3))^2 = 0)) :} we get the equation of parabola is y= −(1/3)x^2 +(7/3)x for a=−3→ { ((b=13)),((c=((25)/3)−((49)/3)=−8)) :} we get the equation of parabola is y = −3x^2 +13x−8

$$\mathrm{let}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{parabola}\:\mathrm{is}\: \\ $$$$\mathrm{y}=\mathrm{ax}^{\mathrm{2}} +\mathrm{bx}+\mathrm{c}\:\rightarrow\mathrm{passes}\:\mathrm{throught}\:\mathrm{points} \\ $$$$\begin{cases}{\left(\mathrm{1},\mathrm{2}\right)\rightarrow\mathrm{2}=\mathrm{a}+\mathrm{b}+\mathrm{c}\:...\left(\mathrm{i}\right)}\\{\left(\mathrm{3},\mathrm{4}\right)\rightarrow\mathrm{4}=\mathrm{9a}+\mathrm{3b}+\mathrm{c}\:...\left(\mathrm{ii}\right)}\end{cases} \\ $$$$\mathrm{substract}\:\mathrm{eq}\:\left(\mathrm{i}\right)\:\mathrm{by}\:\left(\mathrm{ii}\right) \\ $$$$\Rightarrow\mathrm{2}\:=\:\mathrm{8a}+\mathrm{2b}\:;\:\mathrm{1}\:=\:\mathrm{4a}+\mathrm{b}\:;\:\mathrm{b}=\mathrm{1}−\mathrm{4a} \\ $$$$\mathrm{since}\:\mathrm{parabola}\:\mathrm{tangent}\:\mathrm{to}\:\mathrm{the}\:\mathrm{line}\: \\ $$$$\mathrm{y}\:=\:−\mathrm{x}+\frac{\mathrm{25}}{\mathrm{3}},\:\mathrm{we}\:\mathrm{get}\:\mathrm{ax}^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{4a}\right)\mathrm{x}+\mathrm{c}=−\mathrm{x}+\frac{\mathrm{25}}{\mathrm{3}} \\ $$$$\mathrm{ax}^{\mathrm{2}} +\left(\mathrm{2}−\mathrm{4a}\right)\mathrm{x}+\mathrm{c}−\frac{\mathrm{25}}{\mathrm{3}}=\:\mathrm{0}.\:\mathrm{Taking} \\ $$$$\Delta\:=\:\mathrm{4}\left(\mathrm{1}−\mathrm{2a}\right)^{\mathrm{2}} −\mathrm{4a}\left(\mathrm{c}−\frac{\mathrm{25}}{\mathrm{3}}\right)=\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{1}−\mathrm{2a}\right)^{\mathrm{2}} \:=\:\mathrm{a}\left(\mathrm{c}−\frac{\mathrm{25}}{\mathrm{3}}\right) \\ $$$$\Rightarrow\:\mathrm{c}\:=\:\frac{\mathrm{25}}{\mathrm{3}}+\frac{\left(\mathrm{1}−\mathrm{2a}\right)^{\mathrm{2}} }{\mathrm{a}};\:\mathrm{inserting}\:\mathrm{to}\:\left(\mathrm{i}\right) \\ $$$$\Rightarrow\mathrm{2}\:=\:\mathrm{a}+\mathrm{1}−\mathrm{4a}+\frac{\mathrm{25}}{\mathrm{3}}+\frac{\left(\mathrm{1}−\mathrm{2a}\right)^{\mathrm{2}} }{\mathrm{a}} \\ $$$$\Rightarrow\mathrm{1}\:=\:\frac{\mathrm{25}}{\mathrm{3}}−\mathrm{3a}+\frac{\mathrm{1}−\mathrm{4a}+\mathrm{4a}^{\mathrm{2}} }{\mathrm{a}} \\ $$$$\Rightarrow−\mathrm{22}=−\mathrm{9a}+\frac{\mathrm{3}−\mathrm{12a}+\mathrm{12a}^{\mathrm{2}} }{\mathrm{a}} \\ $$$$\Rightarrow−\mathrm{22a}=−\mathrm{9a}^{\mathrm{2}} +\mathrm{12a}^{\mathrm{2}} −\mathrm{12a}+\mathrm{3} \\ $$$$\Rightarrow\mathrm{3a}^{\mathrm{2}} +\mathrm{10a}+\mathrm{3}\:=\:\mathrm{0} \\ $$$$\Rightarrow\left(\mathrm{3a}+\mathrm{1}\right)\left(\mathrm{a}+\mathrm{3}\right)\:=\:\mathrm{0} \\ $$$$\mathrm{for}\:\mathrm{a}=−\frac{\mathrm{1}}{\mathrm{3}}\rightarrow\begin{cases}{\mathrm{b}=\mathrm{1}+\frac{\mathrm{4}}{\mathrm{3}}=\frac{\mathrm{7}}{\mathrm{3}}}\\{\mathrm{c}=\frac{\mathrm{25}}{\mathrm{3}}−\mathrm{3}\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} =\:\mathrm{0}}\end{cases} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{parabola}\:\mathrm{is} \\ $$$$\mathrm{y}=\:−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{7}}{\mathrm{3}}\mathrm{x} \\ $$$$\mathrm{for}\:\mathrm{a}=−\mathrm{3}\rightarrow\begin{cases}{\mathrm{b}=\mathrm{13}}\\{\mathrm{c}=\frac{\mathrm{25}}{\mathrm{3}}−\frac{\mathrm{49}}{\mathrm{3}}=−\mathrm{8}}\end{cases} \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{parabola}\:\mathrm{is} \\ $$$$\mathrm{y}\:=\:−\mathrm{3x}^{\mathrm{2}} +\mathrm{13x}−\mathrm{8}\: \\ $$$$ \\ $$

Commented by bemath last updated on 02/Oct/20

Commented by bemath last updated on 02/Oct/20

yes...correct

$$\mathrm{yes}...\mathrm{correct} \\ $$

Commented by mr W last updated on 02/Oct/20

generally there are infinitely many parabolas which fulfill the given conditions. there are two parabolas with axis in y direction. that′s what you got. there are two parabolas with axis in x direction. there are many other parabolas with skew axis.

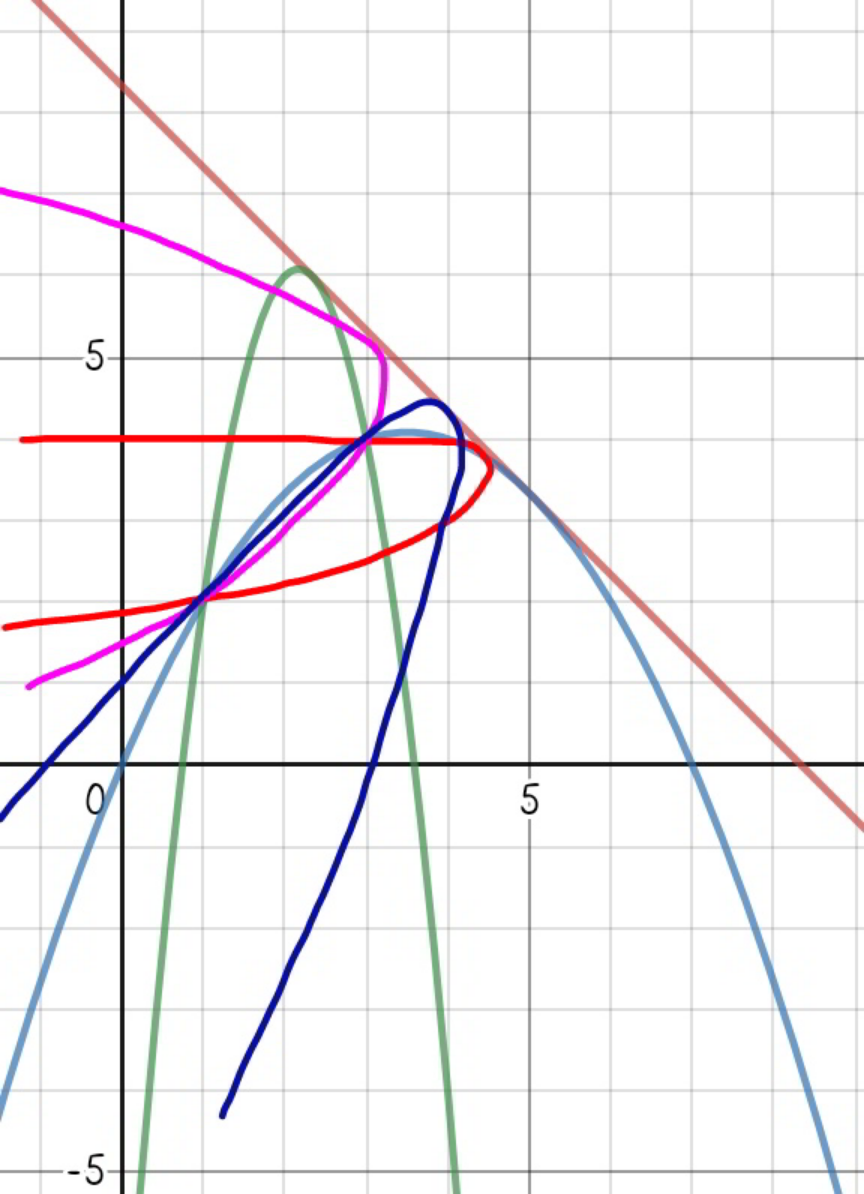
$${generally}\:{there}\:{are}\:{infinitely}\:{many} \\ $$$${parabolas}\:{which}\:{fulfill}\:{the}\:{given} \\ $$$${conditions}. \\ $$$${there}\:{are}\:{two}\:{parabolas}\:{with}\:{axis} \\ $$$${in}\:{y}\:{direction}.\:{that}'{s}\:{what}\:{you}\:{got}. \\ $$$${there}\:{are}\:{two}\:{parabolas}\:{with}\:{axis} \\ $$$${in}\:{x}\:{direction}. \\ $$$${there}\:{are}\:{many}\:{other}\:{parabolas}\:{with} \\ $$$${skew}\:{axis}. \\ $$

Commented by mr W last updated on 02/Oct/20

Commented by bemath last updated on 02/Oct/20

yes...santuyy...

$$\mathrm{yes}...\mathrm{santuyy}... \\ $$

Answered by 1549442205PVT last updated on 03/Oct/20

Dente the equation of the parabol we need find by (P): y=ax^2 +bx+c.Since the P pass through A(1,2)and B(3,4) we have: { ((a+b+c=2(1))),((9a+3b+c=4(2))) :} Substract (1)from(2)we get 8a+2b=2⇒b=1−4a,replace into (1) we get a+1−4a+c=2⇒c=3a+1 ⇒(P):y=ax^2 +(1−4a)x+3a+1 Since P touches to y=−x+((25)/3), infer : ax^2 +(1−4a)x+3a+1=−x+25/3 ⇔3ax^2 +2(3−6a)x+9a−22=0 has unique root⇔Δ′=(3−6a)^2 −3a(9a−22) =0⇔9a^2 +30a+9=0⇔(3a+5)^2 =16 ⇔3a+5=±4⇔a∈{−3,−1/3) we obtain two parabol are: i)y=−3x^2 +13x−8 ii)y=−(1/3)x^2 +(7/3)x

$$\mathrm{Dente}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{parabol}\:\mathrm{we} \\ $$$$\mathrm{need}\:\mathrm{find}\:\mathrm{by}\:\left(\mathrm{P}\right):\:\mathrm{y}=\mathrm{ax}^{\mathrm{2}} +\mathrm{bx}+\mathrm{c}.\mathrm{Since} \\ $$$$\mathrm{the}\:\mathrm{P}\:\mathrm{pass}\:\mathrm{through}\:\mathrm{A}\left(\mathrm{1},\mathrm{2}\right)\mathrm{and}\:\mathrm{B}\left(\mathrm{3},\mathrm{4}\right) \\ $$$$\mathrm{we}\:\mathrm{have}: \\ $$$$\begin{cases}{\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{2}\left(\mathrm{1}\right)}\\{\mathrm{9a}+\mathrm{3b}+\mathrm{c}=\mathrm{4}\left(\mathrm{2}\right)}\end{cases} \\ $$$$\mathrm{Substract}\:\left(\mathrm{1}\right)\mathrm{from}\left(\mathrm{2}\right)\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{8a}+\mathrm{2b}=\mathrm{2}\Rightarrow\mathrm{b}=\mathrm{1}−\mathrm{4a},\mathrm{replace}\:\mathrm{into}\:\left(\mathrm{1}\right) \\ $$$$\mathrm{we}\:\mathrm{get}\:\mathrm{a}+\mathrm{1}−\mathrm{4a}+\mathrm{c}=\mathrm{2}\Rightarrow\mathrm{c}=\mathrm{3a}+\mathrm{1} \\ $$$$\Rightarrow\left(\mathrm{P}\right):\mathrm{y}=\mathrm{ax}^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{4a}\right)\mathrm{x}+\mathrm{3a}+\mathrm{1} \\ $$$$\mathrm{Since}\:\mathrm{P}\:\mathrm{touches}\:\mathrm{to}\:\mathrm{y}=−\mathrm{x}+\frac{\mathrm{25}}{\mathrm{3}},\:\mathrm{infer}\:: \\ $$$$\mathrm{ax}^{\mathrm{2}} +\left(\mathrm{1}−\mathrm{4a}\right)\mathrm{x}+\mathrm{3a}+\mathrm{1}=−\mathrm{x}+\mathrm{25}/\mathrm{3}\: \\ $$$$\Leftrightarrow\mathrm{3ax}^{\mathrm{2}} +\mathrm{2}\left(\mathrm{3}−\mathrm{6a}\right)\mathrm{x}+\mathrm{9a}−\mathrm{22}=\mathrm{0}\:\mathrm{has} \\ $$$$\mathrm{unique}\:\mathrm{root}\Leftrightarrow\Delta'=\left(\mathrm{3}−\mathrm{6a}\right)^{\mathrm{2}} −\mathrm{3a}\left(\mathrm{9a}−\mathrm{22}\right) \\ $$$$=\mathrm{0}\Leftrightarrow\mathrm{9a}^{\mathrm{2}} +\mathrm{30a}+\mathrm{9}=\mathrm{0}\Leftrightarrow\left(\mathrm{3a}+\mathrm{5}\right)^{\mathrm{2}} =\mathrm{16} \\ $$$$\Leftrightarrow\mathrm{3a}+\mathrm{5}=\pm\mathrm{4}\Leftrightarrow\mathrm{a}\in\left\{−\mathrm{3},−\mathrm{1}/\mathrm{3}\right) \\ $$$$\mathrm{we}\:\mathrm{obtain}\:\mathrm{two}\:\mathrm{parabol}\:\mathrm{are}: \\ $$$$\left.\mathrm{i}\right)\mathrm{y}=−\mathrm{3x}^{\mathrm{2}} +\mathrm{13x}−\mathrm{8} \\ $$$$\left.\mathrm{ii}\right)\mathrm{y}=−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{7}}{\mathrm{3}}\mathrm{x} \\ $$

Commented by mr W last updated on 03/Oct/20

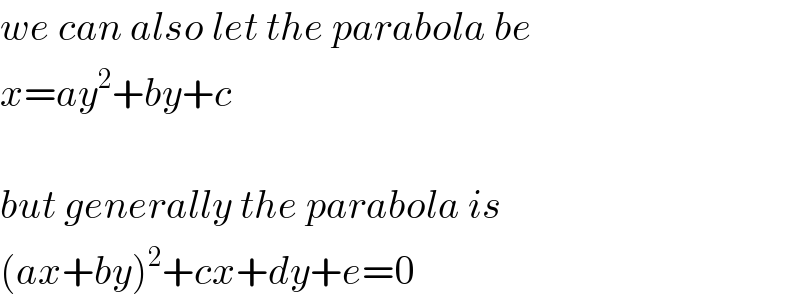
we can also let the parabola be x=ay^2 +by+c but generally the parabola is (ax+by)^2 +cx+dy+e=0

$${we}\:{can}\:{also}\:{let}\:{the}\:{parabola}\:{be} \\ $$$${x}={ay}^{\mathrm{2}} +{by}+{c} \\ $$$$ \\ $$$${but}\:{generally}\:{the}\:{parabola}\:{is} \\ $$$$\left({ax}+{by}\right)^{\mathrm{2}} +{cx}+{dy}+{e}=\mathrm{0} \\ $$

Commented by 1549442205PVT last updated on 07/Oct/20

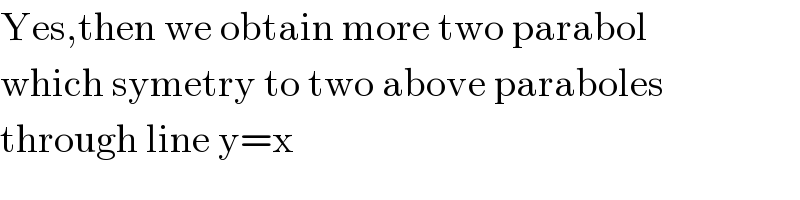
Yes,then we obtain more two parabol which symetry to two above paraboles through line y=x

$$\mathrm{Yes},\mathrm{then}\:\mathrm{we}\:\mathrm{obtain}\:\mathrm{more}\:\mathrm{two}\:\mathrm{parabol}\: \\ $$$$\mathrm{which}\:\mathrm{symetry}\:\mathrm{to}\:\mathrm{two}\:\mathrm{above}\:\mathrm{paraboles}\: \\ $$$$\mathrm{through}\:\mathrm{line}\:\mathrm{y}=\mathrm{x} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com