Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 14752 by Tinkutara last updated on 04/Jun/17

Find ordered pair of (x, y) given x, y ∈ [0, 2π] if 3^(sin x + cos y) = 1 and 5^(sin^2 x + cos^2 y) = 5

$$\mathrm{Find}\:\mathrm{ordered}\:\mathrm{pair}\:\mathrm{of}\:\left({x},\:{y}\right)\:\mathrm{given}\:{x},\:{y}\:\in \\ $$$$\left[\mathrm{0},\:\mathrm{2}\pi\right]\:\mathrm{if}\:\mathrm{3}^{\mathrm{sin}\:{x}\:+\:\mathrm{cos}\:{y}} \:=\:\mathrm{1}\:\mathrm{and} \\ $$$$\mathrm{5}^{\mathrm{sin}^{\mathrm{2}} \:{x}\:+\:\mathrm{cos}^{\mathrm{2}} \:{y}} \:=\:\mathrm{5} \\ $$

Commented by Tinkutara last updated on 04/Jun/17

Answer given is total 8 ordered pairs and there is no such pair as your answer.

$$\mathrm{Answer}\:\mathrm{given}\:\mathrm{is}\:\mathrm{total}\:\mathrm{8}\:\mathrm{ordered}\:\mathrm{pairs} \\ $$$$\mathrm{and}\:\mathrm{there}\:\mathrm{is}\:\mathrm{no}\:\mathrm{such}\:\mathrm{pair}\:\mathrm{as}\:\mathrm{your} \\ $$$$\mathrm{answer}. \\ $$

Commented by 1kanika# last updated on 04/Jun/17

Is ordered pair is (3π/4, 5π/4)

$$\mathrm{Is}\:\mathrm{ordered}\:\mathrm{pair}\:\mathrm{is}\:\left(\mathrm{3}\pi/\mathrm{4},\:\mathrm{5}\pi/\mathrm{4}\right) \\ $$

Commented by mrW1 last updated on 04/Jun/17

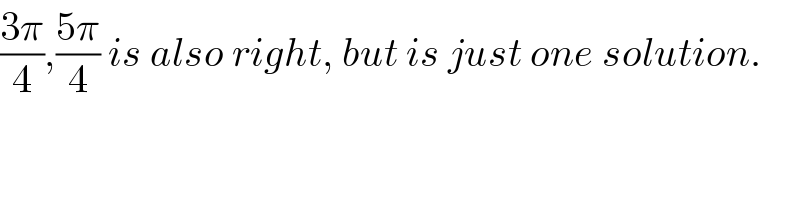
((3π)/4),((5π)/4) is also right, but is just one solution.

$$\frac{\mathrm{3}\pi}{\mathrm{4}},\frac{\mathrm{5}\pi}{\mathrm{4}}\:{is}\:{also}\:{right},\:{but}\:{is}\:{just}\:{one}\:{solution}. \\ $$

Commented by 1kanika# last updated on 04/Jun/17

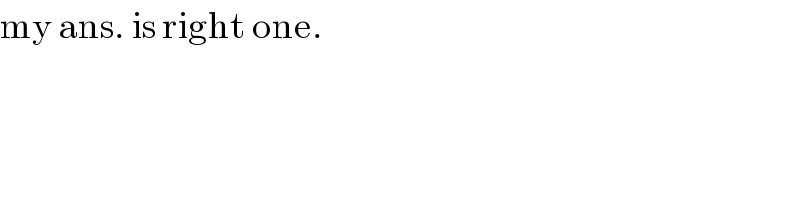
my ans. is right one.

$$\mathrm{my}\:\mathrm{ans}.\:\mathrm{is}\:\mathrm{right}\:\mathrm{one}. \\ $$

Answered by mrW1 last updated on 04/Jun/17

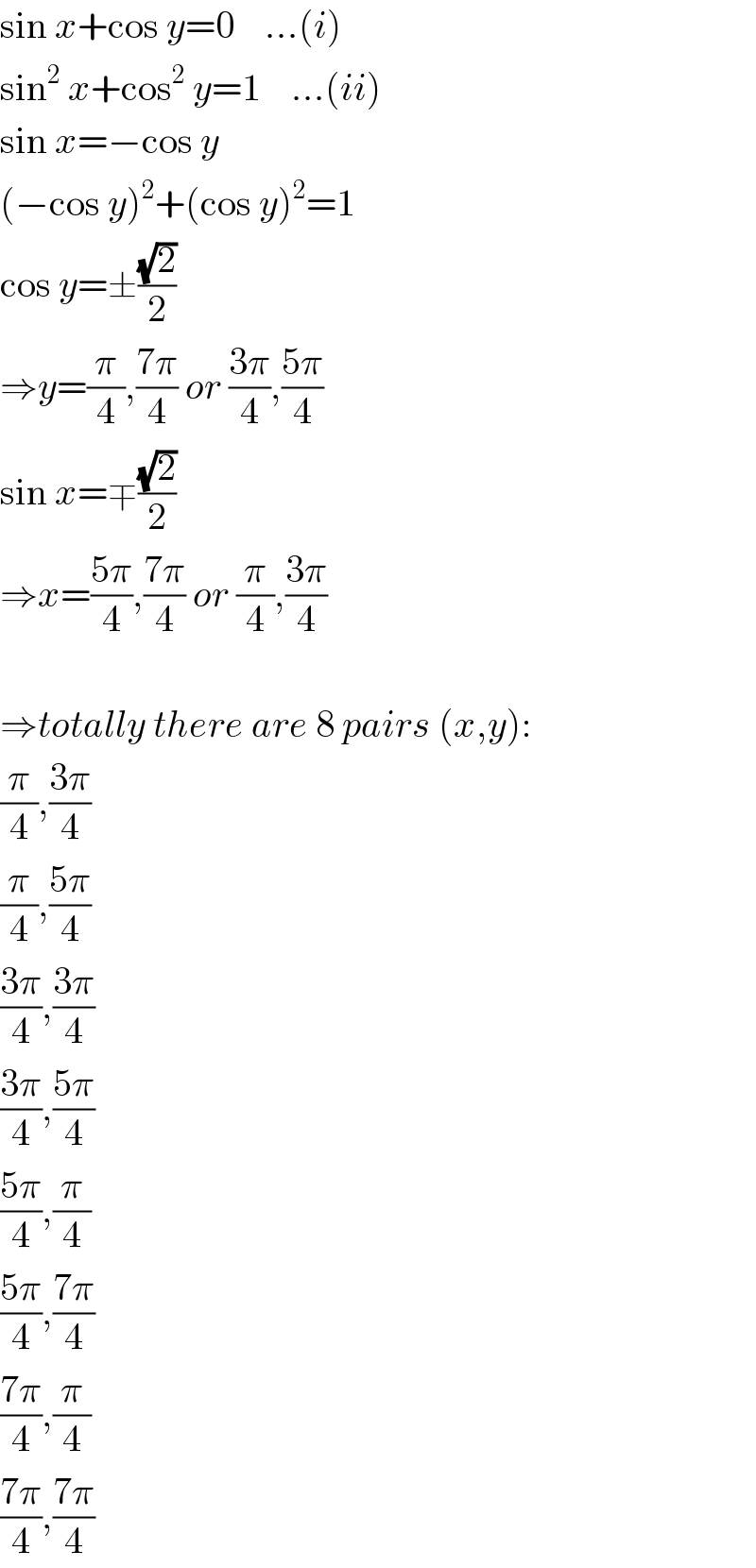
sin x+cos y=0 ...(i) sin^2 x+cos^2 y=1 ...(ii) sin x=−cos y (−cos y)^2 +(cos y)^2 =1 cos y=±((√2)/2) ⇒y=(π/4),((7π)/4) or ((3π)/4),((5π)/4) sin x=∓((√2)/2) ⇒x=((5π)/4),((7π)/4) or (π/4),((3π)/4) ⇒totally there are 8 pairs (x,y): (π/4),((3π)/4) (π/4),((5π)/4) ((3π)/4),((3π)/4) ((3π)/4),((5π)/4) ((5π)/4),(π/4) ((5π)/4),((7π)/4) ((7π)/4),(π/4) ((7π)/4),((7π)/4)

$$\mathrm{sin}\:{x}+\mathrm{cos}\:{y}=\mathrm{0}\:\:\:\:...\left({i}\right) \\ $$$$\mathrm{sin}^{\mathrm{2}} \:{x}+\mathrm{cos}^{\mathrm{2}} \:{y}=\mathrm{1}\:\:\:\:...\left({ii}\right) \\ $$$$\mathrm{sin}\:{x}=−\mathrm{cos}\:{y} \\ $$$$\left(−\mathrm{cos}\:{y}\right)^{\mathrm{2}} +\left(\mathrm{cos}\:{y}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$$\mathrm{cos}\:{y}=\pm\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\Rightarrow{y}=\frac{\pi}{\mathrm{4}},\frac{\mathrm{7}\pi}{\mathrm{4}}\:{or}\:\frac{\mathrm{3}\pi}{\mathrm{4}},\frac{\mathrm{5}\pi}{\mathrm{4}} \\ $$$$\mathrm{sin}\:{x}=\mp\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\Rightarrow{x}=\frac{\mathrm{5}\pi}{\mathrm{4}},\frac{\mathrm{7}\pi}{\mathrm{4}}\:{or}\:\frac{\pi}{\mathrm{4}},\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$ \\ $$$$\Rightarrow{totally}\:{there}\:{are}\:\mathrm{8}\:{pairs}\:\left({x},{y}\right): \\ $$$$\frac{\pi}{\mathrm{4}},\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$\frac{\pi}{\mathrm{4}},\frac{\mathrm{5}\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}},\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{3}\pi}{\mathrm{4}},\frac{\mathrm{5}\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{5}\pi}{\mathrm{4}},\frac{\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{5}\pi}{\mathrm{4}},\frac{\mathrm{7}\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{7}\pi}{\mathrm{4}},\frac{\pi}{\mathrm{4}} \\ $$$$\frac{\mathrm{7}\pi}{\mathrm{4}},\frac{\mathrm{7}\pi}{\mathrm{4}} \\ $$

Commented by Tinkutara last updated on 04/Jun/17

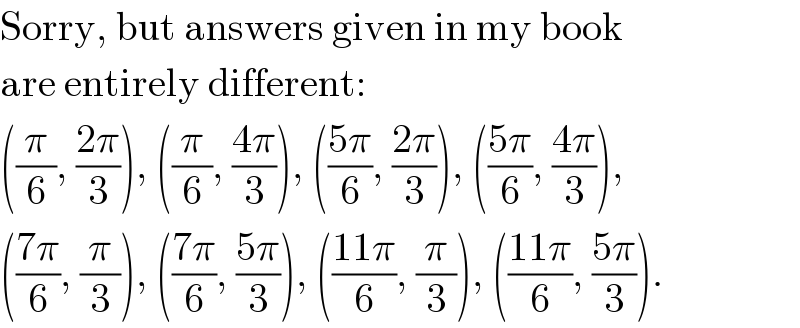
Sorry, but answers given in my book are entirely different: ((π/6), ((2π)/3)), ((π/6), ((4π)/3)), (((5π)/6), ((2π)/3)), (((5π)/6), ((4π)/3)), (((7π)/6), (π/3)), (((7π)/6), ((5π)/3)), (((11π)/6), (π/3)), (((11π)/6), ((5π)/3)).

$$\mathrm{Sorry},\:\mathrm{but}\:\mathrm{answers}\:\mathrm{given}\:\mathrm{in}\:\mathrm{my}\:\mathrm{book} \\ $$$$\mathrm{are}\:\mathrm{entirely}\:\mathrm{different}: \\ $$$$\left(\frac{\pi}{\mathrm{6}},\:\frac{\mathrm{2}\pi}{\mathrm{3}}\right),\:\left(\frac{\pi}{\mathrm{6}},\:\frac{\mathrm{4}\pi}{\mathrm{3}}\right),\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}},\:\frac{\mathrm{2}\pi}{\mathrm{3}}\right),\:\left(\frac{\mathrm{5}\pi}{\mathrm{6}},\:\frac{\mathrm{4}\pi}{\mathrm{3}}\right), \\ $$$$\left(\frac{\mathrm{7}\pi}{\mathrm{6}},\:\frac{\pi}{\mathrm{3}}\right),\:\left(\frac{\mathrm{7}\pi}{\mathrm{6}},\:\frac{\mathrm{5}\pi}{\mathrm{3}}\right),\:\left(\frac{\mathrm{11}\pi}{\mathrm{6}},\:\frac{\pi}{\mathrm{3}}\right),\:\left(\frac{\mathrm{11}\pi}{\mathrm{6}},\:\frac{\mathrm{5}\pi}{\mathrm{3}}\right). \\ $$

Commented by ajfour last updated on 04/Jun/17

wrong answers, what book?

$${wrong}\:{answers},\:{what}\:{book}? \\ $$

Commented by Tinkutara last updated on 04/Jun/17

It′s my coaching institute′s book for JEE preparation in Class XI.

$$\mathrm{It}'\mathrm{s}\:\mathrm{my}\:\mathrm{coaching}\:\mathrm{institute}'\mathrm{s}\:\mathrm{book}\:\mathrm{for} \\ $$$$\mathrm{JEE}\:\mathrm{preparation}\:\mathrm{in}\:\mathrm{Class}\:\mathrm{XI}. \\ $$

Commented by mrW1 last updated on 04/Jun/17

That book is misleading.

$${That}\:{book}\:{is}\:{misleading}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com