
Question Number 213887 by efronzo1 last updated on 20/Nov/24
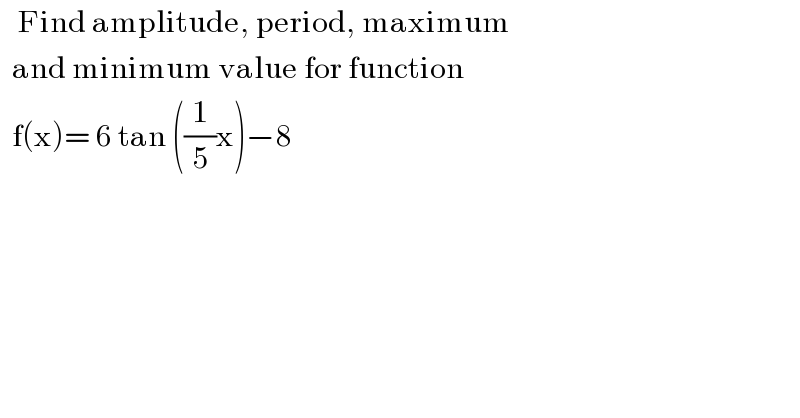
$$\:\:\:\mathrm{Find}\:\mathrm{amplitude},\:\mathrm{period},\:\mathrm{maximum}\: \\ $$$$\:\:\mathrm{and}\:\mathrm{minimum}\:\mathrm{value}\:\mathrm{for}\:\mathrm{function} \\ $$$$\:\:\mathrm{f}\left(\mathrm{x}\right)=\:\mathrm{6}\:\mathrm{tan}\:\left(\frac{\mathrm{1}}{\mathrm{5}}\mathrm{x}\right)−\mathrm{8}\: \\ $$
Answered by alephnull last updated on 08/Jan/25

$${amplitude}={none} \\ $$$${period}=\frac{\pi}{{b}} \\ $$$${b}\:=\:{coefficent}\:{of}\:{x}\: \\ $$$${inside}\:{tangent}\:{function} \\ $$$$ \\ $$$${b}=\frac{\pi}{\mathrm{1}/\mathrm{5}}=\mathrm{5}\pi \\ $$$${period}=\mathrm{5}\pi \\ $$$$ \\ $$$${min}\:{and}\:{max}\:{are}\:{unbounded}\:{so}\:{none} \\ $$
