
Question Number 168885 by Rasheed.Sindhi last updated on 20/Apr/22
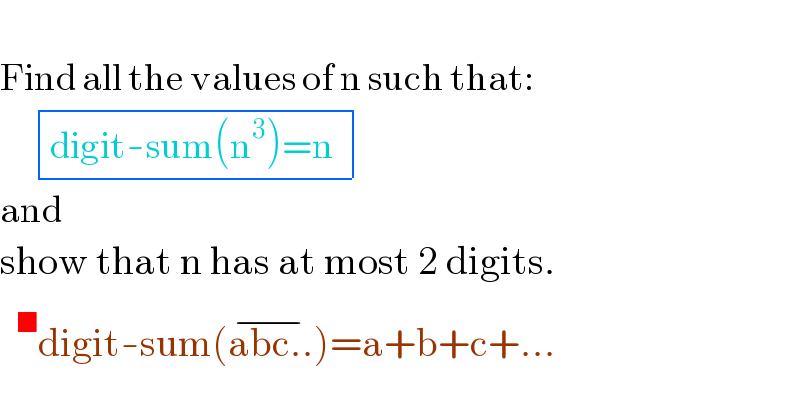
$$ \\ $$$$\mathrm{Find}\:\mathrm{all}\:\mathrm{the}\:\mathrm{values}\:\mathrm{of}\:\mathrm{n}\:\mathrm{such}\:\mathrm{that}: \\ $$$$\:\:\:\:\begin{array}{|c|}{\mathrm{digit}-\mathrm{sum}\left(\mathrm{n}^{\mathrm{3}} \right)=\mathrm{n}\:}\\\hline\end{array} \\ $$$$\mathrm{and} \\ $$$$\mathrm{show}\:\mathrm{that}\:\mathrm{n}\:\mathrm{has}\:\mathrm{at}\:\mathrm{most}\:\mathrm{2}\:\mathrm{digits}. \\ $$$$\:\:^{\blacksquare} \mathrm{digit}-\mathrm{sum}\left(\overline {\mathrm{abc}..}\right)=\mathrm{a}+\mathrm{b}+\mathrm{c}+... \\ $$
Commented by botir last updated on 20/Apr/22

$${very}\:\:\:{nice} \\ $$
Commented by Rasheed.Sindhi last updated on 20/Apr/22

$$\mathcal{T}{han}\mathcal{X}\:{sir}! \\ $$
Commented by MJS_new last updated on 20/Apr/22
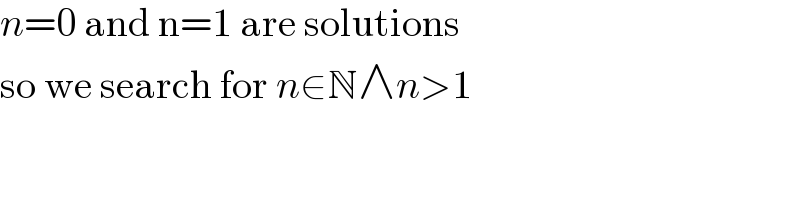
$${n}=\mathrm{0}\:\mathrm{and}\:\mathrm{n}=\mathrm{1}\:\mathrm{are}\:\mathrm{solutions} \\ $$$$\mathrm{so}\:\mathrm{we}\:\mathrm{search}\:\mathrm{for}\:{n}\in\mathbb{N}\wedge{n}>\mathrm{1} \\ $$
Commented by MJS_new last updated on 20/Apr/22
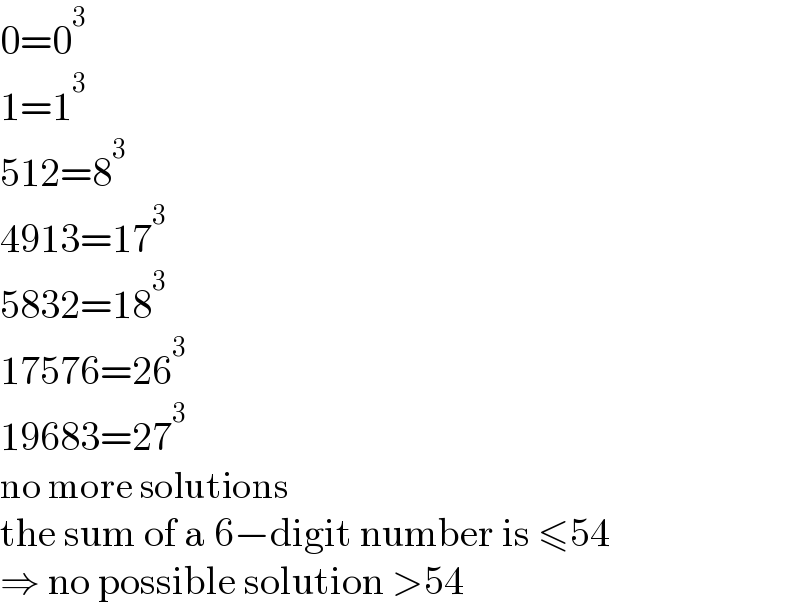
$$\mathrm{0}=\mathrm{0}^{\mathrm{3}} \\ $$$$\mathrm{1}=\mathrm{1}^{\mathrm{3}} \\ $$$$\mathrm{512}=\mathrm{8}^{\mathrm{3}} \\ $$$$\mathrm{4913}=\mathrm{17}^{\mathrm{3}} \\ $$$$\mathrm{5832}=\mathrm{18}^{\mathrm{3}} \\ $$$$\mathrm{17576}=\mathrm{26}^{\mathrm{3}} \\ $$$$\mathrm{19683}=\mathrm{27}^{\mathrm{3}} \\ $$$$\mathrm{no}\:\mathrm{more}\:\mathrm{solutions} \\ $$$$\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{a}\:\mathrm{6}−\mathrm{digit}\:\mathrm{number}\:\mathrm{is}\:\leqslant\mathrm{54} \\ $$$$\Rightarrow\:\mathrm{no}\:\mathrm{possible}\:\mathrm{solution}\:>\mathrm{54} \\ $$
Commented by MJS_new last updated on 21/Apr/22
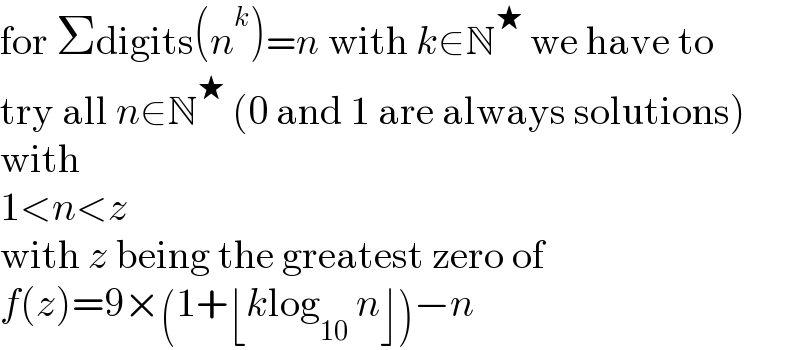
$$\mathrm{for}\:\Sigma\mathrm{digits}\left({n}^{{k}} \right)={n}\:\mathrm{with}\:{k}\in\mathbb{N}^{\bigstar} \:\mathrm{we}\:\mathrm{have}\:\mathrm{to} \\ $$$$\mathrm{try}\:\mathrm{all}\:{n}\in\mathbb{N}^{\bigstar} \:\left(\mathrm{0}\:\mathrm{and}\:\mathrm{1}\:\mathrm{are}\:\mathrm{always}\:\mathrm{solutions}\right) \\ $$$$\mathrm{with} \\ $$$$\mathrm{1}<{n}<{z} \\ $$$$\mathrm{with}\:{z}\:\mathrm{being}\:\mathrm{the}\:\mathrm{greatest}\:\mathrm{zero}\:\mathrm{of} \\ $$$${f}\left({z}\right)=\mathrm{9}×\left(\mathrm{1}+\lfloor{k}\mathrm{log}_{\mathrm{10}} \:{n}\rfloor\right)−{n} \\ $$
Commented by Rasheed.Sindhi last updated on 20/Apr/22

$$\mathrm{Great}! \\ $$$$\mathbb{T}\boldsymbol{\mathrm{han}}\Bbbk\boldsymbol{\mathrm{s}}\:\boldsymbol{\mathrm{sir}}! \\ $$
Commented by Rasheed.Sindhi last updated on 21/Apr/22
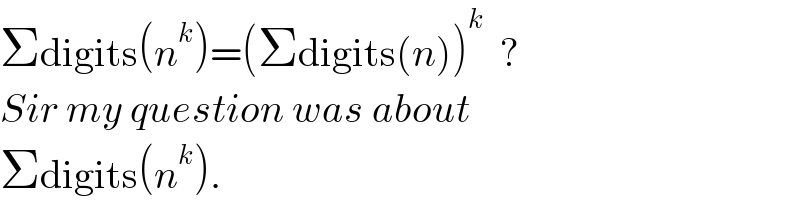
$$\Sigma\mathrm{digits}\left({n}^{{k}} \right)=\left(\Sigma\mathrm{digits}\left({n}\right)\right)^{{k}} \:\:? \\ $$$${Sir}\:{my}\:{question}\:{was}\:{about} \\ $$$$\Sigma\mathrm{digits}\left({n}^{{k}} \right). \\ $$
Commented by MJS_new last updated on 21/Apr/22

$$\mathrm{sorry}\:\mathrm{it}'\mathrm{s}\:\mathrm{a}\:\mathrm{typo}.\:\mathrm{I}\:\mathrm{corrected}\:\mathrm{it}. \\ $$
Commented by Rasheed.Sindhi last updated on 21/Apr/22
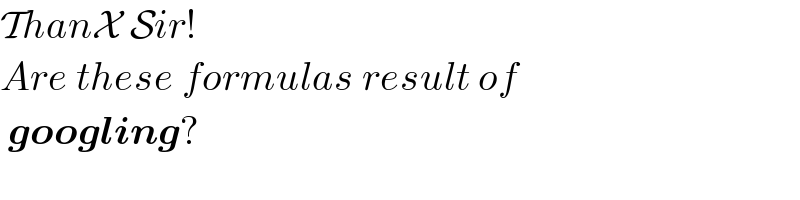
$$\mathcal{T}{han}\mathcal{X}\:\mathcal{S}{ir}! \\ $$$${Are}\:{these}\:{formulas}\:{result}\:{of} \\ $$$$\:\boldsymbol{{googling}}? \\ $$
Commented by MJS_new last updated on 21/Apr/22
no. brainwork. I used a simple calculator and started with n=1, 2, 3, ... then I found that there must be a border because the max sum of k digits is 9k...
Commented by Rasheed.Sindhi last updated on 21/Apr/22
No doubt your calculator is simple but your brain is as complex as mathematics is. No doubt your formulae are creative work sir!
