
Question Number 217040 by ArshadS last updated on 27/Feb/25
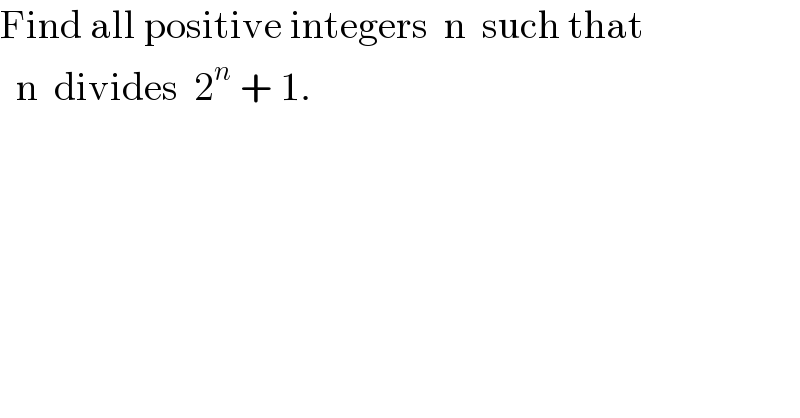
$$\mathrm{Find}\:\mathrm{all}\:\mathrm{positive}\:\mathrm{integers}\:\:\mathrm{n}\:\:\mathrm{such}\:\mathrm{that} \\ $$$$\:\:\mathrm{n}\:\:\mathrm{divides}\:\:\mathrm{2}^{{n}} \:+\:\mathrm{1}.\:\: \\ $$
Answered by Ghisom last updated on 27/Feb/25
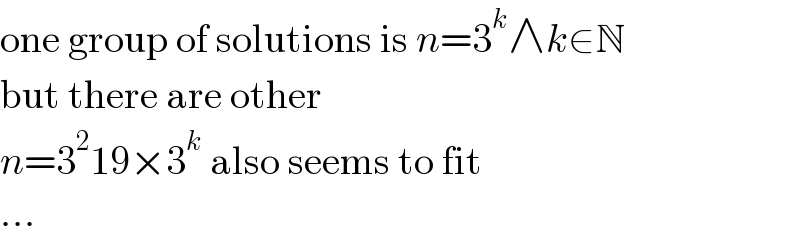
$$\mathrm{one}\:\mathrm{group}\:\mathrm{of}\:\mathrm{solutions}\:\mathrm{is}\:{n}=\mathrm{3}^{{k}} \wedge{k}\in\mathbb{N} \\ $$$$\mathrm{but}\:\mathrm{there}\:\mathrm{are}\:\mathrm{other} \\ $$$${n}=\mathrm{3}^{\mathrm{2}} \mathrm{19}×\mathrm{3}^{{k}} \:\mathrm{also}\:\mathrm{seems}\:\mathrm{to}\:\mathrm{fit} \\ $$$$... \\ $$
Answered by mehdee7396 last updated on 28/Feb/25

$${n}=\mathrm{3}^{{k}} \\ $$$$ \\ $$
