
Question Number 117365 by Dwaipayan Shikari last updated on 11/Oct/20
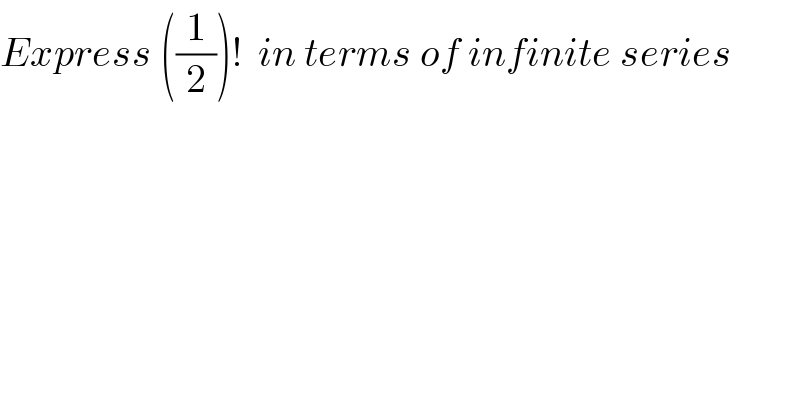
$${Express}\:\left(\frac{\mathrm{1}}{\mathrm{2}}\right)!\:\:{in}\:{terms}\:{of}\:{infinite}\:{series} \\ $$
Commented by Dwaipayan Shikari last updated on 11/Oct/20
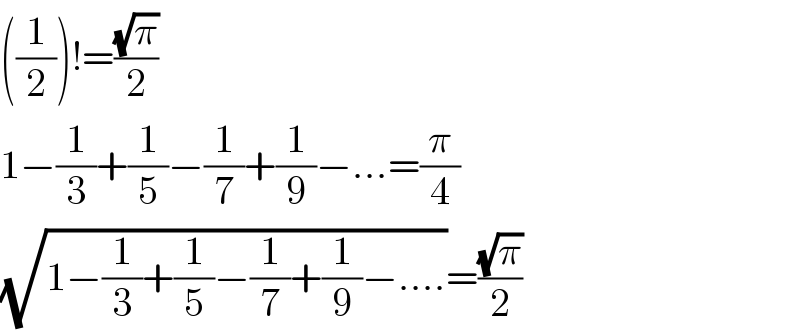
$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)!=\frac{\sqrt{\pi}}{\mathrm{2}} \\ $$$$\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{7}}+\frac{\mathrm{1}}{\mathrm{9}}−...=\frac{\pi}{\mathrm{4}} \\ $$$$\sqrt{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{5}}−\frac{\mathrm{1}}{\mathrm{7}}+\frac{\mathrm{1}}{\mathrm{9}}−....}=\frac{\sqrt{\pi}}{\mathrm{2}} \\ $$
Answered by mindispower last updated on 11/Oct/20

$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)=\sqrt{\pi}=\sqrt{\pi}.\underset{{k}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\mathrm{2}^{{k}} } \\ $$
