Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 9658 by tawakalitu last updated on 23/Dec/16

Experiment shows that the viscous force, F on a spherical body of radius, r moving with angular velocity, n is, F = Kr^a n^b ω^c . Where K is a dimentionless constant. using the method of dimentional analysis, determine the values of a, b and c

$$\mathrm{Experiment}\:\mathrm{shows}\:\mathrm{that}\:\mathrm{the}\:\mathrm{viscous}\:\mathrm{force},\:\mathrm{F} \\ $$$$\mathrm{on}\:\mathrm{a}\:\mathrm{spherical}\:\mathrm{body}\:\mathrm{of}\:\mathrm{radius},\:\mathrm{r}\:\mathrm{moving}\:\mathrm{with} \\ $$$$\mathrm{angular}\:\mathrm{velocity},\:\mathrm{n}\:\mathrm{is},\:\mathrm{F}\:=\:\mathrm{Kr}^{\mathrm{a}} \mathrm{n}^{\mathrm{b}} \omega^{\mathrm{c}} . \\ $$$$\mathrm{Where}\:\mathrm{K}\:\mathrm{is}\:\mathrm{a}\:\mathrm{dimentionless}\:\mathrm{constant}. \\ $$$$\mathrm{using}\:\mathrm{the}\:\mathrm{method}\:\mathrm{of}\:\mathrm{dimentional}\:\mathrm{analysis}, \\ $$$$\mathrm{determine}\:\mathrm{the}\:\mathrm{values}\:\mathrm{of}\:\mathrm{a},\:\mathrm{b}\:\mathrm{and}\:\mathrm{c} \\ $$

Commented by ridwan balatif last updated on 23/Dec/16

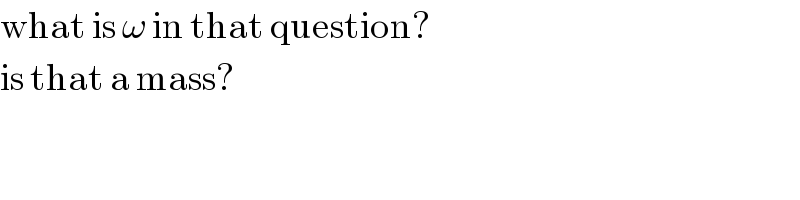
what is ω in that question? is that a mass?

$$\mathrm{what}\:\mathrm{is}\:\omega\:\mathrm{in}\:\mathrm{that}\:\mathrm{question}? \\ $$$$\mathrm{is}\:\mathrm{that}\:\mathrm{a}\:\mathrm{mass}? \\ $$

Commented by ridwan balatif last updated on 23/Dec/16

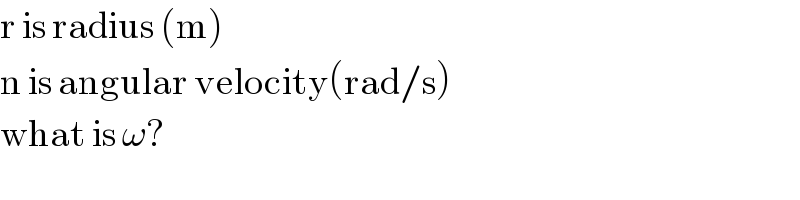
r is radius (m) n is angular velocity(rad/s) what is ω?

$$\mathrm{r}\:\mathrm{is}\:\mathrm{radius}\:\left(\mathrm{m}\right) \\ $$$$\mathrm{n}\:\mathrm{is}\:\mathrm{angular}\:\mathrm{velocity}\left(\mathrm{rad}/\mathrm{s}\right) \\ $$$$\mathrm{what}\:\mathrm{is}\:\omega? \\ $$

Commented by sandy_suhendra last updated on 23/Dec/16

I think that is F=Kr^a η^b v^c η=coefficient of viscosity (Pa s) r=radius of the ball (m) v=velocity of the ball (m/s) and [F]=[M][L][T]^(−2) [r]=[L] [η]=[M][L]^(−1) [T]^(−1) [v]=[L][T]^(−1) F=Kr^a η^b v^c [M][L][T]^(−2) =[L]^a {[M]^b [L]^(−b) [T]^(−b) }{[L]^c [T]^(−c) } [M][L][T]^(−2) =[M]^b [L]^(a−b+c) [T]^(−b−c) so b=1 −b−c=−2 ⇒ c=1 a−b+c=1 ⇒ a=1

$$\mathrm{I}\:\mathrm{think}\:\mathrm{that}\:\mathrm{is}\:\mathrm{F}=\mathrm{Kr}^{\mathrm{a}} \eta^{\mathrm{b}} \mathrm{v}^{\mathrm{c}} \\ $$$$\eta=\mathrm{coefficient}\:\mathrm{of}\:\mathrm{viscosity}\:\left(\mathrm{Pa}\:\mathrm{s}\right) \\ $$$$\mathrm{r}=\mathrm{radius}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ball}\:\left(\mathrm{m}\right) \\ $$$$\mathrm{v}=\mathrm{velocity}\:\mathrm{of}\:\mathrm{the}\:\mathrm{ball}\:\left(\mathrm{m}/\mathrm{s}\right) \\ $$$$\mathrm{and}\:\left[\mathrm{F}\right]=\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\left[\mathrm{r}\right]=\left[\mathrm{L}\right] \\ $$$$\:\:\:\:\:\:\:\:\:\left[\eta\right]=\left[\mathrm{M}\right]\left[\mathrm{L}\right]^{−\mathrm{1}} \left[\mathrm{T}\right]^{−\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\:\left[\mathrm{v}\right]=\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{1}} \\ $$$$ \\ $$$$\mathrm{F}=\mathrm{Kr}^{\mathrm{a}} \eta^{\mathrm{b}} \mathrm{v}^{\mathrm{c}} \\ $$$$\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} =\left[\mathrm{L}\right]^{\mathrm{a}} \left\{\left[\mathrm{M}\right]^{\mathrm{b}} \left[\mathrm{L}\right]^{−\mathrm{b}} \left[\mathrm{T}\right]^{−\mathrm{b}} \right\}\left\{\left[\mathrm{L}\right]^{\mathrm{c}} \left[\mathrm{T}\right]^{−\mathrm{c}} \right\} \\ $$$$\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} =\left[\mathrm{M}\right]^{\mathrm{b}} \left[\mathrm{L}\right]^{\mathrm{a}−\mathrm{b}+\mathrm{c}} \left[\mathrm{T}\right]^{−\mathrm{b}−\mathrm{c}} \\ $$$$\mathrm{so}\:\:\:\:\mathrm{b}=\mathrm{1} \\ $$$$\:\:\:\:\:\:\:−\mathrm{b}−\mathrm{c}=−\mathrm{2}\:\Rightarrow\:\mathrm{c}=\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{a}−\mathrm{b}+\mathrm{c}=\mathrm{1}\:\Rightarrow\:\mathrm{a}=\mathrm{1} \\ $$

Commented by ridwan balatif last updated on 23/Dec/16

now i understand

$$\mathrm{now}\:\mathrm{i}\:\mathrm{understand} \\ $$

Commented by tawakalitu last updated on 23/Dec/16

i really appreciate. God bless you.

$$\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$

Answered by ridwan balatif last updated on 23/Dec/16

dimentional F=kgms^(−2) =[M][L][T]^(−2) r =m =[L] η =Pa.s=kgm^(−1) s^(−1) =[M][L]^(−1) [T]^(−1) ω =rad/s =[T]^(−1) F=k.r^a .η.^b ω^c [M][L][T]^(−2) =([L])^a ([M][L]^(−1) [T]^(−1) )^b ([T]^(−1) )^c [M][L][T]^(−2) =[M]^b [L]^(a−b) [T]^(−b−c) from dimentional [M] we get b=1 from dimentional [L] we get a−b=1 a−1=1 a=2 from dimentional [T] we get −b−c=−2 −1−c=−2 c=1 so, a=2 b=1 c=1 F=kr^2 ηω

$$\mathrm{dimentional} \\ $$$$\mathrm{F}=\mathrm{kgms}^{−\mathrm{2}} \\ $$$$\:\:\:\:=\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} \\ $$$$\mathrm{r}\:\:=\mathrm{m} \\ $$$$\:\:\:\:\:=\left[\mathrm{L}\right] \\ $$$$\eta\:\:=\mathrm{Pa}.\mathrm{s}=\mathrm{kgm}^{−\mathrm{1}} \mathrm{s}^{−\mathrm{1}} \\ $$$$\:\:\:\:\:=\left[\mathrm{M}\right]\left[\mathrm{L}\right]^{−\mathrm{1}} \left[\mathrm{T}\right]^{−\mathrm{1}} \\ $$$$\omega\:=\mathrm{rad}/\mathrm{s} \\ $$$$\:\:\:\:\:=\left[\mathrm{T}\right]^{−\mathrm{1}} \\ $$$$\mathrm{F}=\mathrm{k}.\mathrm{r}^{\mathrm{a}} .\eta.^{\mathrm{b}} \omega^{\mathrm{c}} \\ $$$$\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} =\left(\left[\mathrm{L}\right]\right)^{\mathrm{a}} \left(\left[\mathrm{M}\right]\left[\mathrm{L}\right]^{−\mathrm{1}} \left[\mathrm{T}\right]^{−\mathrm{1}} \right)^{\mathrm{b}} \left(\left[\mathrm{T}\right]^{−\mathrm{1}} \right)^{\mathrm{c}} \\ $$$$\left[\mathrm{M}\right]\left[\mathrm{L}\right]\left[\mathrm{T}\right]^{−\mathrm{2}} =\left[\mathrm{M}\right]^{\mathrm{b}} \left[\mathrm{L}\right]^{\mathrm{a}−\mathrm{b}} \left[\mathrm{T}\right]^{−\mathrm{b}−\mathrm{c}} \\ $$$$\mathrm{from}\:\mathrm{dimentional}\:\left[\mathrm{M}\right]\:\mathrm{we}\:\mathrm{get}\:\mathrm{b}=\mathrm{1} \\ $$$$\mathrm{from}\:\mathrm{dimentional}\:\left[\mathrm{L}\right]\:\mathrm{we}\:\mathrm{get}\:\mathrm{a}−\mathrm{b}=\mathrm{1} \\ $$$$\mathrm{a}−\mathrm{1}=\mathrm{1} \\ $$$$\mathrm{a}=\mathrm{2} \\ $$$$\mathrm{from}\:\mathrm{dimentional}\:\left[\mathrm{T}\right]\:\mathrm{we}\:\mathrm{get}\:−\mathrm{b}−\mathrm{c}=−\mathrm{2} \\ $$$$−\mathrm{1}−\mathrm{c}=−\mathrm{2} \\ $$$$\mathrm{c}=\mathrm{1} \\ $$$$\mathrm{so},\:\mathrm{a}=\mathrm{2}\:\mathrm{b}=\mathrm{1}\:\mathrm{c}=\mathrm{1} \\ $$$$\mathrm{F}=\mathrm{kr}^{\mathrm{2}} \eta\omega \\ $$

Commented by sandy_suhendra last updated on 23/Dec/16

I think that is not ω (rad/s) but v=linear velocity (m/s) because the formula must be F=6πrηv and K=6π [Stokes′ Law]

$$\mathrm{I}\:\mathrm{think}\:\mathrm{that}\:\mathrm{is}\:\mathrm{not}\:\omega\:\left(\mathrm{rad}/\mathrm{s}\right)\:\mathrm{but}\:\mathrm{v}=\mathrm{linear}\:\mathrm{velocity}\:\left(\mathrm{m}/\mathrm{s}\right) \\ $$$$\mathrm{because}\:\mathrm{the}\:\mathrm{formula}\:\mathrm{must}\:\mathrm{be}\:\mathrm{F}=\mathrm{6}\pi\mathrm{r}\eta\mathrm{v} \\ $$$$\mathrm{and}\:\mathrm{K}=\mathrm{6}\pi\:\:\:\left[\mathrm{Stokes}'\:\mathrm{Law}\right] \\ $$

Commented by ridwan balatif last updated on 23/Dec/16

yeah, i remember now thank you sir

$$\mathrm{yeah},\:\mathrm{i}\:\mathrm{remember}\:\mathrm{now} \\ $$$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$

Commented by tawakalitu last updated on 23/Dec/16

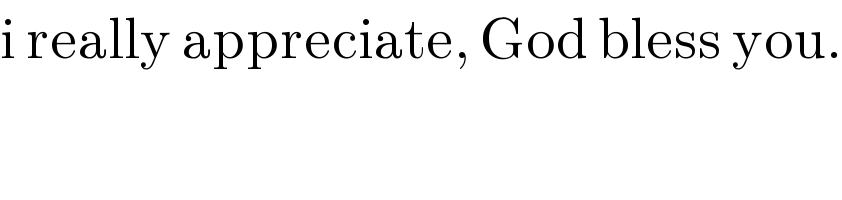
i really appreciate, God bless you.

$$\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate},\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com