
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 137943 by john_santu last updated on 08/Apr/21
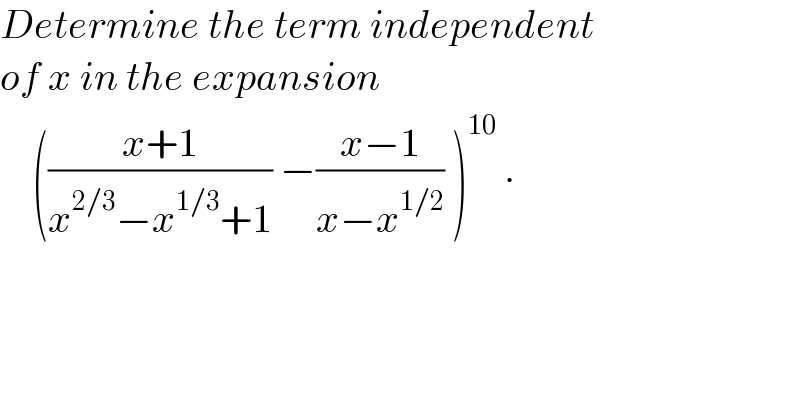
$${Determine}\:{the}\:{term}\:{independent} \\ $$$${of}\:{x}\:{in}\:{the}\:{expansion}\: \\ $$$$\:\:\:\:\left(\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}/\mathrm{3}} −{x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}}\:−\frac{{x}−\mathrm{1}}{{x}−{x}^{\mathrm{1}/\mathrm{2}} }\:\right)^{\mathrm{10}} \:. \\ $$
Answered by EDWIN88 last updated on 08/Apr/21
![[ ((x+1)/(x^(2/3) −x^(1/3) +1)) − ((x−1)/(x−x^(1/2) )) ]^(10) = [ (((x^(1/3) +1)(x^(2/3) −x^(1/3) +1))/(x^(2/3) −x^(1/3) +1)) −(((x^(1/2) −1)(x^(1/2) +1))/(x^(1/2) (x^(1/2) −1))) ]^(10) = [ (x^(1/3) +1)−((x^(1/2) +1)/x^(1/2) ) ]^(10) = [ x^(1/3) −x^(−1/2) ]^(10) = Σ_(k=0) ^(10) C(10,k)x^(k/3) (−x^(−1/2) )^(10−k) the term independent of x it must be from x^((k/3)+(k/2)−5) = x^0 , ((5k)/6) = 5 ⇒k=6 then T_6 = (((10)),(( 6)) ) (x^(1/3) )^6 (−x^(−1/2) )^4 T_6 = ((10×9×8×7)/(4×3×2×1)) (−1)^4 = 210](Q137945.png)
$$\:\left[\:\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}/\mathrm{3}} −{x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}}\:−\:\frac{{x}−\mathrm{1}}{{x}−{x}^{\mathrm{1}/\mathrm{2}} }\:\right]^{\mathrm{10}} = \\ $$$$\:\left[\:\frac{\left({x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}\right)\left({x}^{\mathrm{2}/\mathrm{3}} −{x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}\right)}{{x}^{\mathrm{2}/\mathrm{3}} −{x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}}\:−\frac{\left({x}^{\mathrm{1}/\mathrm{2}} −\mathrm{1}\right)\left({x}^{\mathrm{1}/\mathrm{2}} +\mathrm{1}\right)}{{x}^{\mathrm{1}/\mathrm{2}} \left({x}^{\mathrm{1}/\mathrm{2}} −\mathrm{1}\right)}\:\right]^{\mathrm{10}} = \\ $$$$\left[\:\left({x}^{\mathrm{1}/\mathrm{3}} +\mathrm{1}\right)−\frac{{x}^{\mathrm{1}/\mathrm{2}} +\mathrm{1}}{{x}^{\mathrm{1}/\mathrm{2}} }\:\right]^{\mathrm{10}} = \\ $$$$\left[\:{x}^{\mathrm{1}/\mathrm{3}} −{x}^{−\mathrm{1}/\mathrm{2}} \:\right]^{\mathrm{10}} \:=\:\underset{{k}=\mathrm{0}} {\overset{\mathrm{10}} {\sum}}{C}\left(\mathrm{10},{k}\right){x}^{{k}/\mathrm{3}} \left(−{x}^{−\mathrm{1}/\mathrm{2}} \right)^{\mathrm{10}−{k}} \\ $$$${the}\:{term}\:{independent}\:{of}\:{x}\:{it}\:{must}\:{be} \\ $$$${from}\:{x}^{\frac{{k}}{\mathrm{3}}+\frac{{k}}{\mathrm{2}}−\mathrm{5}} \:=\:{x}^{\mathrm{0}} \:,\:\frac{\mathrm{5}{k}}{\mathrm{6}}\:=\:\mathrm{5}\:\Rightarrow{k}=\mathrm{6} \\ $$$${then}\:{T}_{\mathrm{6}} \:=\:\begin{pmatrix}{\mathrm{10}}\\{\:\:\mathrm{6}}\end{pmatrix}\:\left({x}^{\mathrm{1}/\mathrm{3}} \right)^{\mathrm{6}} \left(−{x}^{−\mathrm{1}/\mathrm{2}} \right)^{\mathrm{4}} \\ $$$${T}_{\mathrm{6}} \:=\:\frac{\mathrm{10}×\mathrm{9}×\mathrm{8}×\mathrm{7}}{\mathrm{4}×\mathrm{3}×\mathrm{2}×\mathrm{1}}\:\left(−\mathrm{1}\right)^{\mathrm{4}} \:=\:\mathrm{210} \\ $$
