
Question Number 30267 by Nayon.Sm last updated on 19/Feb/18
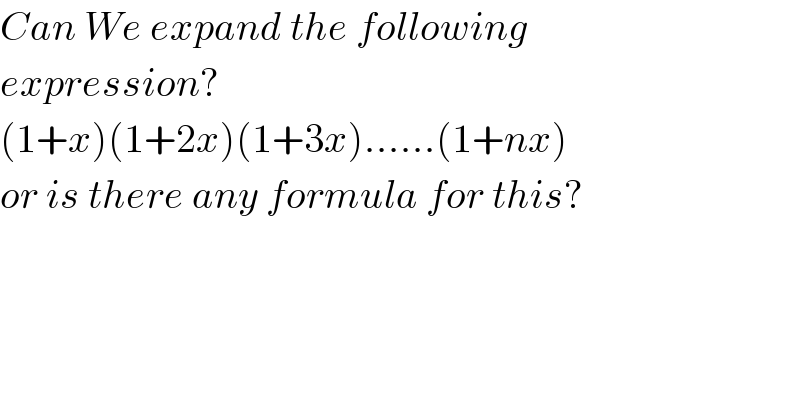
$${Can}\:{We}\:{expand}\:{the}\:{following} \\ $$$${expression}? \\ $$$$\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\mathrm{2}{x}\right)\left(\mathrm{1}+\mathrm{3}{x}\right)......\left(\mathrm{1}+{nx}\right) \\ $$$${or}\:{is}\:{there}\:{any}\:{formula}\:{for}\:{this}? \\ $$
Commented by Penguin last updated on 19/Feb/18
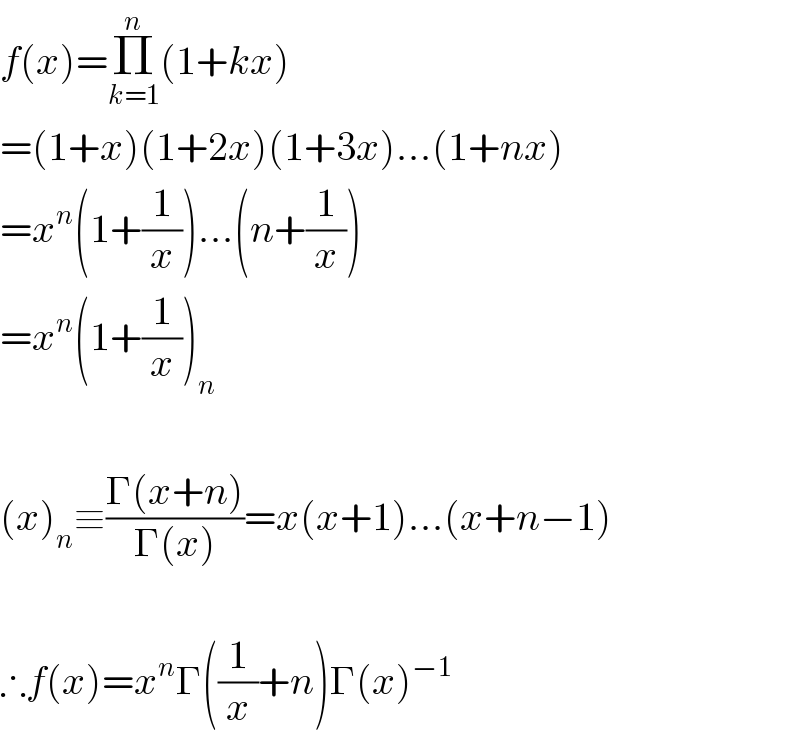
$${f}\left({x}\right)=\underset{{k}=\mathrm{1}} {\overset{{n}} {\prod}}\left(\mathrm{1}+{kx}\right) \\ $$$$=\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\mathrm{2}{x}\right)\left(\mathrm{1}+\mathrm{3}{x}\right)...\left(\mathrm{1}+{nx}\right) \\ $$$$={x}^{{n}} \left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)...\left({n}+\frac{\mathrm{1}}{{x}}\right) \\ $$$$={x}^{{n}} \left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)_{{n}} \\ $$$$\: \\ $$$$\left({x}\right)_{{n}} \equiv\frac{\Gamma\left({x}+{n}\right)}{\Gamma\left({x}\right)}={x}\left({x}+\mathrm{1}\right)...\left({x}+{n}−\mathrm{1}\right) \\ $$$$\: \\ $$$$\therefore{f}\left({x}\right)={x}^{{n}} \Gamma\left(\frac{\mathrm{1}}{{x}}+{n}\right)\Gamma\left({x}\right)^{−\mathrm{1}} \\ $$
Commented by Nayon.Sm last updated on 19/Feb/18

$${its}\:{not} \\ $$
Commented by Penguin last updated on 19/Feb/18

$$\mathrm{Why}? \\ $$
Commented by Nayon.Sm last updated on 19/Feb/18

$${what}\:{is}\Gamma\left({x}\right)\overset{} {?} \\ $$$${what}\:{is}\: \\ $$
Commented by Penguin last updated on 19/Feb/18

$$\mathrm{Gamma}\:\mathrm{function} \\ $$$$\Gamma\left({x}\right)=\left({x}−\mathrm{1}\right)!=\left({x}−\mathrm{1}\right)×\left({x}−\mathrm{2}\right)×...×\mathrm{3}×\mathrm{2}×\mathrm{1} \\ $$
Commented by abdo imad last updated on 20/Feb/18
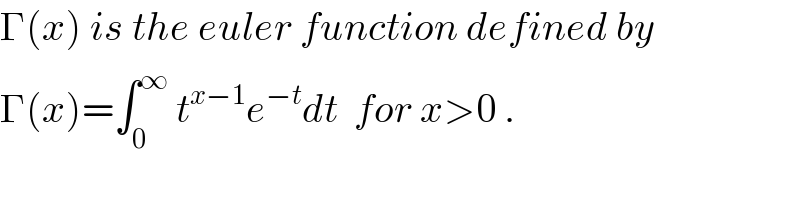
$$\Gamma\left({x}\right)\:{is}\:{the}\:{euler}\:{function}\:{defined}\:{by} \\ $$$$\Gamma\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:{t}^{{x}−\mathrm{1}} {e}^{−{t}} {dt}\:\:{for}\:{x}>\mathrm{0}\:. \\ $$
Commented by Nayon.Sm last updated on 10/Mar/18
Yes
