
Question Number 180902 by nadovic last updated on 19/Nov/22
![Calculate the root mean square speed of the molecules of a Helium gas kept in a gas cylinder at 400K. [Take R = 8.3 Jmol^(−1) K^(−1) ] The answer provided is 1.58 kms^(−1) Please I need help with the solution](Q180902.png)
$$\mathrm{Calculate}\:\mathrm{the}\:\mathrm{root}\:\mathrm{mean}\:\mathrm{square}\: \\ $$$$\mathrm{speed}\:\mathrm{of}\:\mathrm{the}\:\mathrm{molecules}\:\mathrm{of}\:\mathrm{a}\:{Helium} \\ $$$$\mathrm{gas}\:\mathrm{kept}\:\mathrm{in}\:\mathrm{a}\:\mathrm{gas}\:\mathrm{cylinder}\:\mathrm{at}\:\mathrm{400K}. \\ $$$$\:\:\:\:\:\:\left[{Take}\:\mathrm{R}\:=\:\mathrm{8}.\mathrm{3}\:{Jmol}^{−\mathrm{1}} {K}^{−\mathrm{1}} \right] \\ $$$${The}\:{answer}\:{provided}\:{is}\:\mathrm{1}.\mathrm{58}\:{kms}^{−\mathrm{1}} \\ $$$$\mathrm{Please}\:\mathrm{I}\:\mathrm{need}\:\mathrm{help}\:\mathrm{with}\:\mathrm{the}\:\mathrm{solution} \\ $$
Answered by Sam09 last updated on 19/Nov/22
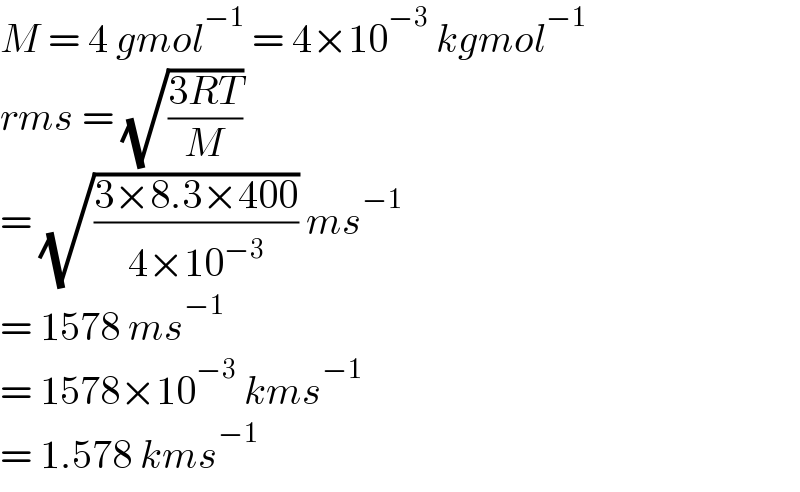
$${M}\:=\:\mathrm{4}\:{gmol}^{−\mathrm{1}} \:=\:\mathrm{4}×\mathrm{10}^{−\mathrm{3}} \:{kgmol}^{−\mathrm{1}} \\ $$$${rms}\:=\:\sqrt{\frac{\mathrm{3}{RT}}{{M}}} \\ $$$$=\:\sqrt{\frac{\mathrm{3}×\mathrm{8}.\mathrm{3}×\mathrm{400}}{\mathrm{4}×\mathrm{10}^{−\mathrm{3}} }}\:{ms}^{−\mathrm{1}} \\ $$$$=\:\mathrm{1578}\:{ms}^{−\mathrm{1}} \\ $$$$=\:\mathrm{1578}×\mathrm{10}^{−\mathrm{3}} \:{kms}^{−\mathrm{1}} \\ $$$$=\:\mathrm{1}.\mathrm{578}\:{kms}^{−\mathrm{1}} \\ $$
Commented by nadovic last updated on 11/Dec/22

$$\mathrm{Thanks}\:\mathrm{Sir} \\ $$
