
Previous in Differential Equation Next in Differential Equation
Question Number 7628 by Tawakalitu. last updated on 06/Sep/16
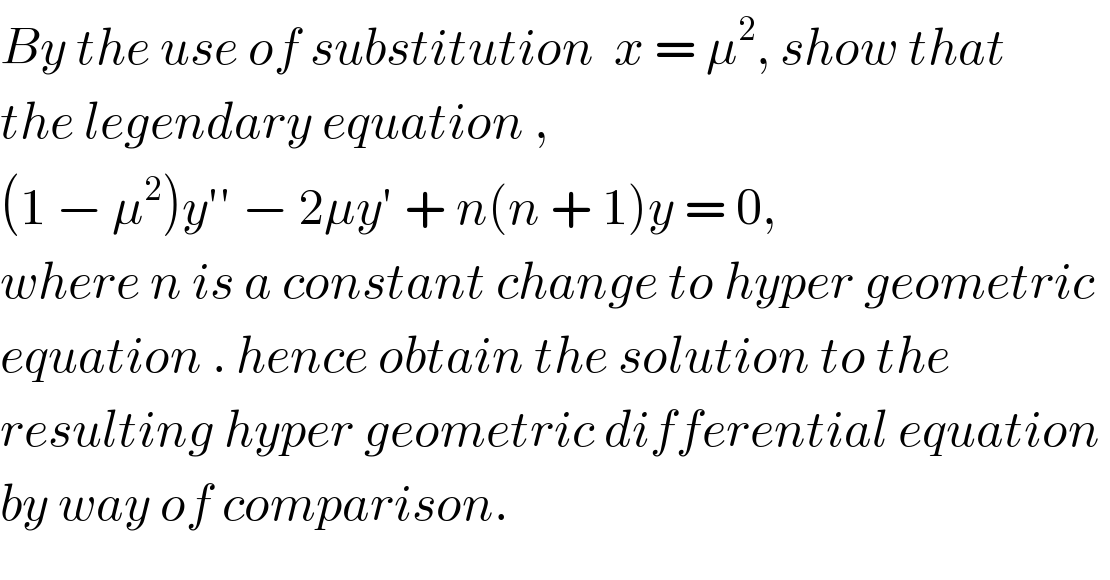
$${By}\:{the}\:{use}\:{of}\:{substitution}\:\:{x}\:=\:\mu^{\mathrm{2}} ,\:{show}\:{that} \\ $$$${the}\:{legendary}\:{equation}\:, \\ $$$$\left(\mathrm{1}\:−\:\mu^{\mathrm{2}} \right){y}''\:−\:\mathrm{2}\mu{y}'\:+\:{n}\left({n}\:+\:\mathrm{1}\right){y}\:=\:\mathrm{0},\: \\ $$$${where}\:{n}\:{is}\:{a}\:{constant}\:{change}\:{to}\:{hyper}\:{geometric} \\ $$$${equation}\:.\:{hence}\:{obtain}\:{the}\:{solution}\:{to}\:{the}\: \\ $$$${resulting}\:{hyper}\:{geometric}\:{differential}\:{equation}\: \\ $$$${by}\:{way}\:{of}\:{comparison}. \\ $$
