
Question Number 122649 by mathocean1 last updated on 18/Nov/20

$${By}\:{divising}\:{an}\:{integer}\:{a}\:{by}\: \\ $$$${integer}\:{b}\:{we}\:{find}\:{the}\:{result}: \\ $$$$\mathrm{0}.\mathrm{285714285714}...\:{followed} \\ $$$${by}\:{a}\:{group}\:{of}\:\mathrm{6}\:{digits}:\:\mathrm{285714} \\ $$$${which}\:{is}\:{repeated}\:{indefinited}. \\ $$$${determinate}\:{the}\:{fraction}\:\frac{{a}}{{b}}\: \\ $$$$ \\ $$$$ \\ $$
Commented by mr W last updated on 18/Nov/20
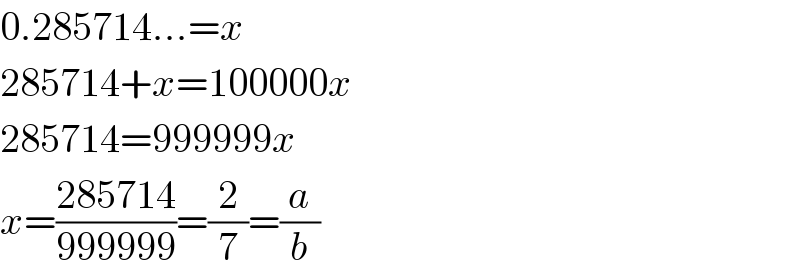
$$\mathrm{0}.\mathrm{285714}...={x} \\ $$$$\mathrm{285714}+{x}=\mathrm{100000}{x} \\ $$$$\mathrm{285714}=\mathrm{999999}{x} \\ $$$${x}=\frac{\mathrm{285714}}{\mathrm{999999}}=\frac{\mathrm{2}}{\mathrm{7}}=\frac{{a}}{{b}} \\ $$
Commented by mathocean1 last updated on 18/Nov/20
$${thank}\:{you}\:{very}\:{much}\:{sir} \\ $$
Answered by liberty last updated on 19/Nov/20
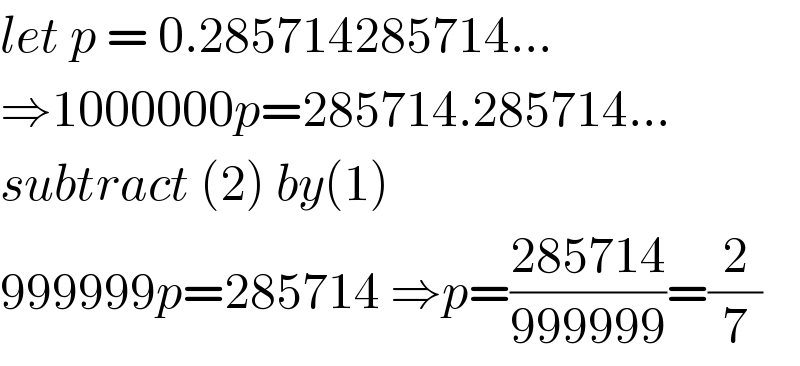
$${let}\:{p}\:=\:\mathrm{0}.\mathrm{285714285714}... \\ $$$$\Rightarrow\mathrm{1000000}{p}=\mathrm{285714}.\mathrm{285714}... \\ $$$${subtract}\:\left(\mathrm{2}\right)\:{by}\left(\mathrm{1}\right) \\ $$$$\mathrm{999999}{p}=\mathrm{285714}\:\Rightarrow{p}=\frac{\mathrm{285714}}{\mathrm{999999}}=\frac{\mathrm{2}}{\mathrm{7}} \\ $$
