Question and Answers Forum

All Questions      Topic List

Permutation and Combination Questions

Previous in All Question      Next in All Question      

Previous in Permutation and Combination      Next in Permutation and Combination      

Question Number 124709 by benjo_mathlover last updated on 05/Dec/20

Between 20000 and 70000 find the number of even integers in which no digits is repeated

$${Between}\:\mathrm{20000}\:{and}\:\mathrm{70000}\: \\ $$$${find}\:{the}\:{number}\:{of}\:{even}\:{integers} \\ $$$${in}\:{which}\:{no}\:{digits}\:{is}\:{repeated} \\ $$

Answered by liberty last updated on 05/Dec/20

Let abcde be a required even integer. As shown in the following diagram, the 1^(st) digit can be chosen from { 2,3,4,5,6} and the 5^(th) digit e can be chosen from {0,2,4,6,8}. ⌊ a ⌋ ⌊ b c d ⌋ ⌊e ⌋ since {2,3,4,5,6} ∩ { 0,2,4,6,8}= {2,4,6} we devide the problem into 2 disjoint cases case(1) a ∈ {2,4,6} there are = 3×4×P _3^8 case(2) a ∈ {3,5} there are = 2×5×P _3^8 The total number of required even numbers is 4032 + 3360 = 7392 .

$${Let}\:{abcde}\:{be}\:{a}\:{required}\:{even}\:{integer}.\: \\ $$$${As}\:{shown}\:{in}\:{the}\:{following}\:{diagram},\:{the} \\ $$$$\mathrm{1}^{{st}} \:{digit}\:{can}\:{be}\:{chosen}\:{from}\:\left\{\:\mathrm{2},\mathrm{3},\mathrm{4},\mathrm{5},\mathrm{6}\right\} \\ $$$${and}\:{the}\:\mathrm{5}^{{th}} \:{digit}\:{e}\:{can}\:{be}\:{chosen}\:{from}\: \\ $$$$\left\{\mathrm{0},\mathrm{2},\mathrm{4},\mathrm{6},\mathrm{8}\right\}. \\ $$$$\:\lfloor\:\:{a}\:\rfloor\:\lfloor\:{b}\:{c}\:{d}\:\rfloor\:\lfloor{e}\:\rfloor\: \\ $$$${since}\:\left\{\mathrm{2},\mathrm{3},\mathrm{4},\mathrm{5},\mathrm{6}\right\}\:\cap\:\left\{\:\mathrm{0},\mathrm{2},\mathrm{4},\mathrm{6},\mathrm{8}\right\}=\:\left\{\mathrm{2},\mathrm{4},\mathrm{6}\right\} \\ $$$${we}\:{devide}\:{the}\:{problem}\:{into}\:\mathrm{2}\:{disjoint}\:{cases} \\ $$$${case}\left(\mathrm{1}\right)\:{a}\:\in\:\left\{\mathrm{2},\mathrm{4},\mathrm{6}\right\}\:{there}\:{are}\:=\:\mathrm{3}×\mathrm{4}×{P}\:_{\mathrm{3}} ^{\mathrm{8}} \\ $$$${case}\left(\mathrm{2}\right)\:{a}\:\in\:\left\{\mathrm{3},\mathrm{5}\right\}\:{there}\:{are}\:=\:\mathrm{2}×\mathrm{5}×{P}\:_{\mathrm{3}} ^{\mathrm{8}} \\ $$$${The}\:{total}\:{number}\:{of}\:{required}\:{even}\:{numbers} \\ $$$${is}\:\mathrm{4032}\:+\:\mathrm{3360}\:=\:\mathrm{7392}\:. \\ $$

Answered by mr W last updated on 06/Dec/20

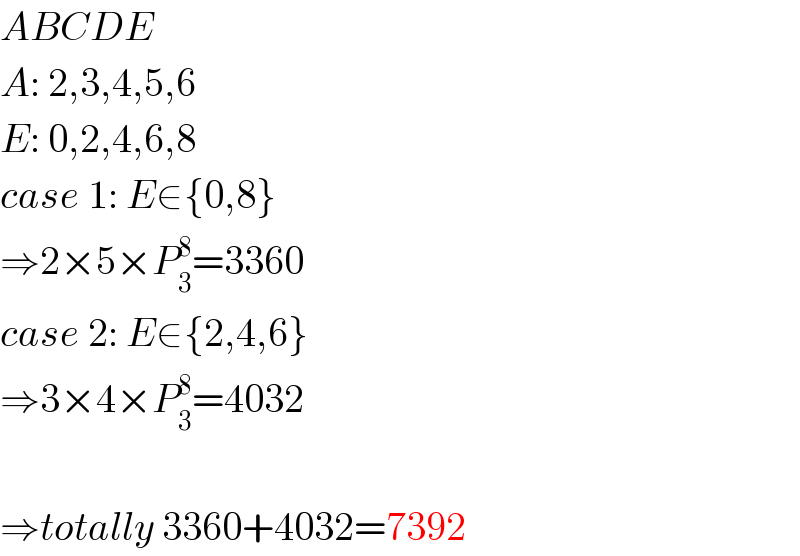
ABCDE A: 2,3,4,5,6 E: 0,2,4,6,8 case 1: E∈{0,8} ⇒2×5×P_3 ^8 =3360 case 2: E∈{2,4,6} ⇒3×4×P_3 ^8 =4032 ⇒totally 3360+4032=7392

$${ABCDE} \\ $$$${A}:\:\mathrm{2},\mathrm{3},\mathrm{4},\mathrm{5},\mathrm{6} \\ $$$${E}:\:\mathrm{0},\mathrm{2},\mathrm{4},\mathrm{6},\mathrm{8} \\ $$$${case}\:\mathrm{1}:\:{E}\in\left\{\mathrm{0},\mathrm{8}\right\} \\ $$$$\Rightarrow\mathrm{2}×\mathrm{5}×{P}_{\mathrm{3}} ^{\mathrm{8}} =\mathrm{3360} \\ $$$${case}\:\mathrm{2}:\:{E}\in\left\{\mathrm{2},\mathrm{4},\mathrm{6}\right\} \\ $$$$\Rightarrow\mathrm{3}×\mathrm{4}×{P}_{\mathrm{3}} ^{\mathrm{8}} =\mathrm{4032} \\ $$$$ \\ $$$$\Rightarrow{totally}\:\mathrm{3360}+\mathrm{4032}=\mathrm{7392} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com