Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 137363 by liberty last updated on 02/Apr/21

As illustrated, the rectangle has an area of 1, and E is the midpoint of AD. BF is one third of AB. What is the area of the shadow?

$$ \\ $$As illustrated, the rectangle has an area of 1, and E is the midpoint of AD. BF is one third of AB. What is the area of the shadow?

Commented by liberty last updated on 02/Apr/21

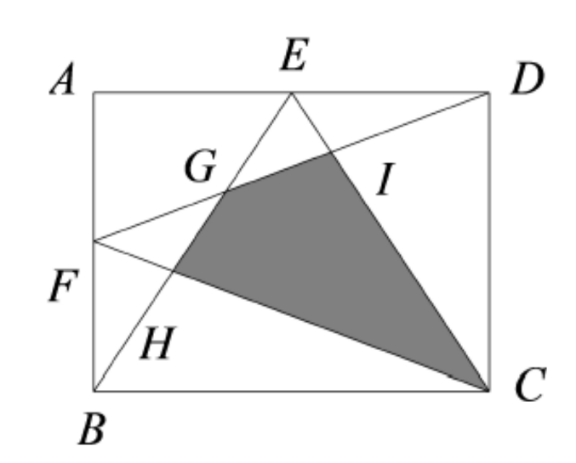
Answered by mr W last updated on 02/Apr/21

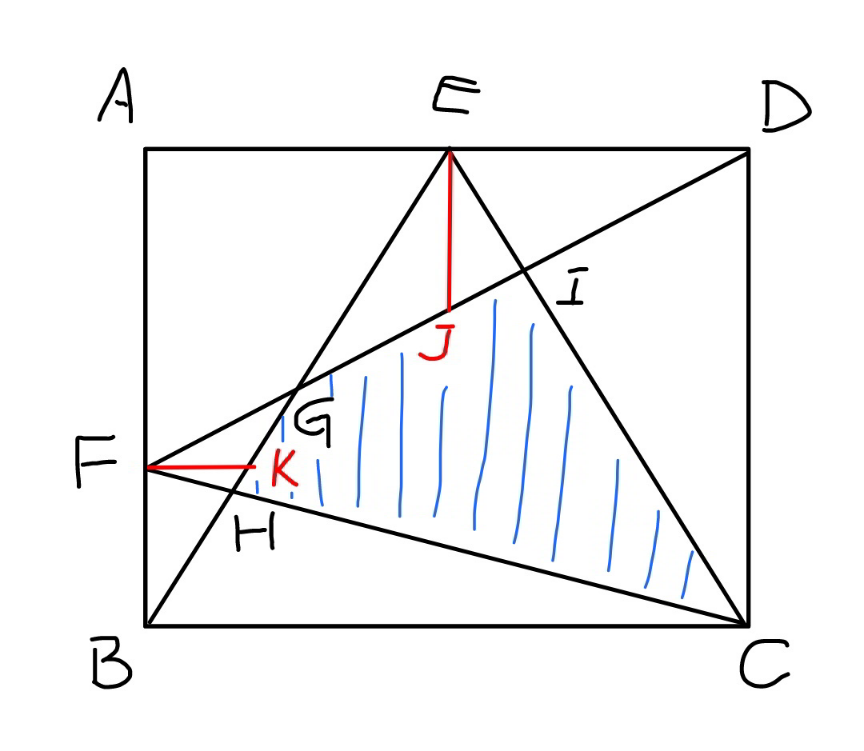
Commented by mr W last updated on 02/Apr/21

EJ=((AF)/2)=BF ⇒BG=GE EJ=((AF)/2)=(1/2)×(2/3)AB=((AB)/3)=((DC)/3) ⇒EI=((IC)/3)=((EC)/4) FK=((AE)/3)=((BC)/6) ⇒BK=6×HK=(6/7)×BK=(6/7)×((BE)/3)=(2/7)×BE ΔBHC=(2/7)×ΔBEC ΔEGI=(1/4)×ΔEGC=(1/8)×ΔBEC shados=(1−(2/7)−(1/8))×ΔBEC =((33)/(56))×ΔBEC =((33)/(56))×(1/2)=((33)/(112))

$${EJ}=\frac{{AF}}{\mathrm{2}}={BF} \\ $$$$\Rightarrow{BG}={GE} \\ $$$${EJ}=\frac{{AF}}{\mathrm{2}}=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{2}}{\mathrm{3}}{AB}=\frac{{AB}}{\mathrm{3}}=\frac{{DC}}{\mathrm{3}} \\ $$$$\Rightarrow{EI}=\frac{{IC}}{\mathrm{3}}=\frac{{EC}}{\mathrm{4}} \\ $$$${FK}=\frac{{AE}}{\mathrm{3}}=\frac{{BC}}{\mathrm{6}} \\ $$$$\Rightarrow{BK}=\mathrm{6}×{HK}=\frac{\mathrm{6}}{\mathrm{7}}×{BK}=\frac{\mathrm{6}}{\mathrm{7}}×\frac{{BE}}{\mathrm{3}}=\frac{\mathrm{2}}{\mathrm{7}}×{BE} \\ $$$$\Delta{BHC}=\frac{\mathrm{2}}{\mathrm{7}}×\Delta{BEC} \\ $$$$\Delta{EGI}=\frac{\mathrm{1}}{\mathrm{4}}×\Delta{EGC}=\frac{\mathrm{1}}{\mathrm{8}}×\Delta{BEC} \\ $$$${shados}=\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{7}}−\frac{\mathrm{1}}{\mathrm{8}}\right)×\Delta{BEC} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{33}}{\mathrm{56}}×\Delta{BEC} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{33}}{\mathrm{56}}×\frac{\mathrm{1}}{\mathrm{2}}=\frac{\mathrm{33}}{\mathrm{112}} \\ $$

Answered by EDWIN88 last updated on 02/Apr/21

we can make simpler by considering this a 12×12 square with B at origin. We have to remember to divide by 12^2 at the end. We get coordinates B(0,0) ,A(0,12),F(0,4) E(6,12), D(12,12),C(12,0). we need to? calculate G,H,I. G is the meet if BE and DF ⇒ G(3,6); H(((12)/7),((24)/7)) ; I(((15)/2), 9). The shadow area is = (1/2) ∣ determinant (((12 0)),((((12)/7) ((24)/7))))+ determinant (((((12)/7) ((24)/7))),(( 3 6)))+ determinant (((3 6)),((((15)/2) 9)))+ determinant (((((15)/2) 9)),((12 0)))∣ =(1/2)∣((288)/7) + 0 −18−108 ∣ =(1/2). ((594)/7) = ((297)/7) .Therefore we get the shadow area is ((297)/(7×144)) = ((33)/(112))

$$\mathrm{we}\:\mathrm{can}\:\mathrm{make}\:\mathrm{simpler}\:\mathrm{by}\:\mathrm{considering}\:\mathrm{this} \\ $$$$\mathrm{a}\:\mathrm{12}×\mathrm{12}\:\mathrm{square}\:\mathrm{with}\:\mathrm{B}\:\mathrm{at}\:\mathrm{origin}.\:\mathrm{We}\:\mathrm{have} \\ $$$$\mathrm{to}\:\mathrm{remember}\:\mathrm{to}\:\mathrm{divide}\:\mathrm{by}\:\mathrm{12}^{\mathrm{2}} \:\mathrm{at}\:\mathrm{the}\:\mathrm{end}. \\ $$$$\mathrm{We}\:\mathrm{get}\:\mathrm{coordinates}\:\mathrm{B}\left(\mathrm{0},\mathrm{0}\right)\:,\mathrm{A}\left(\mathrm{0},\mathrm{12}\right),\mathrm{F}\left(\mathrm{0},\mathrm{4}\right) \\ $$$$\mathrm{E}\left(\mathrm{6},\mathrm{12}\right),\:\mathrm{D}\left(\mathrm{12},\mathrm{12}\right),\mathrm{C}\left(\mathrm{12},\mathrm{0}\right).\:\mathrm{we}\:\mathrm{need}\:\mathrm{to}? \\ $$$$\mathrm{calculate}\:\mathrm{G},\mathrm{H},\mathrm{I}.\:\mathrm{G}\:\mathrm{is}\:\mathrm{the}\:\mathrm{meet}\:\mathrm{if}\:\mathrm{BE}\:\mathrm{and}\:\mathrm{DF} \\ $$$$\:\Rightarrow\:\mathrm{G}\left(\mathrm{3},\mathrm{6}\right);\:\mathrm{H}\left(\frac{\mathrm{12}}{\mathrm{7}},\frac{\mathrm{24}}{\mathrm{7}}\right)\:;\:\mathrm{I}\left(\frac{\mathrm{15}}{\mathrm{2}},\:\mathrm{9}\right).\: \\ $$$$\mathrm{The}\:\mathrm{shadow}\:\mathrm{area}\:\mathrm{is}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}\:\mid\:\begin{vmatrix}{\mathrm{12}\:\:\:\:\mathrm{0}}\\{\frac{\mathrm{12}}{\mathrm{7}}\:\:\:\:\frac{\mathrm{24}}{\mathrm{7}}}\end{vmatrix}+\begin{vmatrix}{\frac{\mathrm{12}}{\mathrm{7}}\:\:\:\:\frac{\mathrm{24}}{\mathrm{7}}}\\{\:\mathrm{3}\:\:\:\:\:\:\:\mathrm{6}}\end{vmatrix}+\begin{vmatrix}{\mathrm{3}\:\:\:\:\mathrm{6}}\\{\frac{\mathrm{15}}{\mathrm{2}}\:\:\mathrm{9}}\end{vmatrix}+\begin{vmatrix}{\frac{\mathrm{15}}{\mathrm{2}}\:\:\:\mathrm{9}}\\{\mathrm{12}\:\:\:\mathrm{0}}\end{vmatrix}\mid \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\mid\frac{\mathrm{288}}{\mathrm{7}}\:+\:\mathrm{0}\:−\mathrm{18}−\mathrm{108}\:\mid \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}.\:\frac{\mathrm{594}}{\mathrm{7}}\:=\:\frac{\mathrm{297}}{\mathrm{7}}\:.\mathrm{Therefore}\:\mathrm{we}\:\mathrm{get}\:\mathrm{the} \\ $$$$\mathrm{shadow}\:\mathrm{area}\:\mathrm{is}\:\frac{\mathrm{297}}{\mathrm{7}×\mathrm{144}}\:=\:\frac{\mathrm{33}}{\mathrm{112}}\: \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com