Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 999

Question Number 118917 Answers: 0 Comments: 1

x(x+y)dy−x^2 dx

$${x}\left({x}+{y}\right){dy}−{x}^{\mathrm{2}} {dx} \\ $$

Question Number 118936 Answers: 3 Comments: 0

show by recurrence that: for n ∈ N^∗ , 2^(6n−5) +3^(2n ) is divisible by 11.

$${show}\:{by}\:{recurrence}\:{that}: \\ $$$${for}\:{n}\:\in\:\mathbb{N}^{\ast} ,\:\mathrm{2}^{\mathrm{6}{n}−\mathrm{5}} +\mathrm{3}^{\mathrm{2}{n}\:} \:{is}\:{divisible}\:{by} \\ $$$$\mathrm{11}. \\ $$

Question Number 118907 Answers: 0 Comments: 0

where can u fond a formula for denesting ramanujan type roots?

$${where}\:{can}\:{u}\:{fond}\:{a}\:{formula}\:{for} \\ $$$${denesting}\:{ramanujan}\:{type}\:{roots}? \\ $$

Question Number 118905 Answers: 4 Comments: 0

∫ (dλ/((λ^2 −9)^2 )) =?

$$\:\:\:\int\:\frac{{d}\lambda}{\left(\lambda^{\mathrm{2}} −\mathrm{9}\right)^{\mathrm{2}} }\:=?\: \\ $$

Question Number 118896 Answers: 1 Comments: 0

Question Number 118899 Answers: 2 Comments: 0

sin ((π/(14)))sin (((2π)/(14)))sin (((3π)/(14)))...sin (((6π)/(14)))=?

$$\:\mathrm{sin}\:\left(\frac{\pi}{\mathrm{14}}\right)\mathrm{sin}\:\left(\frac{\mathrm{2}\pi}{\mathrm{14}}\right)\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{14}}\right)...\mathrm{sin}\:\left(\frac{\mathrm{6}\pi}{\mathrm{14}}\right)=? \\ $$

Question Number 118891 Answers: 2 Comments: 0

∫_0 ^π arctan(3^(cosx) )dx=??? please help

$$\:\int_{\mathrm{0}} ^{\pi} \boldsymbol{{arctan}}\left(\mathrm{3}^{\boldsymbol{{cosx}}} \right)\boldsymbol{{dx}}=??? \\ $$$$ \\ $$$$\boldsymbol{{please}}\:\boldsymbol{{help}} \\ $$

Question Number 118887 Answers: 2 Comments: 0

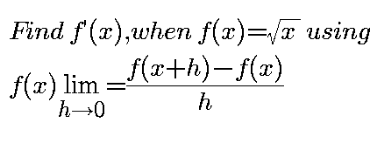
Question Number 118886 Answers: 1 Comments: 0

... advanced calculus... evaluate :: {_(2. Ω_2 = ∫_0 ^( (1/2)) ((ln^2 (1+x))/x) dx=??) ^(1. Ω_1 =∫_0 ^( (1/2)) ((ln^2 (1−x))/x)dx=??) ... M.N.1970...

$$\:\:\:\:\:\:\:\:\:\:...\:\:{advanced}\:\:{calculus}... \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:{evaluate}\:::\:\:\left\{_{\mathrm{2}.\:\Omega_{\mathrm{2}} =\:\int_{\mathrm{0}} ^{\:\frac{\mathrm{1}}{\mathrm{2}}} \:\frac{{ln}^{\mathrm{2}} \left(\mathrm{1}+{x}\right)}{{x}}\:{dx}=??} ^{\mathrm{1}.\:\Omega_{\mathrm{1}} =\int_{\mathrm{0}} ^{\:\frac{\mathrm{1}}{\mathrm{2}}} \:\frac{{ln}^{\mathrm{2}} \left(\mathrm{1}−{x}\right)}{{x}}{dx}=??} \right. \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:\mathscr{M}.\mathscr{N}.\mathrm{1970}... \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Question Number 118880 Answers: 3 Comments: 0

8(1+(1/2))(1+(1/2^2 ))(1+(1/2^4 ))(1+(1/2^8 ))...(1+(1/2^(32) ))+ (1/2^(60) )=?

$$\mathrm{8}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{4}} }\right)\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{8}} }\right)...\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{32}} }\right)+\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{60}} }=? \\ $$

Question Number 118876 Answers: 3 Comments: 0

Find the value of ⌊ (((3+(√(17)))/2))^6 ⌋ .

$${Find}\:{the}\:{value}\:{of}\:\lfloor\:\left(\frac{\mathrm{3}+\sqrt{\mathrm{17}}}{\mathrm{2}}\right)^{\mathrm{6}} \:\rfloor\:. \\ $$

Question Number 118875 Answers: 0 Comments: 0

CAS ^1 H=1.008 u 99.985% ^2 H=2.014 u 0.013% 1.008•((99.985)/(100))+2.014•((0.013)/(100))=1.0078u+0.0003=1.0081u

$$\mathrm{CAS} \\ $$$$\:^{\mathrm{1}} \mathrm{H}=\mathrm{1}.\mathrm{008}\:\mathrm{u}\:\:\:\:\:\mathrm{99}.\mathrm{985\%} \\ $$$$\:^{\mathrm{2}} \mathrm{H}=\mathrm{2}.\mathrm{014}\:\mathrm{u}\:\:\:\:\:\mathrm{0}.\mathrm{013\%} \\ $$$$\mathrm{1}.\mathrm{008}\bullet\frac{\mathrm{99}.\mathrm{985}}{\mathrm{100}}+\mathrm{2}.\mathrm{014}\bullet\frac{\mathrm{0}.\mathrm{013}}{\mathrm{100}}=\mathrm{1}.\mathrm{0078u}+\mathrm{0}.\mathrm{0003}=\mathrm{1}.\mathrm{0081u} \\ $$$$ \\ $$

Question Number 118874 Answers: 1 Comments: 0

In xy−plane , which of the following is the reflection of the graph of y = ((1+x)/(x^2 +1)) about the line y=2x.

$${In}\:{xy}−{plane}\:,\:{which}\:{of}\:{the}\:{following} \\ $$$${is}\:{the}\:{reflection}\:{of}\:{the}\:{graph} \\ $$$${of}\:{y}\:=\:\frac{\mathrm{1}+{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\:{about}\:{the}\:{line}\:{y}=\mathrm{2}{x}.\: \\ $$

Question Number 118872 Answers: 1 Comments: 1

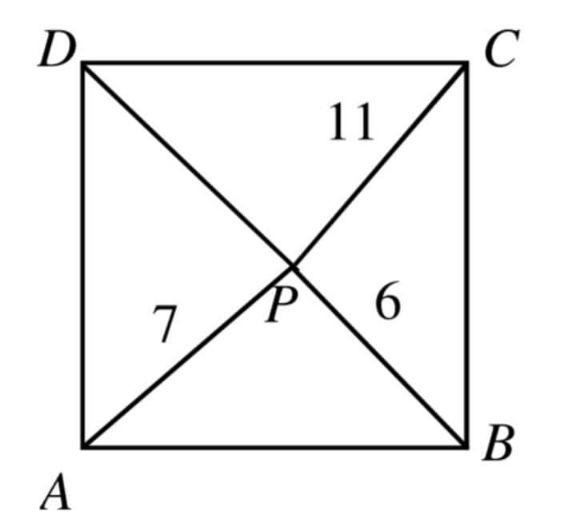
Question Number 118868 Answers: 1 Comments: 0

If the graphs of y=x^2 +2ax+6b and y=x^2 +2bx+6a intersect at? only one point in the xy−plane , what is the x−coordinate of the point of intersection ?

$${If}\:{the}\:{graphs}\:{of}\:{y}={x}^{\mathrm{2}} +\mathrm{2}{ax}+\mathrm{6}{b}\: \\ $$$${and}\:{y}={x}^{\mathrm{2}} +\mathrm{2}{bx}+\mathrm{6}{a}\:{intersect}\:{at}? \\ $$$${only}\:{one}\:{point}\:{in}\:{the}\:{xy}−{plane} \\ $$$$,\:{what}\:{is}\:{the}\:{x}−{coordinate}\:{of}\:{the} \\ $$$${point}\:{of}\:{intersection}\:? \\ $$

Question Number 118861 Answers: 1 Comments: 0

sin^(−1) (−1/2)

$${sin}^{−\mathrm{1}} \left(−\mathrm{1}/\mathrm{2}\right) \\ $$

Question Number 118966 Answers: 1 Comments: 0

lim_(x→0) (((√(x+bx^2 ))−(√x))/(bx(√x))) =?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{{x}+{bx}^{\mathrm{2}} }−\sqrt{{x}}}{{bx}\sqrt{{x}}}\:=? \\ $$

Question Number 118849 Answers: 1 Comments: 2

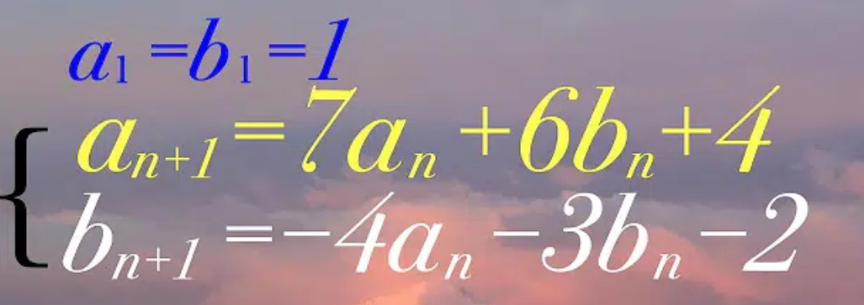
Question Number 118841 Answers: 1 Comments: 1

Question Number 118839 Answers: 0 Comments: 1

Question Number 118834 Answers: 2 Comments: 0

∫ ((x^4 +1)/(x^5 +4x^3 )) dx

$$\:\:\int\:\frac{{x}^{\mathrm{4}} +\mathrm{1}}{{x}^{\mathrm{5}} +\mathrm{4}{x}^{\mathrm{3}} }\:{dx}\: \\ $$

Question Number 118857 Answers: 0 Comments: 0

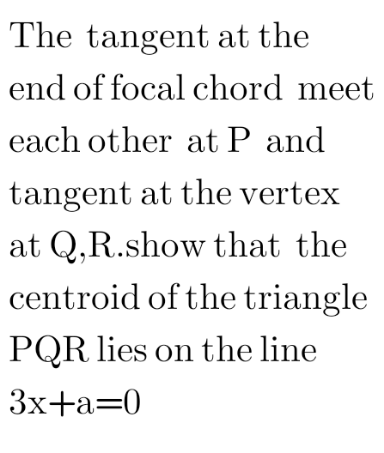
Question Number 118825 Answers: 2 Comments: 0

I am thinking of a two−digit number .If i write 3 to the left of my number and double this three digit number the result is 27 times my original number. what is my number ?

$${I}\:{am}\:{thinking}\:{of}\:{a}\:{two}−{digit}\:{number} \\ $$$$.{If}\:{i}\:{write}\:\mathrm{3}\:{to}\:{the}\:{left}\:{of}\:{my}\:{number} \\ $$$${and}\:{double}\:{this}\:{three}\:{digit}\:{number}\:{the} \\ $$$${result}\:{is}\:\mathrm{27}\:{times}\:{my}\:{original}\:{number}. \\ $$$${what}\:{is}\:{my}\:{number}\:? \\ $$

Question Number 118822 Answers: 2 Comments: 0

In how many ways can the letters of the word LEVITATE be arranged if the vowels must not be next to each other

$$\:\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{the}\:\mathrm{letters}\:\mathrm{of}\: \\ $$$$\:\mathrm{the}\:\mathrm{word}\:{LEVITATE}\:\mathrm{be}\:\mathrm{arranged}\:\mathrm{if} \\ $$$$\:\mathrm{the}\:\mathrm{vowels}\:\mathrm{must}\:\mathrm{not}\:\mathrm{be}\:\mathrm{next}\:\mathrm{to}\:\mathrm{each} \\ $$$$\:\mathrm{other} \\ $$

Question Number 118819 Answers: 3 Comments: 0

∫((x^4 +x^2 +1)/((x^2 +4)^2 (x^2 +1))) dx

$$\int\frac{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}}{\left({x}^{\mathrm{2}} +\mathrm{4}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{1}\right)}\:{dx}\: \\ $$

Question Number 118813 Answers: 1 Comments: 0

(d^2 y/dx^2 ) −4x (dy/dx) + y(3x^2 −2)= 0

$$\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\:−\mathrm{4}{x}\:\frac{{dy}}{{dx}}\:+\:{y}\left(\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}\right)=\:\mathrm{0}\: \\ $$

Pg 994 Pg 995 Pg 996 Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002 Pg 1003

Terms of Service

Contact: info@tinkutara.com