Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 756

Question Number 142865 Answers: 1 Comments: 1

Let a_1 , a_2 , a_3 , ... be an arithmethic progression of positive real numbers. Then (1/( (√a_1 )+(√a_2 )))+(1/( (√a_2 )+(√a_3 )))+∙∙∙+(1/( (√a_(n−1) )+(√a_n )))= (A) ((n+1)/( (√a_1 )+(√a_n ))) (B) ((n−1)/( (√a_1 )+(√a_n ))) (C) (n/( (√a_1 )+(√a_n ))) (D) (n/( (√a_n )−(√a_1 )))

$$\mathrm{Let}\:{a}_{\mathrm{1}} ,\:{a}_{\mathrm{2}} ,\:{a}_{\mathrm{3}} ,\:...\:\mathrm{be}\:\mathrm{an}\:\mathrm{arithmethic}\:\mathrm{progression}\:\mathrm{of} \\ $$$$\mathrm{positive}\:\mathrm{real}\:\mathrm{numbers}.\:\mathrm{Then} \\ $$$$\:\:\:\:\:\:\:\frac{\mathrm{1}}{\:\sqrt{{a}_{\mathrm{1}} }+\sqrt{{a}_{\mathrm{2}} }}+\frac{\mathrm{1}}{\:\sqrt{{a}_{\mathrm{2}} }+\sqrt{{a}_{\mathrm{3}} }}+\centerdot\centerdot\centerdot+\frac{\mathrm{1}}{\:\sqrt{{a}_{{n}−\mathrm{1}} }+\sqrt{{a}_{{n}} }}= \\ $$$$\left(\mathrm{A}\right)\:\frac{{n}+\mathrm{1}}{\:\sqrt{{a}_{\mathrm{1}} }+\sqrt{{a}_{{n}} }}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\frac{{n}−\mathrm{1}}{\:\sqrt{{a}_{\mathrm{1}} }+\sqrt{{a}_{{n}} }} \\ $$$$\left(\mathrm{C}\right)\:\frac{{n}}{\:\sqrt{{a}_{\mathrm{1}} }+\sqrt{{a}_{{n}} }}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\frac{{n}}{\:\sqrt{{a}_{{n}} }−\sqrt{{a}_{\mathrm{1}} }} \\ $$

Question Number 142570 Answers: 1 Comments: 0

Find all functions f:R→R such that f(x+y)=2f(x)+3f(y)−4xyf(2x−3y) (∀x;y∈R)

$${Find}\:{all}\:{functions}\:{f}:\mathbb{R}\rightarrow\mathbb{R}\:{such}\:{that} \\ $$$${f}\left({x}+{y}\right)=\mathrm{2}{f}\left({x}\right)+\mathrm{3}{f}\left({y}\right)−\mathrm{4}{xyf}\left(\mathrm{2}{x}−\mathrm{3}{y}\right) \\ $$$$\left(\forall{x};{y}\in\mathbb{R}\right) \\ $$

Question Number 142582 Answers: 2 Comments: 0

z^4 + 12z + 3 = 0 (z=?)

$$\boldsymbol{{z}}^{\mathrm{4}} \:+\:\mathrm{12}\boldsymbol{{z}}\:+\:\mathrm{3}\:=\:\mathrm{0}\:\left(\boldsymbol{{z}}=?\right) \\ $$

Question Number 142577 Answers: 2 Comments: 0

Let a,b,c ≥ 0 and a+b+c = 1.Prove that (1/(a^2 +1))+(1/(b^2 +1))+(1/(c^2 +1)) ≥ (5/2)

$$\mathrm{Let}\:{a},{b},{c}\:\geqslant\:\mathrm{0}\:\mathrm{and}\:{a}+{b}+{c}\:=\:\mathrm{1}.\mathrm{Prove}\:\mathrm{that}\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{{a}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{1}}{{b}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{1}}{{c}^{\mathrm{2}} +\mathrm{1}}\:\geqslant\:\frac{\mathrm{5}}{\mathrm{2}} \\ $$$$ \\ $$

Question Number 142560 Answers: 1 Comments: 0

Question Number 142558 Answers: 0 Comments: 0

find the solution of x^2 =5^(log_2 x) −3^(log_2 x) on R.

$$\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{x}^{\mathrm{2}} =\mathrm{5}^{\mathrm{log}_{\mathrm{2}} \mathrm{x}} −\mathrm{3}^{\mathrm{log}_{\mathrm{2}} \mathrm{x}} \:\mathrm{on}\:\mathbb{R}. \\ $$

Question Number 142556 Answers: 1 Comments: 0

{ ((x^2 +y^2 =2yz+2−z^2 )),((z=4022−x−y)) :} x,y,z∈Z^+ . then z=

$$\begin{cases}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{2}{yz}+\mathrm{2}−{z}^{\mathrm{2}} }\\{{z}=\mathrm{4022}−{x}−{y}}\end{cases} \\ $$$$\:{x},{y},{z}\in\mathbb{Z}^{+} \:.\:{then}\:{z}= \\ $$

Question Number 142553 Answers: 2 Comments: 0

Evaluate : ∫_0 ^1 ((log(x)log((x/(1−x))))/( (√(x/(1−x))))) dx

$$ \\ $$$$\:\:\:\:{Evaluate}\::\: \\ $$$$\:\:\:\:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{log}\left({x}\right){log}\left(\frac{{x}}{\mathrm{1}−{x}}\right)}{\:\sqrt{\frac{{x}}{\mathrm{1}−{x}}}}\:{dx} \\ $$

Question Number 142548 Answers: 0 Comments: 1

Question Number 142546 Answers: 0 Comments: 1

i need help

$${i}\:{need}\:{help} \\ $$

Question Number 142545 Answers: 1 Comments: 0

nice .....integral Ω:=∫_(−∞) ^( +∞) (dx/((x^2 +π^2 )cosh(x))) =? .....

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{nice}\:.....{integral} \\ $$$$\:\:\:\:\:\Omega:=\int_{−\infty} ^{\:\:+\infty} \frac{{dx}}{\left({x}^{\mathrm{2}} +\pi^{\mathrm{2}} \right){cosh}\left({x}\right)}\:=? \\ $$$$..... \\ $$

Question Number 142538 Answers: 1 Comments: 0

2x^7 +x^(28) =3x^(21) find x

$$\mathrm{2}\boldsymbol{{x}}^{\mathrm{7}} +\boldsymbol{{x}}^{\mathrm{28}} =\mathrm{3}\boldsymbol{{x}}^{\mathrm{21}} \\ $$$$\boldsymbol{\mathrm{find}}\:\boldsymbol{\mathrm{x}} \\ $$

Question Number 142537 Answers: 0 Comments: 0

Given: z_1 =e^(i(π/3)) (z+3)−3 and z_2 =e^(−i((2π)/3)) (z−3)+3. Show that ((z_2 −z)/(z_1 −z))=i(√3)((z−3)/(z+3))

$$\mathrm{Given}: \\ $$$$\mathrm{z}_{\mathrm{1}} =\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{3}}} \left(\mathrm{z}+\mathrm{3}\right)−\mathrm{3}\:\mathrm{and}\:\mathrm{z}_{\mathrm{2}} =\mathrm{e}^{−\mathrm{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \left(\mathrm{z}−\mathrm{3}\right)+\mathrm{3}. \\ $$$$\mathrm{Show}\:\mathrm{that}\: \\ $$$$\frac{\mathrm{z}_{\mathrm{2}} −\mathrm{z}}{\mathrm{z}_{\mathrm{1}} −\mathrm{z}}=\mathrm{i}\sqrt{\mathrm{3}}\frac{\mathrm{z}−\mathrm{3}}{\mathrm{z}+\mathrm{3}} \\ $$

Question Number 142534 Answers: 0 Comments: 0

Question Number 142531 Answers: 0 Comments: 0

if 𝛗 (q):= ∫_1 ^( ∞) (1/( (√x) (q+x)^x ))dx then :: lim _(q→1) 𝛗(q):=?

$$\: \\ $$$$\:\:{if}\:\:\:\:\boldsymbol{\phi}\:\left({q}\right):=\:\int_{\mathrm{1}} ^{\:\infty} \frac{\mathrm{1}}{\:\sqrt{{x}}\:\left({q}+{x}\right)^{{x}} }{dx} \\ $$$$\:\:\:\:{then}\:::\:\:{lim}\:_{{q}\rightarrow\mathrm{1}} \boldsymbol{\phi}\left({q}\right):=? \\ $$$$\:\:\:\:\:\: \\ $$

Question Number 142528 Answers: 1 Comments: 0

∫_1 ^( ∞) (dx/(e^x −2^x ))

$$\int_{\mathrm{1}} ^{\:\infty} \:\frac{{dx}}{{e}^{{x}} −\mathrm{2}^{{x}} } \\ $$

Question Number 142516 Answers: 0 Comments: 0

∫(dx/((−lnx)^(1/x) ))

$$\int\frac{{dx}}{\left(−{lnx}\right)^{\frac{\mathrm{1}}{{x}}} }\:\: \\ $$

Question Number 142514 Answers: 5 Comments: 0

..... number theory..... Solve in Z : (1/x)+(1/y)+(1/(xy)) =(1/4) ....? .........

$$\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:.....\:{number}\:\:{theory}..... \\ $$$$\:\:\:\:\:\:\:{Solve}\:{in}\:\mathbb{Z}\:: \\ $$$$\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{xy}}\:=\frac{\mathrm{1}}{\mathrm{4}}\:....? \\ $$$$\:\:\:\:\:......... \\ $$

Question Number 142518 Answers: 0 Comments: 0

Question Number 142510 Answers: 0 Comments: 1

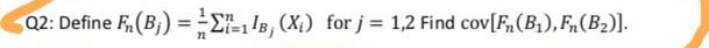
Question Number 142505 Answers: 0 Comments: 0

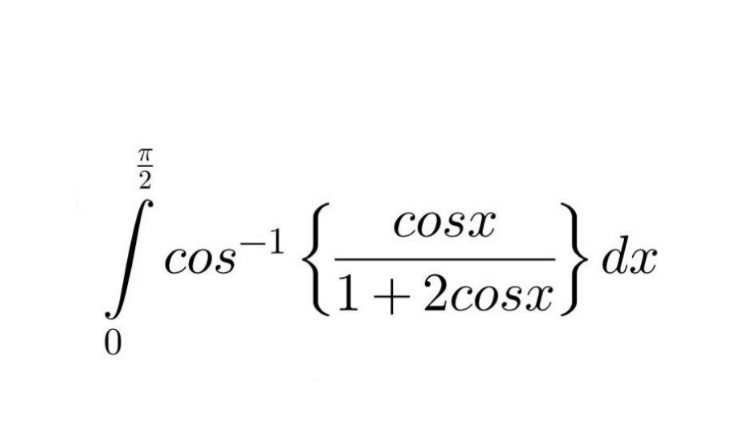
Question Number 142504 Answers: 1 Comments: 0

Question Number 142503 Answers: 1 Comments: 0

Question Number 142502 Answers: 0 Comments: 0

(dy/dx) y= 3a^x −cot 2x

$$\frac{{dy}}{{dx}}\: \\ $$$${y}=\:\mathrm{3}{a}^{{x}} −\mathrm{cot}\:\mathrm{2}{x} \\ $$

Question Number 142499 Answers: 2 Comments: 1

Question Number 142492 Answers: 1 Comments: 0

Prove that Σ_(n = 0) ^∞ (n/(3n^2 + 2)) diverges.

$$\mathrm{Prove}\:\mathrm{that}\:\underset{{n}\:=\:\mathrm{0}} {\overset{\infty} {\sum}}\frac{{n}}{\mathrm{3}{n}^{\mathrm{2}} \:+\:\mathrm{2}}\:\mathrm{diverges}. \\ $$

Pg 751 Pg 752 Pg 753 Pg 754 Pg 755 Pg 756 Pg 757 Pg 758 Pg 759 Pg 760

Terms of Service

Contact: info@tinkutara.com