
AllQuestion and Answers: Page 755
Question Number 142723 Answers: 1 Comments: 0
Question Number 142721 Answers: 1 Comments: 0
Question Number 142719 Answers: 0 Comments: 1
Question Number 142717 Answers: 2 Comments: 1

Question Number 142765 Answers: 1 Comments: 1
Question Number 142763 Answers: 2 Comments: 2

Question Number 142712 Answers: 0 Comments: 0

Question Number 142710 Answers: 1 Comments: 0
Question Number 142708 Answers: 1 Comments: 0
Question Number 142698 Answers: 1 Comments: 0
Question Number 142691 Answers: 1 Comments: 1
Question Number 142690 Answers: 1 Comments: 0
Question Number 142689 Answers: 1 Comments: 0

Question Number 142687 Answers: 1 Comments: 0
Question Number 142681 Answers: 0 Comments: 2
Question Number 142679 Answers: 0 Comments: 5
Question Number 142668 Answers: 3 Comments: 2
Question Number 142667 Answers: 1 Comments: 0
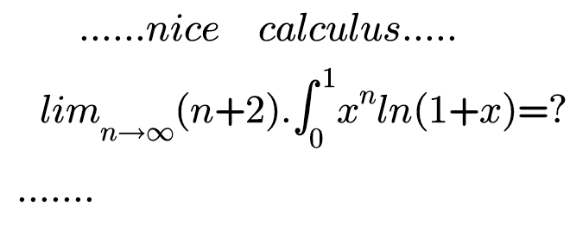
Question Number 142655 Answers: 2 Comments: 0
Question Number 142656 Answers: 2 Comments: 0
Question Number 142653 Answers: 2 Comments: 0
Question Number 142647 Answers: 2 Comments: 0
Question Number 142646 Answers: 1 Comments: 0
Question Number 142643 Answers: 1 Comments: 0
$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{cos}^{\mathrm{2}} {t}}{{sint}}{dt} \\ $$
Question Number 142639 Answers: 2 Comments: 2
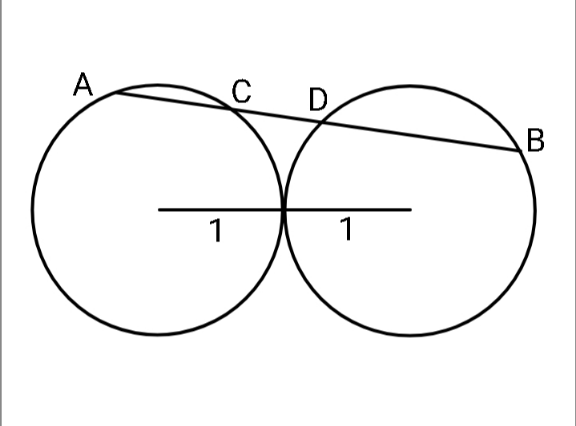
Question Number 142674 Answers: 1 Comments: 0
Pg 750 Pg 751 Pg 752 Pg 753 Pg 754 Pg 755 Pg 756 Pg 757 Pg 758 Pg 759
