Question and Answers Forum

AllQuestion and Answers: Page 752

Question Number 143350 Answers: 1 Comments: 0

if tan^2 αtan^2 β+tan^2 βtan^2 γ+ tan^2 γtan^2 α+2tan^2 αtan^2 βtan^2 γ=1 then find sin^2 α+sin^2 β+sin^2 γ

$$\mathrm{if}\:\mathrm{tan}^{\mathrm{2}} \alpha\mathrm{tan}^{\mathrm{2}} \beta+\mathrm{tan}^{\mathrm{2}} \beta\mathrm{tan}^{\mathrm{2}} \gamma+ \\ $$$$\mathrm{tan}^{\mathrm{2}} \gamma\mathrm{tan}^{\mathrm{2}} \alpha+\mathrm{2tan}^{\mathrm{2}} \alpha\mathrm{tan}^{\mathrm{2}} \beta\mathrm{tan}^{\mathrm{2}} \gamma=\mathrm{1} \\ $$$$\mathrm{then}\:\mathrm{find}\:\mathrm{sin}^{\mathrm{2}} \alpha+\mathrm{sin}^{\mathrm{2}} \beta+\mathrm{sin}^{\mathrm{2}} \gamma \\ $$

Question Number 143348 Answers: 1 Comments: 0

if ((cos^4 x)/(cos^2 y))+((sin^4 x)/(sin^2 y))=1 then find ((cos^4 y)/(cos^2 x))+((sin^4 y)/(sin^2 x))=?

$$\mathrm{if}\:\:\frac{\mathrm{cos}\:^{\mathrm{4}} \mathrm{x}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{y}}+\frac{\mathrm{sin}\:^{\mathrm{4}} \mathrm{x}}{\mathrm{sin}\:^{\mathrm{2}} \mathrm{y}}=\mathrm{1}\:\mathrm{then}\: \\ $$$$\mathrm{find}\:\frac{\mathrm{cos}\:^{\mathrm{4}} \mathrm{y}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}+\frac{\mathrm{sin}\:^{\mathrm{4}} \mathrm{y}}{\mathrm{sin}\:^{\mathrm{2}} \mathrm{x}}=? \\ $$

Question Number 143339 Answers: 1 Comments: 1

Question Number 143332 Answers: 2 Comments: 2

Question Number 143329 Answers: 1 Comments: 0

log_(2x+1) (x^2 +1)+log_(x+1) (3(√(2x+5))+18)=2x

$$\mathrm{log}_{\mathrm{2x}+\mathrm{1}} \left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)+\mathrm{log}_{\mathrm{x}+\mathrm{1}} \left(\mathrm{3}\sqrt{\mathrm{2x}+\mathrm{5}}+\mathrm{18}\right)=\mathrm{2x} \\ $$

Question Number 143328 Answers: 3 Comments: 0

x+(√(xy))+y=14 and x^2 +xy+y^2 =84 , find x and y

$${x}+\sqrt{{xy}}+{y}=\mathrm{14}\:\:{and}\: \\ $$$${x}^{\mathrm{2}} +{xy}+{y}^{\mathrm{2}} =\mathrm{84}\:, \\ $$$${find}\:{x}\:{and}\:{y} \\ $$

Question Number 143327 Answers: 1 Comments: 0

1/.lim_(n→+∝) ((6+((6+((6+..........+(6)^(1/3) ))^(1/3) ))^(1/3) ))^(1/3) =?

$$\mathrm{1}/.\underset{\mathrm{n}\rightarrow+\propto} {\mathrm{lim}}\sqrt[{\mathrm{3}}]{\mathrm{6}+\sqrt[{\mathrm{3}}]{\mathrm{6}+\sqrt[{\mathrm{3}}]{\mathrm{6}+..........+\sqrt[{\mathrm{3}}]{\mathrm{6}}}}}=? \\ $$

Question Number 143326 Answers: 1 Comments: 0

L= lim_(n→+∝) (((2^3 −1)(3^3 −1)(4^3 −1)...(n^3 −1))/((2^3 +1)(3^3 +1)(4^3 +1)...(n^3 +1)))

$$\mathrm{L}=\:\underset{\mathrm{n}\rightarrow+\propto} {\mathrm{lim}}\frac{\left(\mathrm{2}^{\mathrm{3}} −\mathrm{1}\right)\left(\mathrm{3}^{\mathrm{3}} −\mathrm{1}\right)\left(\mathrm{4}^{\mathrm{3}} −\mathrm{1}\right)...\left(\mathrm{n}^{\mathrm{3}} −\mathrm{1}\right)}{\left(\mathrm{2}^{\mathrm{3}} +\mathrm{1}\right)\left(\mathrm{3}^{\mathrm{3}} +\mathrm{1}\right)\left(\mathrm{4}^{\mathrm{3}} +\mathrm{1}\right)...\left(\mathrm{n}^{\mathrm{3}} +\mathrm{1}\right)} \\ $$

Question Number 143324 Answers: 2 Comments: 0

(x^(2x^(−(1/5)) ) )^(−1) =(1/(25)) solve for x

$$\left(\boldsymbol{{x}}^{\mathrm{2}\boldsymbol{{x}}^{−\frac{\mathrm{1}}{\mathrm{5}}} } \right)^{−\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{25}} \\ $$$$\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{for}}\:\:\boldsymbol{\mathrm{x}} \\ $$

Question Number 143322 Answers: 1 Comments: 0

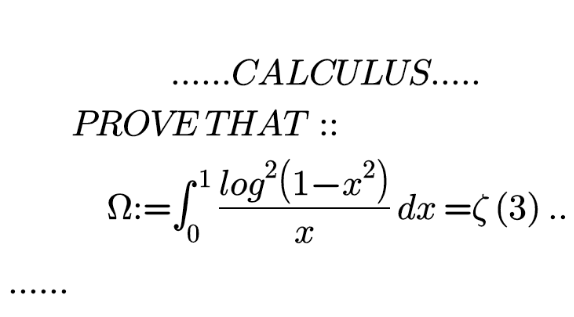
Question Number 143320 Answers: 3 Comments: 0

prove that: tan^2 36° + tan^2 72° = 5

$${prove}\:{that}:\:\:{tan}^{\mathrm{2}} \mathrm{36}°\:+\:{tan}^{\mathrm{2}} \mathrm{72}°\:=\:\mathrm{5} \\ $$

Question Number 143317 Answers: 0 Comments: 1

Question Number 143312 Answers: 1 Comments: 0

∫_0 ^∞ ((sin^4 x)/x^4 )dx=(π/3)

$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sin}^{\mathrm{4}} \mathrm{x}}{\mathrm{x}^{\mathrm{4}} }\mathrm{dx}=\frac{\pi}{\mathrm{3}} \\ $$

Question Number 143296 Answers: 3 Comments: 0

Montrer que Γ(n)=(n−1)!

$${Montrer}\:{que} \\ $$$$\Gamma\left({n}\right)=\left({n}−\mathrm{1}\right)! \\ $$$$ \\ $$

Question Number 143293 Answers: 2 Comments: 0

f(x)=ln(1+ln(x)). f(x)=ln(f′(x)).Find x

$${f}\left({x}\right)={ln}\left(\mathrm{1}+{ln}\left({x}\right)\right). \\ $$$${f}\left({x}\right)={ln}\left({f}'\left({x}\right)\right).{Find}\:{x} \\ $$

Question Number 143287 Answers: 2 Comments: 0

Question Number 143284 Answers: 1 Comments: 0

Question Number 143274 Answers: 3 Comments: 0

Question Number 143270 Answers: 1 Comments: 0

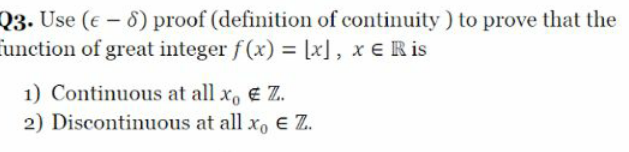
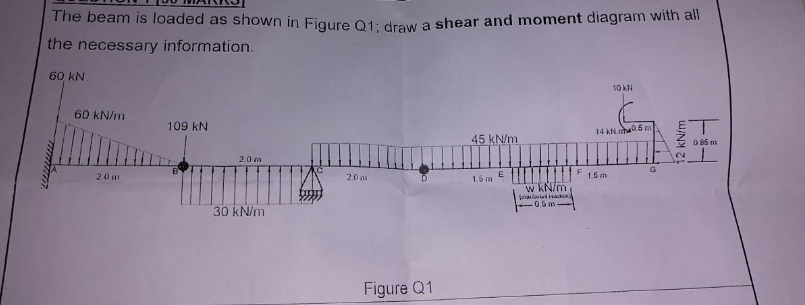
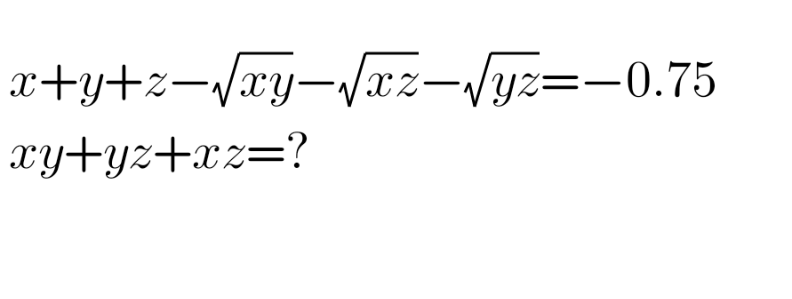
Question Number 143268 Answers: 2 Comments: 0

lim_(x→∞) (((x+3)/(x−4)))^(−2) =?

$${li}\underset{{x}\rightarrow\infty} {{m}}\left(\frac{{x}+\mathrm{3}}{{x}−\mathrm{4}}\right)^{−\mathrm{2}} =? \\ $$

Question Number 143262 Answers: 1 Comments: 0

developp at fourier serie f(x)=(1/(cosx +2sinx))

$${developp}\:{at}\:{fourier}\:{serie} \\ $$$${f}\left({x}\right)=\frac{\mathrm{1}}{{cosx}\:+\mathrm{2}{sinx}} \\ $$

Question Number 143261 Answers: 1 Comments: 0

find Y_n =∫_0 ^∞ (dx/((x+1)(x+2)....(x+n))) (n>1 integr)

$${find}\:{Y}_{{n}} =\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left({x}+\mathrm{1}\right)\left({x}+\mathrm{2}\right)....\left({x}+{n}\right)} \\ $$$$\left({n}>\mathrm{1}\:{integr}\right) \\ $$

Question Number 143260 Answers: 0 Comments: 1

find ∫ (dx/( (√x)+(√(x+1))+(√(x+2))))

$${find}\:\int\:\frac{{dx}}{\:\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}+\sqrt{{x}+\mathrm{2}}} \\ $$

Question Number 143259 Answers: 1 Comments: 0

solve y^(′′) −y^′ +2=xsin(3x)

$${solve}\:{y}^{''} −{y}^{'} +\mathrm{2}={xsin}\left(\mathrm{3}{x}\right) \\ $$

Question Number 143258 Answers: 1 Comments: 0

calculate lim_(x→1) ∫_x ^x^2 ((sh(xt))/(x+t))dt

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{1}} \int_{{x}} ^{{x}^{\mathrm{2}} } \:\frac{{sh}\left({xt}\right)}{{x}+{t}}{dt} \\ $$

Question Number 143257 Answers: 0 Comments: 0

find ∫∫_([0,1]) e^(−(x^2 +y^2 )) arctan(2(√(x^2 +y^2 )))dxdy

$${find}\:\int\int_{\left[\mathrm{0},\mathrm{1}\right]} {e}^{−\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)} {arctan}\left(\mathrm{2}\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }\right){dxdy} \\ $$

Pg 747 Pg 748 Pg 749 Pg 750 Pg 751 Pg 752 Pg 753 Pg 754 Pg 755 Pg 756

Contact: info@tinkutara.com