Question and Answers Forum

AllQuestion and Answers: Page 751

Question Number 143764 Answers: 1 Comments: 0

can anyone tell me,how can I bring everything in this app to the new phone. And after bringing it to the new phone, I will be able to edit everything again.

$${can}\:{anyone}\:{tell}\:{me},{how}\:{can}\:{I} \\ $$$${bring}\:{everything}\:{in}\:{this}\:{app}\:{to} \\ $$$${the}\:{new}\:{phone}. \\ $$$${And}\:{after}\:{bringing}\:{it}\:{to}\:{the}\:{new} \\ $$$${phone},\:{I}\:{will}\:{be}\:{able}\:{to}\:{edit}\:{everything} \\ $$$${again}. \\ $$

Question Number 143763 Answers: 0 Comments: 0

$$ \: \: \: \: \: \: \: \\ $$$$ \: \: \: \: \: \: \: \: \\ $$$$ \: \: \: \: \: \: \: \: \: \\ $$$$ \: \: \\ $$

Question Number 143758 Answers: 0 Comments: 1

Question Number 143755 Answers: 1 Comments: 0

Study the convergence with respect to α and β the improper integral below; ∫_0 ^∞ (dx/(x^α (lnx)^β ))

$$\mathrm{Study}\:\mathrm{the}\:\mathrm{convergence}\:\mathrm{with}\:\mathrm{respect}\:\mathrm{to} \\ $$$$\alpha\:\mathrm{and}\:\beta\:\mathrm{the}\:\mathrm{improper}\:\mathrm{integral}\:\mathrm{below}; \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{dx}}{\mathrm{x}^{\alpha} \left(\mathrm{lnx}\right)^{\beta} } \\ $$

Question Number 143751 Answers: 1 Comments: 0

Question Number 143740 Answers: 1 Comments: 0

Prove that lim_(n→+∞) 2n−(2n+1)ln(n)+Σ_(p=0) ^n ln(1+p^2 )= ln(e^π −e^(−π) )

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\underset{\mathrm{n}\rightarrow+\infty} {\mathrm{lim}2n}−\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{ln}\left(\mathrm{n}\right)+\underset{\mathrm{p}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\mathrm{ln}\left(\mathrm{1}+\mathrm{p}^{\mathrm{2}} \right)=\:\mathrm{ln}\left({e}^{\pi} −{e}^{−\pi} \right) \\ $$

Question Number 143735 Answers: 1 Comments: 0

.....Calculus..... Ω:= ∫_(−∞) ^( ∞) (dx/(x^( 2) e^(a/x^2 ) )) =? (a > 0 )

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.....{Calculus}..... \\ $$$$\:\:\:\:\:\:\:\:\Omega:=\:\int_{−\infty} ^{\:\infty} \frac{{dx}}{{x}^{\:\mathrm{2}} \:{e}^{\frac{{a}}{{x}^{\mathrm{2}} }} }\:=?\:\:\left({a}\:>\:\mathrm{0}\:\right) \\ $$

Question Number 143731 Answers: 1 Comments: 0

Question Number 143730 Answers: 2 Comments: 0

find lim_(x→0) ((sin(sin(1−cosx))−1+cos(x−sinx))/x^3 )

$$\mathrm{find}\:\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\:\frac{\mathrm{sin}\left(\mathrm{sin}\left(\mathrm{1}−\mathrm{cosx}\right)\right)−\mathrm{1}+\mathrm{cos}\left(\mathrm{x}−\mathrm{sinx}\right)}{\mathrm{x}^{\mathrm{3}} } \\ $$

Question Number 143728 Answers: 1 Comments: 0

∫_1 ^∞ (({x})/x^3 )dx=1−(π^2 /(12))

$$\int_{\mathrm{1}} ^{\infty} \frac{\left\{{x}\right\}}{{x}^{\mathrm{3}} }{dx}=\mathrm{1}−\frac{\pi^{\mathrm{2}} }{\mathrm{12}} \\ $$

Question Number 143726 Answers: 1 Comments: 2

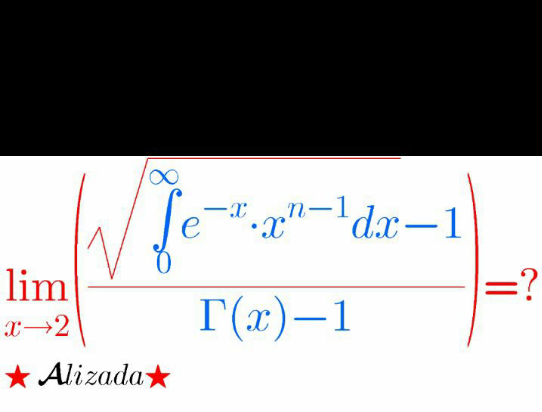
Question Number 143718 Answers: 0 Comments: 5

Question Number 143709 Answers: 1 Comments: 2

Question Number 143708 Answers: 3 Comments: 0

Prove that (3/4^ )+(3^2 /4^2 )+(3^3 /4^3 )+(3^4 /4^4 )+(3^5 /4^5 )+…=3

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\frac{\mathrm{3}}{\mathrm{4}^{} }+\frac{\mathrm{3}^{\mathrm{2}} }{\mathrm{4}^{\mathrm{2}} }+\frac{\mathrm{3}^{\mathrm{3}} }{\mathrm{4}^{\mathrm{3}} }+\frac{\mathrm{3}^{\mathrm{4}} }{\mathrm{4}^{\mathrm{4}} }+\frac{\mathrm{3}^{\mathrm{5}} }{\mathrm{4}^{\mathrm{5}} }+\ldots=\mathrm{3} \\ $$

Question Number 143706 Answers: 1 Comments: 0

2^x +9^y =x^2 +9xy+y^2 Find x,y∈N

$$\mathrm{2}^{\mathrm{x}} +\mathrm{9}^{\mathrm{y}} =\mathrm{x}^{\mathrm{2}} +\mathrm{9xy}+\mathrm{y}^{\mathrm{2}} \\ $$$$\mathrm{Find}\:\mathrm{x},\mathrm{y}\in\mathbb{N} \\ $$

Question Number 143702 Answers: 2 Comments: 0

n ∈ IN. I_n = ∫_1 ^( e) x^(n+1) lnx dx. 1. prove that (I_n ) is positive and increasing. 2. using a part−by−part integration, calculate I_n .

Question Number 143701 Answers: 1 Comments: 1

Question Number 143698 Answers: 0 Comments: 5

My first Contribution to this forum. One year later Q 98831

$$\:{My}\:{first}\:{Contribution}\:{to}\:{this}\:{forum}.\: \\ $$$${One}\:{year}\:{later}\: \\ $$$${Q}\:\mathrm{98831} \\ $$

Question Number 143688 Answers: 0 Comments: 2

Question Number 143684 Answers: 1 Comments: 0

x^3 +x−1=^3 (√(2x^3 +11))+(√(5x^2 +16)) Find x∈R

$$\mathrm{x}^{\mathrm{3}} +\mathrm{x}−\mathrm{1}=^{\mathrm{3}} \sqrt{\mathrm{2x}^{\mathrm{3}} +\mathrm{11}}+\sqrt{\mathrm{5x}^{\mathrm{2}} +\mathrm{16}} \\ $$$$\mathrm{Find}\:\mathrm{x}\in\mathbb{R} \\ $$

Question Number 143680 Answers: 1 Comments: 1

tan 76=4 sin^2 14=?

$$\mathrm{tan}\:\mathrm{76}=\mathrm{4} \\ $$$$\mathrm{sin}\:^{\mathrm{2}} \mathrm{14}=? \\ $$

Question Number 143677 Answers: 3 Comments: 0

Question Number 143671 Answers: 0 Comments: 2

Question Number 143666 Answers: 2 Comments: 1

Question Number 143653 Answers: 1 Comments: 0

Question Number 143650 Answers: 3 Comments: 0

If the function f and g are defined on the set of real numbers,are such that gof(x)=((2x−5)/(3x+7)) and g(x)=((3x+2)/(x−5)). find an expression for f(x)

$${If}\:\:{the}\:{function}\:{f}\:{and}\:{g}\:{are}\:{defined} \\ $$$${on}\:{the}\:{set}\:{of}\:{real}\:{numbers},{are}\:{such} \\ $$$${that}\:\boldsymbol{{gof}}\left(\boldsymbol{{x}}\right)=\frac{\mathrm{2}\boldsymbol{{x}}−\mathrm{5}}{\mathrm{3}\boldsymbol{{x}}+\mathrm{7}}\:\:\:{and}\: \\ $$$$\boldsymbol{{g}}\left(\boldsymbol{{x}}\right)=\frac{\mathrm{3}\boldsymbol{{x}}+\mathrm{2}}{\boldsymbol{{x}}−\mathrm{5}}. \\ $$$$\boldsymbol{\mathrm{find}}\:\:\boldsymbol{\mathrm{an}}\:\boldsymbol{\mathrm{expression}}\:\boldsymbol{\mathrm{for}}\:\:\boldsymbol{\mathrm{f}}\left(\boldsymbol{\mathrm{x}}\right) \\ $$

Pg 746 Pg 747 Pg 748 Pg 749 Pg 750 Pg 751 Pg 752 Pg 753 Pg 754 Pg 755

Contact: info@tinkutara.com