Question and Answers Forum

AllQuestion and Answers: Page 748

Question Number 143006 Answers: 0 Comments: 0

1. y(∂z/∂x) + z(∂z/∂y) = (y/x) 2. x^2 (∂z/∂x) − xy(∂z/∂y) + y^2 = 0 3. { (((∂z/∂x) = (z/x))),(((∂z/∂y) = ((2z)/y))) :}

$$\mathrm{1}.\:{y}\frac{\partial{z}}{\partial{x}}\:+\:{z}\frac{\partial{z}}{\partial{y}}\:=\:\frac{{y}}{{x}} \\ $$$$\mathrm{2}.\:{x}^{\mathrm{2}} \frac{\partial{z}}{\partial{x}}\:−\:{xy}\frac{\partial{z}}{\partial{y}}\:+\:{y}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$$$\mathrm{3}.\:\begin{cases}{\frac{\partial{z}}{\partial{x}}\:=\:\frac{{z}}{{x}}}\\{\frac{\partial{z}}{\partial{y}}\:=\:\frac{\mathrm{2}{z}}{{y}}}\end{cases} \\ $$

Question Number 143003 Answers: 1 Comments: 0

Question Number 142992 Answers: 0 Comments: 3

Question Number 142990 Answers: 2 Comments: 0

find ∫_0 ^∞ (e^(−x^2 ) /((3+x^2 )^2 ))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{x}^{\mathrm{2}} } }{\left(\mathrm{3}+\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 142989 Answers: 2 Comments: 0

calculate ∫_0 ^∞ (e^(−3x^2 ) /(1+x^2 ))dx

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{e}^{−\mathrm{3x}^{\mathrm{2}} } }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 142988 Answers: 0 Comments: 0

find the sequence u_n wich verify u_n +u_(n+1) =(2/( (√n))) give a equivalent of u_n (n→∞)

$$\mathrm{find}\:\mathrm{the}\:\mathrm{sequence}\:\mathrm{u}_{\mathrm{n}} \mathrm{wich}\:\mathrm{verify}\:\mathrm{u}_{\mathrm{n}} +\mathrm{u}_{\mathrm{n}+\mathrm{1}} =\frac{\mathrm{2}}{\:\sqrt{\mathrm{n}}} \\ $$$$\mathrm{give}\:\mathrm{a}\:\mathrm{equivalent}\:\mathrm{of}\:\mathrm{u}_{\mathrm{n}} \:\:\left(\mathrm{n}\rightarrow\infty\right) \\ $$

Question Number 142987 Answers: 1 Comments: 0

find the sequence u_n wich verify u_(n+1) =u_n −λu_(n−1) λ real

$$\mathrm{find}\:\mathrm{the}\:\mathrm{sequence}\:\mathrm{u}_{\mathrm{n}} \mathrm{wich}\:\mathrm{verify}\:\mathrm{u}_{\mathrm{n}+\mathrm{1}} =\mathrm{u}_{\mathrm{n}} −\lambda\mathrm{u}_{\mathrm{n}−\mathrm{1}} \\ $$$$\lambda\:\mathrm{real} \\ $$

Question Number 142986 Answers: 2 Comments: 2

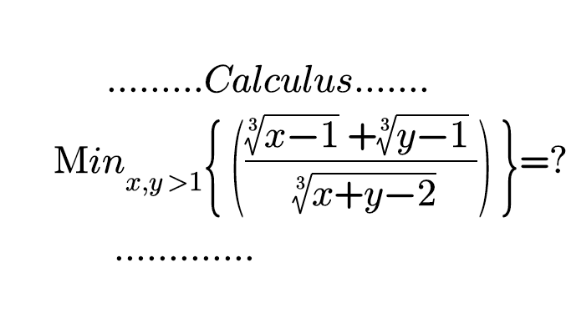
Question Number 142983 Answers: 0 Comments: 0

Question Number 142980 Answers: 0 Comments: 0

find U_n =∫_0 ^∞ e^(−nx^2 ) log(2+e^x )dx (n≥1) determine nature of Σ U_n and Σ nU_n

$$\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\infty} \:\mathrm{e}^{−\mathrm{nx}^{\mathrm{2}} } \mathrm{log}\left(\mathrm{2}+\mathrm{e}^{\mathrm{x}} \right)\mathrm{dx}\:\:\:\left(\mathrm{n}\geqslant\mathrm{1}\right) \\ $$$$\mathrm{determine}\:\mathrm{nature}\:\mathrm{of}\:\Sigma\:\mathrm{U}_{\mathrm{n}} \:\mathrm{and}\:\Sigma\:\mathrm{nU}_{\mathrm{n}} \\ $$

Question Number 142971 Answers: 2 Comments: 1

Question Number 142970 Answers: 1 Comments: 0

..........CALCULUS........... prove that:: 𝛗:=Σ_(n=1) ^∞ (((−1)^(n−1) ((n−1)!)^2 )/((2n)!))=2log^2 (ϕ) ϕ=golden ratio.... .............

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:..........{CALCULUS}........... \\ $$$$\:\:\:\:\:\:\:{prove}\:{that}::\:\: \\ $$$$\:\:\:\:\:\:\boldsymbol{\phi}:=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} \left(\left({n}−\mathrm{1}\right)!\right)^{\mathrm{2}} }{\left(\mathrm{2}{n}\right)!}=\mathrm{2}{log}^{\mathrm{2}} \left(\varphi\right) \\ $$$$\:\:\:\:\varphi={golden}\:{ratio}.... \\ $$$$\:\:\:\:............. \\ $$

Question Number 142968 Answers: 1 Comments: 0

Given p<x<q is solution set inequality 1+2^x +2^(2x) +2^(3x) +...>2 for x≠1. find the value of 5p−3q .

$${Given}\:{p}<{x}<{q}\:{is}\:{solution}\:{set} \\ $$$${inequality}\:\mathrm{1}+\mathrm{2}^{{x}} +\mathrm{2}^{\mathrm{2}{x}} +\mathrm{2}^{\mathrm{3}{x}} +...>\mathrm{2} \\ $$$${for}\:{x}\neq\mathrm{1}.\:{find}\:{the}\:{value}\:{of}\: \\ $$$$\mathrm{5}{p}−\mathrm{3}{q}\:. \\ $$

Question Number 142966 Answers: 0 Comments: 0

Question Number 142977 Answers: 0 Comments: 2

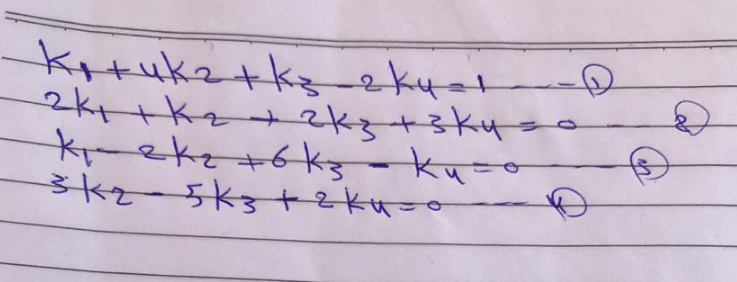
Question Number 142947 Answers: 0 Comments: 5

What is the probability that 3 points will fall twice when a dice is thrown 4 times?

$${What}\:{is}\:{the}\:{probability}\:{that}\:\mathrm{3}\:{points} \\ $$$${will}\:{fall}\:{twice}\:{when}\:{a}\:{dice}\:{is}\:{thrown} \\ $$$$\mathrm{4}\:{times}? \\ $$

Question Number 142945 Answers: 1 Comments: 0

∫_0 ^(0.5) ((1+x^3 ))^(1/3) dx

$$\int_{\mathrm{0}} ^{\mathrm{0}.\mathrm{5}} \sqrt[{\mathrm{3}}]{\mathrm{1}+\boldsymbol{{x}}^{\mathrm{3}} }\boldsymbol{{dx}} \\ $$$$ \\ $$

Question Number 142939 Answers: 2 Comments: 0

Q#141663 by ajfour sir reposted. x^2 (x−12)(x−15)=k(x−16) ;k>0 Find x in terms of k.

$${Q}#\mathrm{141663}\:{by}\:\:{ajfour}\:{sir}\:{reposted}. \\ $$$$\:\:\:\:\:{x}^{\mathrm{2}} \left({x}−\mathrm{12}\right)\left({x}−\mathrm{15}\right)={k}\left({x}−\mathrm{16}\right)\:;{k}>\mathrm{0} \\ $$$$\:\:\:\:\:{Find}\:{x}\:{in}\:{terms}\:{of}\:{k}. \\ $$

Question Number 142936 Answers: 0 Comments: 0

Find all real values of a such that f(x)=((x^2 +ax+1)/(x^2 +x+1)) is surjective f :ℜ⇒ℜ

$${Find}\:{all}\:{real}\:{values}\:{of}\:{a}\:\:\:{such}\:{that}\:\:{f}\left({x}\right)=\frac{{x}^{\mathrm{2}} +{ax}+\mathrm{1}}{{x}^{\mathrm{2}} +{x}+\mathrm{1}}\:\: \\ $$$${is}\:{surjective}\:\:{f}\::\boldsymbol{\Re}\Rightarrow\Re \\ $$

Question Number 142935 Answers: 2 Comments: 0

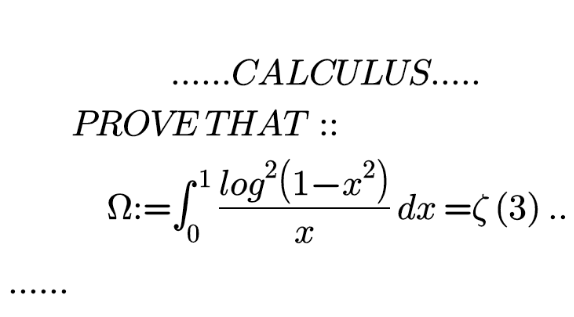
Question Number 142931 Answers: 0 Comments: 0

Question Number 142922 Answers: 2 Comments: 0

lim_(x→1) ((sin(x+1))/(2x−(√(x^2 +3))))=?

$$\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\frac{\mathrm{sin}\left({x}+\mathrm{1}\right)}{\mathrm{2}{x}−\sqrt{{x}^{\mathrm{2}} +\mathrm{3}}}=? \\ $$

Question Number 142927 Answers: 2 Comments: 0

Question Number 142920 Answers: 1 Comments: 2

A student did not notice that the multiplication sign between two 7−digits numbers amd wrote one 14−digits number which turned out to be 3 times the would be product. What are the initial numbers ?

$$\mathrm{A}\:\mathrm{student}\:\mathrm{did}\:\mathrm{not}\:\mathrm{notice}\:\mathrm{that}\:\mathrm{the}\:\mathrm{multiplication} \\ $$$$\mathrm{sign}\:\mathrm{between}\:\mathrm{two}\:\mathrm{7}−\mathrm{digits}\:\mathrm{numbers}\:\mathrm{amd}\:\mathrm{wrote} \\ $$$$\mathrm{one}\:\mathrm{14}−\mathrm{digits}\:\mathrm{number}\:\mathrm{which}\:\mathrm{turned}\:\mathrm{out}\:\mathrm{to}\:\mathrm{be} \\ $$$$\mathrm{3}\:\mathrm{times}\:\mathrm{the}\:\mathrm{would}\:\mathrm{be}\:\mathrm{product}.\:\mathrm{What}\:\mathrm{are}\:\mathrm{the}\:\mathrm{initial} \\ $$$$\mathrm{numbers}\:? \\ $$

Question Number 142917 Answers: 1 Comments: 0

∫(sin^7 (x))dx

$$\int\left(\boldsymbol{\mathrm{sin}}^{\mathrm{7}} \left(\boldsymbol{\mathrm{x}}\right)\right)\boldsymbol{\mathrm{dx}} \\ $$

Question Number 142915 Answers: 0 Comments: 1

Pg 743 Pg 744 Pg 745 Pg 746 Pg 747 Pg 748 Pg 749 Pg 750 Pg 751 Pg 752

Contact: info@tinkutara.com