Question and Answers Forum

AllQuestion and Answers: Page 734

Question Number 144220 Answers: 1 Comments: 0

find A_n =∫_0 ^1 arctan(x^n )dx n ∈N

$${find}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\left({x}^{{n}} \right){dx} \\ $$$${n}\:\in{N} \\ $$

Question Number 144219 Answers: 1 Comments: 0

let f(x)=(1/((2+cosx)^2 )) developp f at fourier serie

$${let}\:{f}\left({x}\right)=\frac{\mathrm{1}}{\left(\mathrm{2}+{cosx}\right)^{\mathrm{2}} } \\ $$$${developp}\:{f}\:{at}\:{fourier}\:{serie} \\ $$

Question Number 144218 Answers: 1 Comments: 0

U_n =Σ_(k=0) ^n (1/( (√(2k+1)))) find a eqivalent of U_n (n→∞)

$${U}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}} \frac{\mathrm{1}}{\:\sqrt{\mathrm{2}{k}+\mathrm{1}}} \\ $$$${find}\:{a}\:{eqivalent}\:{of}\:{U}_{{n}} \left({n}\rightarrow\infty\right) \\ $$

Question Number 144217 Answers: 0 Comments: 0

find ∫_0 ^∞ (x^2 /((x+2)^5 (3x+1)^4 ))dx

$${find}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{x}^{\mathrm{2}} }{\left({x}+\mathrm{2}\right)^{\mathrm{5}} \left(\mathrm{3}{x}+\mathrm{1}\right)^{\mathrm{4}} }{dx} \\ $$

Question Number 144216 Answers: 1 Comments: 0

find ∫ (dx/( (√(x^2 +x+2))+(√(x^2 −x+2))))

$${find}\:\int\:\:\:\frac{{dx}}{\:\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{2}}+\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{2}}} \\ $$

Question Number 144215 Answers: 0 Comments: 0

find ∫_0 ^∞ e^(−3x) (√(x^2 +x+1))dx

$${find}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−\mathrm{3}{x}} \sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$

Question Number 144214 Answers: 1 Comments: 0

calculate ∫_0 ^(4π) ((sinx)/((3+cosx)^2 ))dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{4}\pi} \:\:\frac{{sinx}}{\left(\mathrm{3}+{cosx}\right)^{\mathrm{2}} }{dx} \\ $$

Question Number 144213 Answers: 1 Comments: 0

find ∫ (dx/(1+cosx+cos(2x)))

$${find}\:\int\:\:\frac{{dx}}{\mathrm{1}+{cosx}+{cos}\left(\mathrm{2}{x}\right)} \\ $$

Question Number 144212 Answers: 0 Comments: 0

calculate Σ_(n=0) ^∞ (1/(n^3 +1))

$${calculate}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{3}} +\mathrm{1}} \\ $$

Question Number 144211 Answers: 0 Comments: 0

find Σ_(n=0) ^∞ (1/(n^4 +1))

$${find}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{{n}^{\mathrm{4}} +\mathrm{1}} \\ $$

Question Number 144210 Answers: 1 Comments: 0

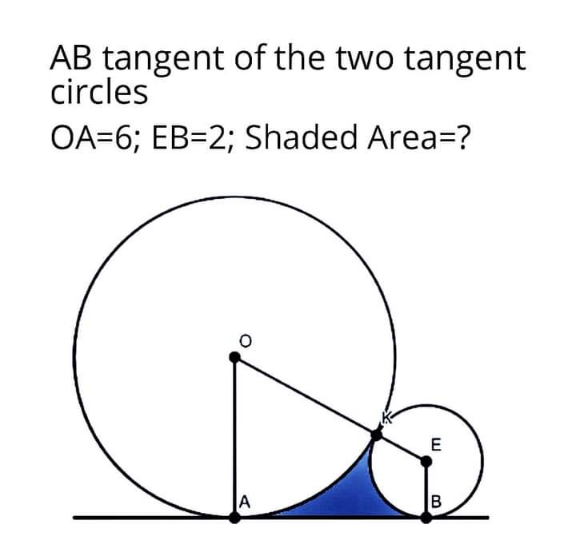
Question Number 144209 Answers: 1 Comments: 0

Question Number 144204 Answers: 1 Comments: 0

Find lim_(h→0) ((f(2h+2+h^2 )−f(2))/(f(h−h^2 +1)−f(1)))=? if given that { ((f ′(2)=6)),((f ′(1)=4)) :}

$$\mathrm{Find}\:\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{f}\left(\mathrm{2h}+\mathrm{2}+\mathrm{h}^{\mathrm{2}} \right)−\mathrm{f}\left(\mathrm{2}\right)}{\mathrm{f}\left(\mathrm{h}−\mathrm{h}^{\mathrm{2}} +\mathrm{1}\right)−\mathrm{f}\left(\mathrm{1}\right)}=? \\ $$$$\mathrm{if}\:\mathrm{given}\:\mathrm{that}\:\begin{cases}{\mathrm{f}\:'\left(\mathrm{2}\right)=\mathrm{6}}\\{\mathrm{f}\:'\left(\mathrm{1}\right)=\mathrm{4}}\end{cases} \\ $$

Question Number 144201 Answers: 1 Comments: 0

Find the equations of the circles passing through (−4,3) and touching the lines x+y=2 and x−y=2

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equations}\:\mathrm{of}\:\mathrm{the}\:\mathrm{circles} \\ $$$$\mathrm{passing}\:\mathrm{through}\:\left(−\mathrm{4},\mathrm{3}\right)\:\mathrm{and}\:\mathrm{touching} \\ $$$$\mathrm{the}\:\mathrm{lines}\:\mathrm{x}+\mathrm{y}=\mathrm{2}\:\mathrm{and}\:\mathrm{x}−\mathrm{y}=\mathrm{2} \\ $$

Question Number 144200 Answers: 1 Comments: 0

Find, among all right circular cylinders of fixed volume V that one with smallest surface area (counting the areas of the faces at top and bottom )

$$\mathrm{Find},\:\mathrm{among}\:\mathrm{all}\:\mathrm{right}\:\mathrm{circular} \\ $$$$\mathrm{cylinders}\:\mathrm{of}\:\mathrm{fixed}\:\mathrm{volume}\:\mathrm{V}\: \\ $$$$\mathrm{that}\:\mathrm{one}\:\mathrm{with}\:\mathrm{smallest}\:\mathrm{surface}\:\mathrm{area} \\ $$$$\left(\mathrm{counting}\:\mathrm{the}\:\mathrm{areas}\:\mathrm{of}\:\mathrm{the}\:\mathrm{faces}\:\right. \\ $$$$\left.\mathrm{at}\:\mathrm{top}\:\mathrm{and}\:\mathrm{bottom}\:\right) \\ $$

Question Number 144196 Answers: 0 Comments: 0

Let a,b,c>0 and a+b+c=3.Prove that (1+a^2 )(1+b^2 )(1+c^2 )≤(1+(1/( ((abc))^(1/3) )))^3

$$\mathrm{Let}\:\mathrm{a},\mathrm{b},\mathrm{c}>\mathrm{0}\:\mathrm{and}\:\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{3}.\mathrm{Prove}\:\mathrm{that} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{1}+\mathrm{a}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{b}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{c}^{\mathrm{2}} \right)\leqslant\left(\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt[{\mathrm{3}}]{\mathrm{abc}}}\right)^{\mathrm{3}} \\ $$

Question Number 144190 Answers: 1 Comments: 0

Σ_(n=1) ^∞ ((5n)/(n^2 + 3)) = ?

$$\underset{\boldsymbol{{n}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{5}{n}}{{n}^{\mathrm{2}} \:+\:\mathrm{3}}\:=\:? \\ $$

Question Number 144186 Answers: 2 Comments: 0

Estimate ∫_0 ^(0.5) (√(1+x^4 )) dx with an error 0.0001

$${Estimate}\:\int_{\mathrm{0}} ^{\mathrm{0}.\mathrm{5}} \sqrt{\mathrm{1}+{x}^{\mathrm{4}} }\:{dx} \\ $$$${with}\:{an}\:{error}\:\mathrm{0}.\mathrm{0001} \\ $$

Question Number 144187 Answers: 1 Comments: 0

∫_0 ^(+∞) (u^2 /(u^8 +2u^4 +1))du

$$\int_{\mathrm{0}} ^{+\infty} \frac{{u}^{\mathrm{2}} }{{u}^{\mathrm{8}} +\mathrm{2}{u}^{\mathrm{4}} +\mathrm{1}}{du} \\ $$

Question Number 144180 Answers: 2 Comments: 0

Prove that ∫^( +∞) _0 ((sh(𝛂t))/(sh(t)))dt = (𝛑/2)tan(((𝛑𝛂)/2))

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\underset{\mathrm{0}} {\int}^{\:+\infty} \:\frac{\boldsymbol{\mathrm{sh}}\left(\boldsymbol{\alpha\mathrm{t}}\right)}{\boldsymbol{\mathrm{sh}}\left(\boldsymbol{\mathrm{t}}\right)}\boldsymbol{{dt}}\:=\:\frac{\boldsymbol{\pi}}{\mathrm{2}}\boldsymbol{{tan}}\left(\frac{\boldsymbol{\pi\alpha}}{\mathrm{2}}\right) \\ $$

Question Number 144177 Answers: 1 Comments: 0

Question Number 144174 Answers: 1 Comments: 1

∫_0 ^π (sinx)^(2n) dx=....? ∀n∈N

$$\int_{\mathrm{0}} ^{\pi} \left({sinx}\right)^{\mathrm{2}{n}} {dx}=....?\:\:\:\forall{n}\in\mathbb{N} \\ $$

Question Number 144170 Answers: 2 Comments: 0

Question Number 144169 Answers: 1 Comments: 1

please find the value of sin ((2Π)/7)+sin ((4Π)/7)+sin ((8Π)/7)

$$\mathrm{please}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\: \\ $$$$\mathrm{sin}\:\frac{\mathrm{2}\Pi}{\mathrm{7}}+\mathrm{sin}\:\frac{\mathrm{4}\Pi}{\mathrm{7}}+\mathrm{sin}\:\frac{\mathrm{8}\Pi}{\mathrm{7}} \\ $$

Question Number 144168 Answers: 1 Comments: 0

Question Number 144166 Answers: 1 Comments: 0

Given p^→ =(√2) i^ +2(√3) j^ + (√3) k^ & q^→ =a i^ +j^ +2k^ . If proj_q^→ p^→ = ((2(√2))/9) q^→ then ∣q^→ ∣ =?

$$\mathrm{Given}\:\overset{\rightarrow} {\mathrm{p}}=\sqrt{\mathrm{2}}\:\hat {\mathrm{i}}+\mathrm{2}\sqrt{\mathrm{3}}\:\hat {\mathrm{j}}+\:\sqrt{\mathrm{3}}\:\hat {\mathrm{k}}\:\&\: \\ $$$$\:\overset{\rightarrow} {\mathrm{q}}=\mathrm{a}\:\hat {\mathrm{i}}+\hat {\mathrm{j}}\:+\mathrm{2}\hat {\mathrm{k}}\:.\:\mathrm{If}\:\mathrm{proj}_{\overset{\rightarrow} {\mathrm{q}}} \:\overset{\rightarrow} {\mathrm{p}}\:=\:\frac{\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{9}}\:\overset{\rightarrow} {\mathrm{q}}\: \\ $$$$\mathrm{then}\:\mid\overset{\rightarrow} {\mathrm{q}}\mid\:=?\: \\ $$

Pg 729 Pg 730 Pg 731 Pg 732 Pg 733 Pg 734 Pg 735 Pg 736 Pg 737 Pg 738

Contact: info@tinkutara.com