Question and Answers Forum

AllQuestion and Answers: Page 717

Question Number 146156 Answers: 0 Comments: 0

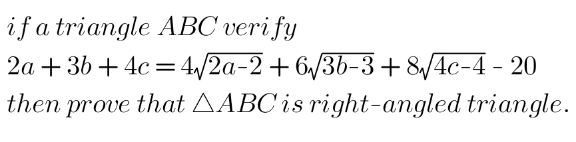
Question Number 146155 Answers: 0 Comments: 2

lim_(n→∞) (Arcsin(x))^( n) =0 ∴ x ∈ ? Q : mr liberty

$$ \\ $$$$\:\:\:\:\:\:{lim}_{{n}\rightarrow\infty} \:\left({Arcsin}\left({x}\right)\right)^{\:{n}} =\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\therefore\:\:\:\:\:\:\:{x}\:\in\:?\: \\ $$$$\:\:\:\:\:\:{Q}\::\:{mr}\:{liberty} \\ $$$$\:\:\:\:\:\:\:\:\:\:\: \\ $$

Question Number 146154 Answers: 0 Comments: 0

Question Number 146146 Answers: 0 Comments: 1

to admin tinku tara. why can't i post in hebrew ?

$$ \\ $$to admin tinku tara. why can't i post in hebrew ?

Question Number 146141 Answers: 0 Comments: 4

An incident ray is reflected normally by a plane mirror onto a screen where it forms a bright spot. The mirror and screen are parallel and 1m apart. If the mirror is rotated through 5°, calculate the displacement of the spot

$$ \\ $$An incident ray is reflected normally by a plane mirror onto a screen where it forms a bright spot. The mirror and screen are parallel and 1m apart. If the mirror is rotated through 5°, calculate the displacement of the spot

Question Number 146150 Answers: 0 Comments: 0

(Level - 2) 10th maths assignment of polynomials by PP sir Defind upwards and downwards parabolas.

$$\:\:\:\left(\boldsymbol{\mathrm{L}}\mathrm{evel}\:-\:\mathrm{2}\right)\:\:\:\:\:\mathrm{10}\boldsymbol{\mathrm{th}}\:\boldsymbol{\mathrm{maths}}\:\boldsymbol{\mathrm{assignment}}\:\boldsymbol{\mathrm{of}}\:\boldsymbol{\mathrm{polynomials}}\:\boldsymbol{\mathrm{by}}\:\boldsymbol{\mathrm{PP}}\:\boldsymbol{\mathrm{sir}} \\ $$$$\mathrm{Defind}\:\mathrm{upwards}\:\mathrm{and}\:\mathrm{downwards}\:\mathrm{parabolas}. \\ $$$$ \\ $$$$ \\ $$

Question Number 146147 Answers: 2 Comments: 0

Υ = ∫ (dx/(x^4 (√(x^2 −a^2 )))) =?

$$\:\Upsilon\:=\:\int\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} \:\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} }}\:=? \\ $$

Question Number 146119 Answers: 3 Comments: 2

determinant (((lim_(x→0) ((√(5x^2 +4x^4 ))/(3x)) =?)),((lim_(x→0) (x^3 /( (√(x^6 +3x^7 )))) =?)))

$$\:\:\:\:\:\:\:\:\:\begin{array}{|c|c|}{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{5}{x}^{\mathrm{2}} +\mathrm{4}{x}^{\mathrm{4}} }}{\mathrm{3}{x}}\:=?}\\{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{3}} }{\:\sqrt{{x}^{\mathrm{6}} +\mathrm{3}{x}^{\mathrm{7}} }}\:=?}\\\hline\end{array} \\ $$

Question Number 146110 Answers: 2 Comments: 0

∫((x+1)/(2x^2 +x+1))dx

$$\int\frac{\mathrm{x}+\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}}\mathrm{dx} \\ $$

Question Number 146108 Answers: 0 Comments: 0

Solve in Z[X] 1) XP ′ ≡ −1 mod(X^4 +1) 2) X^3 P −P ′ ≡ 1−X^2 mod(X^4 +1) 3) P^2 −X^3 P−X^2 ≡ 0 mod(X^2 +2)

$$\:{Solve}\:\:{in}\:\mathbb{Z}\left[{X}\right] \\ $$$$\left.\mathrm{1}\right)\:{XP}\:'\:\equiv\:−\mathrm{1}\:{mod}\left({X}^{\mathrm{4}} +\mathrm{1}\right) \\ $$$$\left.\mathrm{2}\right)\:{X}^{\mathrm{3}} {P}\:−{P}\:'\:\equiv\:\mathrm{1}−{X}^{\mathrm{2}} \:{mod}\left({X}^{\mathrm{4}} +\mathrm{1}\right) \\ $$$$\left.\mathrm{3}\right)\:{P}\:^{\mathrm{2}} −{X}^{\mathrm{3}} {P}−{X}^{\mathrm{2}} \:\:\equiv\:\mathrm{0}\:{mod}\left({X}^{\mathrm{2}} +\mathrm{2}\right) \\ $$

Question Number 151714 Answers: 0 Comments: 2

Question Number 146131 Answers: 1 Comments: 1

Question Number 146106 Answers: 2 Comments: 0

Question Number 146102 Answers: 1 Comments: 0

prove by mathmatical indiction 5+7+9+.....+(4n+1)=2n^2 +3n

$${prove}\:{by}\:{mathmatical}\:{indiction}\: \\ $$$$\mathrm{5}+\mathrm{7}+\mathrm{9}+.....+\left(\mathrm{4}{n}+\mathrm{1}\right)=\mathrm{2}{n}^{\mathrm{2}} +\mathrm{3}{n} \\ $$

Question Number 146100 Answers: 1 Comments: 1

Question Number 146096 Answers: 1 Comments: 0

Question Number 146090 Answers: 2 Comments: 0

∫_0 ^∞ ((sinh(at)sinh(bt))/(sinh(ct)e^(tz) ))dt= ((ab)/(c(z^2 +c^2 −a^2 −b^2 +K_(k=1) ^∞ ((−4k^2 (k^2 c^2 −a^2 )(k^2 c^2 −b^2 ))/((2k+1)(z^2 +(2k^2 +2k+1)c^2 −a^2 −b^2 ))))))

$$\int_{\mathrm{0}} ^{\infty} \frac{\mathrm{sinh}\left(\mathrm{at}\right)\mathrm{sinh}\left(\mathrm{bt}\right)}{\mathrm{sinh}\left(\mathrm{ct}\right)\mathrm{e}^{\mathrm{tz}} }\mathrm{dt}= \\ $$$$\frac{\mathrm{ab}}{\mathrm{c}\left(\mathrm{z}^{\mathrm{2}} +\mathrm{c}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} −\mathrm{b}^{\mathrm{2}} +\underset{\mathrm{k}=\mathrm{1}} {\overset{\infty} {\mathrm{K}}}\frac{−\mathrm{4k}^{\mathrm{2}} \left(\mathrm{k}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} \right)\left(\mathrm{k}^{\mathrm{2}} \mathrm{c}^{\mathrm{2}} −\mathrm{b}^{\mathrm{2}} \right)}{\left(\mathrm{2k}+\mathrm{1}\right)\left(\mathrm{z}^{\mathrm{2}} +\left(\mathrm{2k}^{\mathrm{2}} +\mathrm{2k}+\mathrm{1}\right)\mathrm{c}^{\mathrm{2}} −\mathrm{a}^{\mathrm{2}} −\mathrm{b}^{\mathrm{2}} \right)}\right)} \\ $$

Question Number 146091 Answers: 0 Comments: 0

Question Number 146087 Answers: 1 Comments: 0

1)find U_n =∫_0 ^1 x^n e^(−2x) dx 2)nature of Σ U_n ?

$$\left.\mathrm{1}\right)\mathrm{find}\:\mathrm{U}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{x}^{\mathrm{n}} \:\mathrm{e}^{−\mathrm{2x}} \:\mathrm{dx} \\ $$$$\left.\mathrm{2}\right)\mathrm{nature}\:\mathrm{of}\:\Sigma\:\mathrm{U}_{\mathrm{n}} ? \\ $$

Question Number 146085 Answers: 0 Comments: 1

f(x,y)=x−(√(x+2y)) 1)condition on x and y to have f symetric 2) find (∂f/∂x) ,(∂f/∂y) ,(∂^2 f/(∂x∂y)) ,(∂^2 f/(∂y∂x)) 3) find (∂^2 f/∂^2 x) and (∂^2 f/∂^2 y)

$$\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)=\mathrm{x}−\sqrt{\mathrm{x}+\mathrm{2y}} \\ $$$$\left.\mathrm{1}\right)\mathrm{condition}\:\mathrm{on}\:\mathrm{x}\:\mathrm{and}\:\mathrm{y}\:\mathrm{to}\:\mathrm{have}\:\mathrm{f}\:\mathrm{symetric} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{find}\:\frac{\partial\mathrm{f}}{\partial\mathrm{x}}\:,\frac{\partial\mathrm{f}}{\partial\mathrm{y}}\:,\frac{\partial^{\mathrm{2}} \mathrm{f}}{\partial\mathrm{x}\partial\mathrm{y}}\:,\frac{\partial^{\mathrm{2}} \mathrm{f}}{\partial\mathrm{y}\partial\mathrm{x}} \\ $$$$\left.\mathrm{3}\right)\:\mathrm{find}\:\frac{\partial^{\mathrm{2}} \mathrm{f}}{\partial^{\mathrm{2}} \mathrm{x}}\:\mathrm{and}\:\frac{\partial^{\mathrm{2}} \mathrm{f}}{\partial^{\mathrm{2}} \mathrm{y}} \\ $$

Question Number 146083 Answers: 1 Comments: 0

F(x)=x^n −e^(inα) 1) roots of F(x)? 2) factorize F(x) inside C[x]

$$\mathrm{F}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{n}} \:−\mathrm{e}^{\mathrm{in}\alpha} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{roots}\:\mathrm{of}\:\mathrm{F}\left(\mathrm{x}\right)? \\ $$$$\left.\mathrm{2}\right)\:\mathrm{factorize}\:\mathrm{F}\left(\mathrm{x}\right)\:\mathrm{inside}\:\mathrm{C}\left[\mathrm{x}\right] \\ $$

Question Number 146082 Answers: 0 Comments: 0

p(x)=(x^2 −x+1)^n −(x^2 +x+1)^n 1) roots of p(x)? 2) factorize p(x) inside C[x]

$$\mathrm{p}\left(\mathrm{x}\right)=\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{n}} −\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)^{\mathrm{n}} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{roots}\:\mathrm{of}\:\mathrm{p}\left(\mathrm{x}\right)? \\ $$$$\left.\mathrm{2}\right)\:\mathrm{factorize}\:\mathrm{p}\left(\mathrm{x}\right)\:\mathrm{inside}\:\mathrm{C}\left[\mathrm{x}\right] \\ $$

Question Number 146076 Answers: 1 Comments: 0

if f(x)=∫(3x^2 −2)dx and f(2)=9 then f(−2)= ?

$${if}\:{f}\left({x}\right)=\int\left(\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}\right){dx}\:{and}\:{f}\left(\mathrm{2}\right)=\mathrm{9}\:{then} \\ $$$${f}\left(−\mathrm{2}\right)=\:? \\ $$

Question Number 146073 Answers: 0 Comments: 0

Let K be nonempty corps , K^∗ =K−{0_K } Prove that 1) Π_(x∈K^∗ ) x = −1 2)Deduce that p is prime ⇔ (p−1)!≡−1[p]