
AllQuestion and Answers: Page 716
Question Number 146096 Answers: 1 Comments: 0

Question Number 146090 Answers: 2 Comments: 0
Question Number 146091 Answers: 0 Comments: 0

Question Number 146087 Answers: 1 Comments: 0
Question Number 146085 Answers: 0 Comments: 1
Question Number 146083 Answers: 1 Comments: 0
Question Number 146082 Answers: 0 Comments: 0
Question Number 146076 Answers: 1 Comments: 0
Question Number 146073 Answers: 0 Comments: 0
Question Number 146072 Answers: 0 Comments: 0
Question Number 146067 Answers: 1 Comments: 0
Question Number 146063 Answers: 1 Comments: 0
Question Number 146062 Answers: 0 Comments: 1
Question Number 146061 Answers: 0 Comments: 0
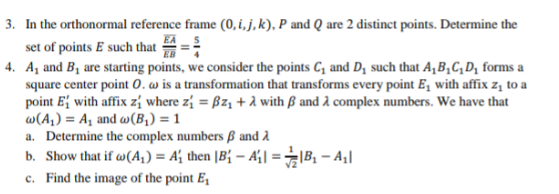
Question Number 146057 Answers: 1 Comments: 0

Question Number 146054 Answers: 0 Comments: 0
Question Number 146048 Answers: 1 Comments: 0
Question Number 146046 Answers: 1 Comments: 0
Question Number 146043 Answers: 2 Comments: 0
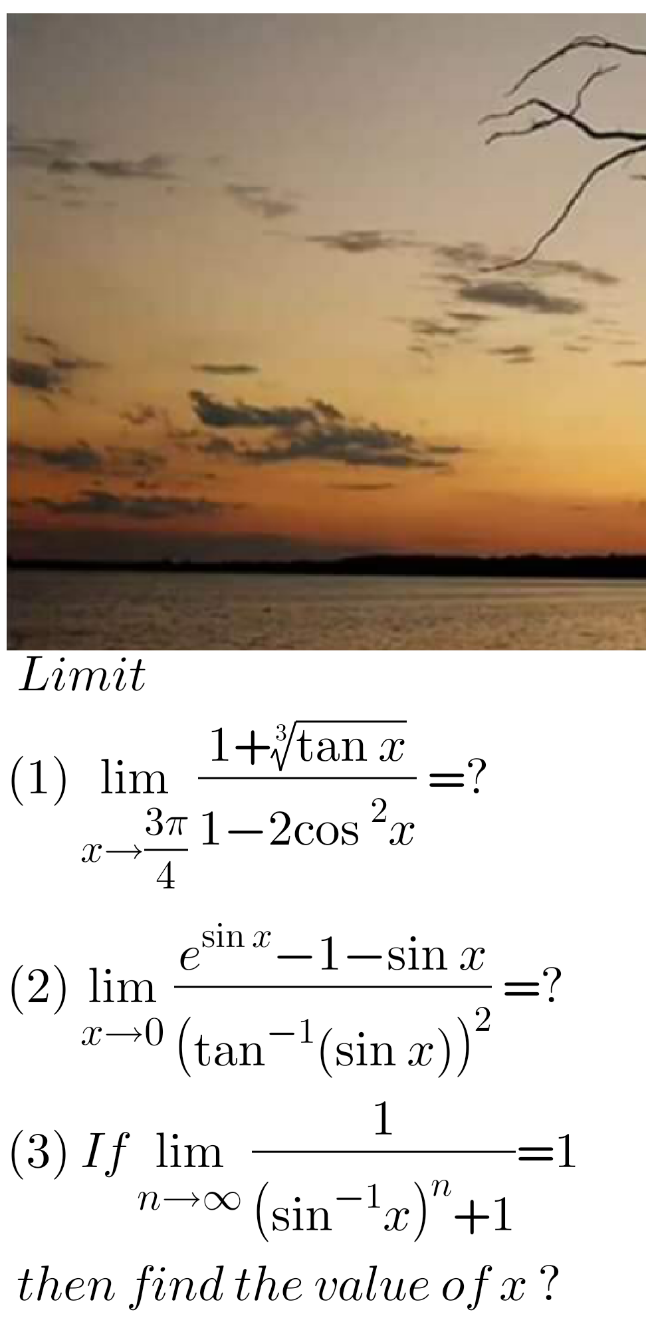
Question Number 146044 Answers: 1 Comments: 0
Question Number 146035 Answers: 2 Comments: 0
$${help}\:{me}\:{please} \\ $$$$\int\frac{{ln}\left({x}+\mathrm{1}\right)}{{x}}{dx}=?? \\ $$$$ \\ $$
Question Number 146030 Answers: 0 Comments: 1

Question Number 146026 Answers: 2 Comments: 3
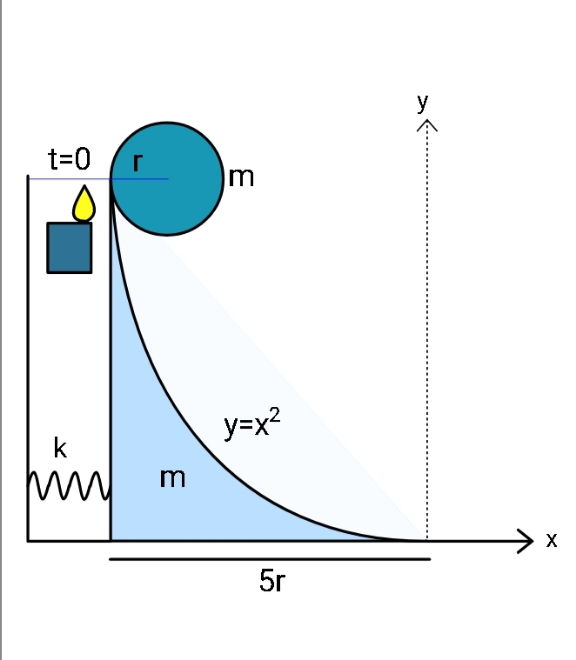
Question Number 146017 Answers: 1 Comments: 0

Question Number 146013 Answers: 0 Comments: 0

Question Number 146009 Answers: 1 Comments: 0
Pg 711 Pg 712 Pg 713 Pg 714 Pg 715 Pg 716 Pg 717 Pg 718 Pg 719 Pg 720
