Question and Answers Forum

AllQuestion and Answers: Page 715

Question Number 141191 Answers: 1 Comments: 0

find the volume of the solid generated when the region bounded by the y=x y=x+2, x=2 and x=4 revolved about the x-axis

$${find}\:{the}\:{volume}\:{of}\:{the}\:{solid}\:{generated} \\ $$$${when}\:{the}\:{region}\:{bounded}\:{by}\:{the}\:{y}={x} \\ $$$${y}={x}+\mathrm{2},\:{x}=\mathrm{2}\:{and}\:{x}=\mathrm{4}\:{revolved}\:{about}\:{the}\:{x}-{axis} \\ $$

Question Number 141189 Answers: 0 Comments: 3

find the area of the shaded region shown below which is boinded by to functions f(x)=x^2 g(x)=2−x and the x-axis

$${find}\:{the}\:{area}\:{of}\:{the}\:{shaded}\:{region} \\ $$$${shown}\:{below}\:{which}\:{is}\:{boinded}\:{by}\:{to}\:{functions}\: \\ $$$${f}\left({x}\right)={x}^{\mathrm{2}} \:\:{g}\left({x}\right)=\mathrm{2}−{x}\:{and}\:{the}\:{x}-{axis} \\ $$$$ \\ $$

Question Number 141186 Answers: 1 Comments: 4

Question Number 141171 Answers: 1 Comments: 6

Question Number 141167 Answers: 0 Comments: 0

Question Number 141164 Answers: 2 Comments: 1

Find maximum value of the product xy(72−3x−4y) for positive value of x & y.

$$\:\:\:\:{Find}\:{maximum}\:{value}\: \\ $$$$\:\:\:{of}\:{the}\:{product}\:{xy}\left(\mathrm{72}−\mathrm{3}{x}−\mathrm{4}{y}\right) \\ $$$$\:\:\:\:{for}\:{positive}\:{value}\:{of}\:{x}\:\&\:{y}. \\ $$

Question Number 141163 Answers: 1 Comments: 0

Question Number 141153 Answers: 2 Comments: 0

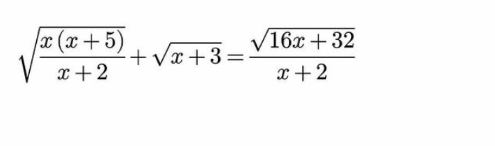
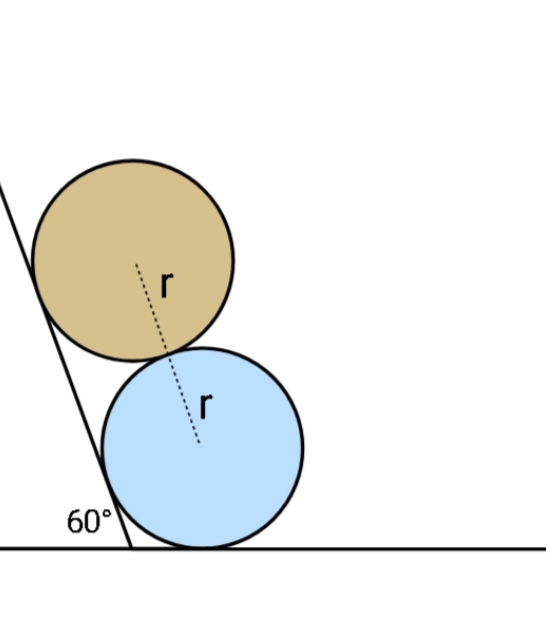
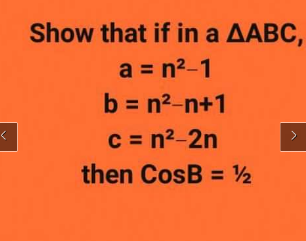
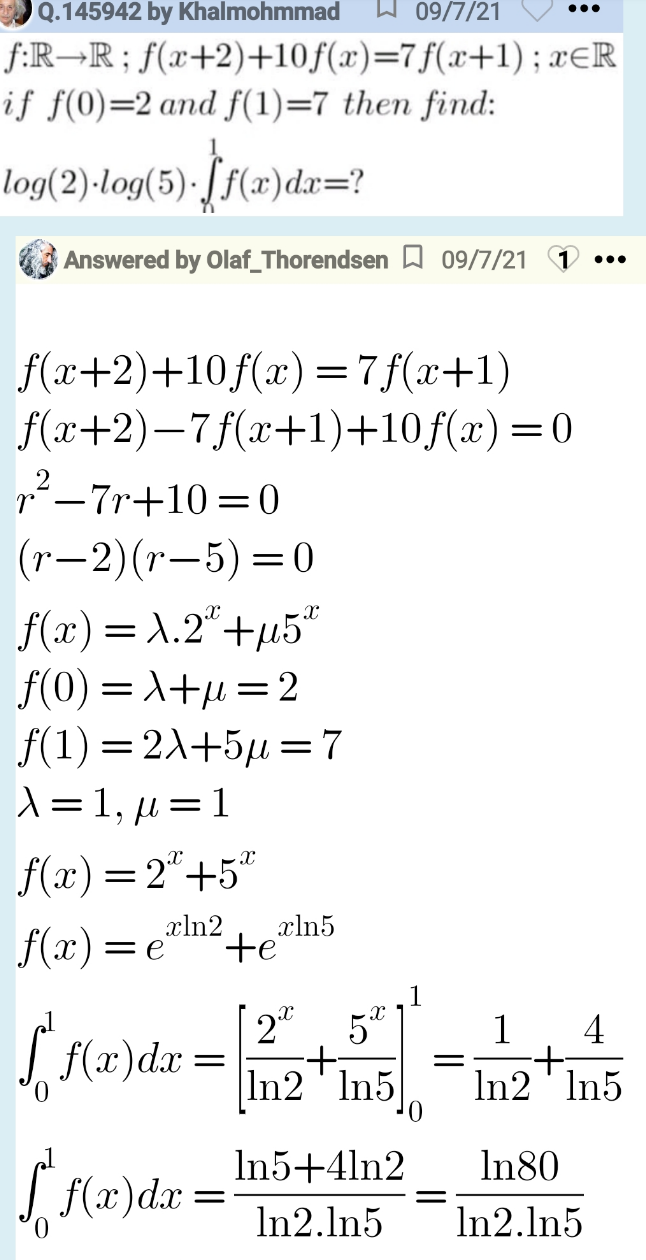
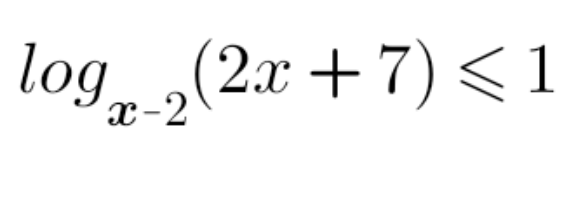
Question Number 141152 Answers: 2 Comments: 0

((3,5),(2,4) ) ((x),(y) )= (((x′)),((y′)) ) and ((a,b),(c,d) ) (((x′)),((y′)) )= ((x),(y) ) are true for any values of x,y,x′,y′, find the values of a,b,c,d.

$$\begin{pmatrix}{\mathrm{3}}&{\mathrm{5}}\\{\mathrm{2}}&{\mathrm{4}}\end{pmatrix}\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}=\begin{pmatrix}{{x}'}\\{{y}'}\end{pmatrix}\:\mathrm{and}\:\begin{pmatrix}{{a}}&{{b}}\\{{c}}&{{d}}\end{pmatrix}\begin{pmatrix}{{x}'}\\{{y}'}\end{pmatrix}=\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix} \\ $$$$\mathrm{are}\:\mathrm{true}\:\mathrm{for}\:\mathrm{any}\:\mathrm{values}\:\mathrm{of}\:{x},{y},{x}',{y}', \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{values}\:\mathrm{of}\:{a},{b},{c},{d}. \\ $$

Question Number 141149 Answers: 3 Comments: 0

lim_(x→∞) Σ_(i=0) ^(x−1) (x/((x+i)))

$${lim}_{{x}\rightarrow\infty} \underset{{i}=\mathrm{0}} {\overset{{x}−\mathrm{1}} {\sum}}\frac{{x}}{\left({x}+{i}\right)} \\ $$

Question Number 141148 Answers: 0 Comments: 0

$$ \\ $$

Question Number 141150 Answers: 0 Comments: 0

Determine the concentration of[OH^− ] in 0.4M NH_4 OH solution having 5.35g NH_4 Cl in a one(1) litre solution. (k_b =1.8×10^(−5) ,N=14,H=1,Cl=35.5)

$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{concentration}\:\mathrm{of}\left[\mathrm{OH}^{−} \right]\: \\ $$$$\mathrm{in}\:\mathrm{0}.\mathrm{4M}\:\mathrm{NH}_{\mathrm{4}} \mathrm{OH}\:\mathrm{solution}\:\mathrm{having}\: \\ $$$$\mathrm{5}.\mathrm{35g}\:\mathrm{NH}_{\mathrm{4}} \mathrm{Cl}\:\mathrm{in}\:\mathrm{a}\:\mathrm{one}\left(\mathrm{1}\right)\:\mathrm{litre}\:\mathrm{solution}. \\ $$$$\left(\mathrm{k}_{\mathrm{b}} =\mathrm{1}.\mathrm{8}×\mathrm{10}^{−\mathrm{5}} ,\mathrm{N}=\mathrm{14},\mathrm{H}=\mathrm{1},\mathrm{Cl}=\mathrm{35}.\mathrm{5}\right) \\ $$$$ \\ $$

Question Number 141142 Answers: 0 Comments: 0

Question Number 141136 Answers: 2 Comments: 0

Find the smallest value 5x + ((16)/x) + 21 over positive value of x

$$\:\:\:\:{Find}\:{the}\:{smallest}\:{value}\: \\ $$$$\:\:\:\:\mathrm{5}{x}\:+\:\frac{\mathrm{16}}{{x}}\:+\:\mathrm{21}\:{over}\:{positive}\: \\ $$$$\:\:\:{value}\:{of}\:{x}\: \\ $$

Question Number 141135 Answers: 3 Comments: 0

Find the minimum of ((12)/x) + ((18)/y) + xy for all positive number x & y .

$$\:\:\:\:\:\:\:{Find}\:{the}\:{minimum}\:{of}\: \\ $$$$\:\:\:\:\:\:\frac{\mathrm{12}}{{x}}\:+\:\frac{\mathrm{18}}{{y}}\:+\:{xy}\:{for}\:{all}\: \\ $$$$\:\:\:\:\:\:{positive}\:{number}\:{x}\:\&\:{y}\:. \\ $$

Question Number 141134 Answers: 1 Comments: 0

prove that:: Φ:=Σ_(m,n=1) ^∞ (((−1)^(n−1) )/(m^2 +n^2 ))= (π^2 /(24))+((πln(2))/8)

$$\:\:\:\:\: \\ $$$$\:\:\:{prove}\:{that}:: \\ $$$$\:\:\Phi:=\underset{{m},{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{m}^{\mathrm{2}} +{n}^{\mathrm{2}} }=\:\frac{\pi^{\mathrm{2}} }{\mathrm{24}}+\frac{\pi{ln}\left(\mathrm{2}\right)}{\mathrm{8}} \\ $$$$ \\ $$

Question Number 141133 Answers: 1 Comments: 0

I:=∫_0 ^( ∞) (((x^n −1)(x−1))/(x^(n+3) −1))dx=??

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\boldsymbol{\mathrm{I}}:=\int_{\mathrm{0}} ^{\:\infty} \frac{\left({x}^{{n}} −\mathrm{1}\right)\left({x}−\mathrm{1}\right)}{{x}^{{n}+\mathrm{3}} −\mathrm{1}}{dx}=?? \\ $$$$ \\ $$

Question Number 141132 Answers: 1 Comments: 0

...mathematical ...analysis... prove that: Π_(n=0) ^∞ (1+(1/2^2^n ) ) =^? 2 ......

$$\:\:\: \\ $$$$\:...{mathematical}\:...{analysis}... \\ $$$$\:\:\:\:{prove}\:\:{that}: \\ $$$$\:\:\:\:\:\:\:\:\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\prod}}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}^{{n}} } }\:\right)\:\overset{?} {=}\:\mathrm{2}\:\:\: \\ $$$$\:\:\:\:\:\:...... \\ $$

Question Number 141129 Answers: 1 Comments: 0

For what values of λ are the vectors λi^ + 2j^ + k^ , 3i^ +4j^ +λk^ , j^ + k^ coplanar

$${For}\:{what}\:{values}\:{of}\:\lambda\:{are}\:{the} \\ $$$${vectors}\:\lambda\hat {{i}}\:+\:\mathrm{2}\hat {{j}}\:+\:\hat {{k}}\:,\:\mathrm{3}\hat {{i}}\:+\mathrm{4}\hat {{j}}\:+\lambda\hat {{k}}\: \\ $$$$,\:\hat {{j}}\:+\:\hat {{k}}\:\:{coplanar}\: \\ $$

Question Number 141127 Answers: 1 Comments: 0

lim_(n→∞) Σ_(i=0) ^(n−1) (n/((n+i)^2 )) =?

$$\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}\:\underset{{i}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\:\frac{{n}}{\left({n}+{i}\right)^{\mathrm{2}} }\:=?\: \\ $$

Question Number 141124 Answers: 2 Comments: 0

Question Number 141122 Answers: 0 Comments: 4

M=<a;a+1;a+2;...;a+n> N=<a;a^2 ;a^3 ;...;a^n > be an ideals in Q[a] ; where n∈2Z M/N=?

$${M}=<{a};{a}+\mathrm{1};{a}+\mathrm{2};...;{a}+{n}> \\ $$$${N}=<{a};{a}^{\mathrm{2}} ;{a}^{\mathrm{3}} ;...;{a}^{{n}} > \\ $$$${be}\:{an}\:{ideals}\:{in}\:{Q}\left[{a}\right]\:;\:{where}\:\:{n}\in\mathrm{2}\mathbb{Z} \\ $$$${M}/{N}=? \\ $$

Question Number 141111 Answers: 1 Comments: 1

A gap must be left between steel rails to allow for thermal expansion. How large a gap is needed if the maximum temperature reached is 50° above the temperature at which the rails were laid. The length of the rail is 10m and the α_(steal) =12×10^(−6) /°c

$$\mathrm{A}\:\mathrm{gap}\:\mathrm{must}\:\mathrm{be}\:\mathrm{left}\:\mathrm{between}\:\mathrm{steel}\: \\ $$$$\mathrm{rails}\:\mathrm{to}\:\mathrm{allow}\:\mathrm{for}\:\mathrm{thermal}\:\mathrm{expansion}. \\ $$$$\:\mathrm{How}\:\mathrm{large}\:\mathrm{a}\:\mathrm{gap}\:\mathrm{is}\:\mathrm{needed}\:\mathrm{if}\:\mathrm{the}\: \\ $$$$\mathrm{maximum}\:\mathrm{temperature}\:\mathrm{reached} \\ $$$$\:\mathrm{is}\:\mathrm{50}°\:\mathrm{above}\:\mathrm{the}\:\mathrm{temperature}\:\mathrm{at}\: \\ $$$$\mathrm{which}\:\mathrm{the}\:\mathrm{rails}\:\mathrm{were}\:\mathrm{laid}.\:\mathrm{The}\: \\ $$$$\mathrm{length}\:\mathrm{of}\:\mathrm{the}\:\mathrm{rail}\:\mathrm{is}\:\mathrm{10m}\:\mathrm{and} \\ $$$$\:\mathrm{the}\:\alpha_{\mathrm{steal}} =\mathrm{12}×\mathrm{10}^{−\mathrm{6}} /°\mathrm{c} \\ $$

Question Number 141110 Answers: 1 Comments: 0

sin^2 x+sinx=(4/3) , x∈[0,π] equation sum of roots in range?

$${sin}^{\mathrm{2}} {x}+{sinx}=\frac{\mathrm{4}}{\mathrm{3}}\:\:\:\:\:\:\:,\:\:\:{x}\in\left[\mathrm{0},\pi\right] \\ $$$${equation}\:\:{sum}\:\:{of}\:{roots}\:{in}\:{range}? \\ $$

Question Number 141107 Answers: 0 Comments: 0

Question Number 141104 Answers: 1 Comments: 0

Pg 710 Pg 711 Pg 712 Pg 713 Pg 714 Pg 715 Pg 716 Pg 717 Pg 718 Pg 719

Contact: info@tinkutara.com