Question and Answers Forum

AllQuestion and Answers: Page 711

Question Number 147746 Answers: 1 Comments: 0

Question Number 147738 Answers: 2 Comments: 0

lim_(x→0) ((arctan x−arcsin x)/(sin^3 x)) =?

$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{arctan}\:{x}−\mathrm{arcsin}\:{x}}{\mathrm{sin}\:^{\mathrm{3}} {x}}\:=? \\ $$

Question Number 147737 Answers: 1 Comments: 0

Σ_(n≥1) (1/n^2 )(1+(1/2)+(1/3)+...+(1/n))^2 =?

$$\:\underset{{n}\geqslant\mathrm{1}} {\sum}\:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{3}}+...+\frac{\mathrm{1}}{{n}}\right)^{\mathrm{2}} =? \\ $$

Question Number 147714 Answers: 1 Comments: 0

Resoudre log_a (x^2 ) > log_a^2 (3x−2) avec a∈R_+ \{1} NB: a et a^2 sont les bases des logarithmes des nombres de l′ine^ galite.. les resultats se donnerons soua forme d′ine^ galite selon les cas..

$$\mathrm{Resoudre} \\ $$$$\mathrm{log}_{\mathrm{a}} \left(\mathrm{x}^{\mathrm{2}} \right)\:>\:\mathrm{log}_{\mathrm{a}^{\mathrm{2}} } \left(\mathrm{3x}−\mathrm{2}\right) \\ $$$$\mathrm{avec}\:\:\mathrm{a}\in\mathbb{R}_{+} \backslash\left\{\mathrm{1}\right\} \\ $$$$\mathrm{NB}:\:\mathrm{a}\:\mathrm{et}\:\mathrm{a}^{\mathrm{2}} \:\mathrm{sont}\:\mathrm{les}\:\mathrm{bases}\:\mathrm{des}\:\mathrm{logarithmes} \\ $$$$\mathrm{des}\:\mathrm{nombres}\:\mathrm{de}\:\mathrm{l}'\mathrm{in}\acute {\mathrm{e}galite}.. \\ $$$$\mathrm{les}\:\mathrm{resultats}\:\mathrm{se}\:\mathrm{donnerons}\:\mathrm{soua}\:\mathrm{forme} \\ $$$$\mathrm{d}'\mathrm{in}\acute {\mathrm{e}galite}\:\mathrm{selon}\:\mathrm{les}\:\mathrm{cas}.. \\ $$

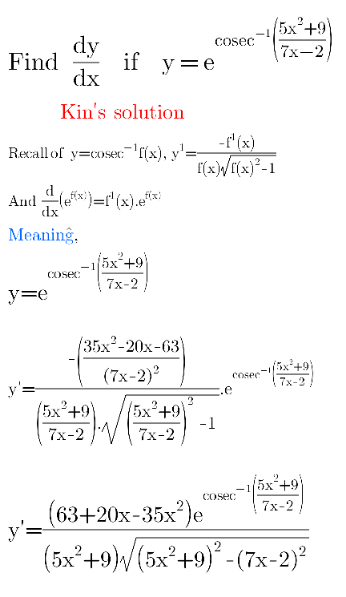
Question Number 147713 Answers: 1 Comments: 0

Find a point on the curve y=(√x) where the tangent makes an angle 45° with the positive x-axis

$${Find}\:{a}\:{point}\:{on}\:{the}\:{curve}\:{y}=\sqrt{{x}} \\ $$$${where}\:{the}\:{tangent}\:{makes}\:{an}\:{angle}\: \\ $$$$\mathrm{45}°\:{with}\:{the}\:{positive}\:{x}-{axis} \\ $$

Question Number 147711 Answers: 1 Comments: 0

Determiner l′original de laplace F(x)=(1/((x^2 +x+1)^2 ))

$${Determiner}\:{l}'{original}\:{de}\:{laplace} \\ $$$${F}\left({x}\right)=\frac{\mathrm{1}}{\left({x}^{\mathrm{2}} +{x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 147706 Answers: 1 Comments: 0

Question Number 147699 Answers: 1 Comments: 0

a^2 = 36^7 + 9^b + 6^8 ; a∈Z find b=?

$$\boldsymbol{{a}}^{\mathrm{2}} \:=\:\mathrm{36}^{\mathrm{7}} \:+\:\mathrm{9}^{\boldsymbol{{b}}} \:+\:\mathrm{6}^{\mathrm{8}} \:\:;\:\:\boldsymbol{{a}}\in\mathbb{Z} \\ $$$${find}\:\:\boldsymbol{{b}}=? \\ $$

Question Number 147689 Answers: 1 Comments: 0

(234)_5 ∙ (23)_5 = (x)_5 ⇒ x=?

$$\left(\mathrm{234}\right)_{\mathrm{5}} \:\centerdot\:\left(\mathrm{23}\right)_{\mathrm{5}} \:=\:\left({x}\right)_{\mathrm{5}} \:\:\Rightarrow\:\:\boldsymbol{{x}}=? \\ $$

Question Number 147688 Answers: 1 Comments: 0

find lim_(n→+∞) ∫_(1/n) ^(√n) xe^(−x^2 ) arctan(nx)dx

$$\mathrm{find}\:\mathrm{lim}_{\mathrm{n}\rightarrow+\infty} \int_{\frac{\mathrm{1}}{\mathrm{n}}} ^{\sqrt{\mathrm{n}}} \:\:\:\mathrm{xe}^{−\mathrm{x}^{\mathrm{2}} } \mathrm{arctan}\left(\mathrm{nx}\right)\mathrm{dx} \\ $$

Question Number 147687 Answers: 2 Comments: 0

calculate lim_(n→0) ((e^(−nx^2 ) −nx−1)/x^3 )

$$\mathrm{calculate}\:\mathrm{lim}_{\mathrm{n}\rightarrow\mathrm{0}} \:\frac{\mathrm{e}^{−\mathrm{nx}^{\mathrm{2}} } −\mathrm{nx}−\mathrm{1}}{\mathrm{x}^{\mathrm{3}} } \\ $$

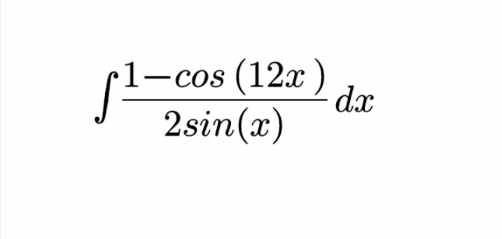
Question Number 147686 Answers: 0 Comments: 0

Question Number 147685 Answers: 2 Comments: 0

calculate lim_(x→0) ((sin(2sinx))/x^2 )

$$\mathrm{calculate}\:\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\frac{\mathrm{sin}\left(\mathrm{2sinx}\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$

Question Number 147684 Answers: 0 Comments: 0

decompse F(x)=(x^3 /((x^2 +1)^4 )) inside C(x)

$$\mathrm{decompse}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{x}^{\mathrm{3}} }{\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{4}} }\:\:\:\mathrm{inside}\:\mathrm{C}\left(\mathrm{x}\right) \\ $$

Question Number 147683 Answers: 1 Comments: 0

let F(x)=(1/((x+1)^5 (2x−3)^4 )) 1) find ∫ F(x)dx 2)en deduire la decomposition de F en element simples

$$\mathrm{let}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}+\mathrm{1}\right)^{\mathrm{5}} \left(\mathrm{2x}−\mathrm{3}\right)^{\mathrm{4}} } \\ $$$$\left.\mathrm{1}\right)\:\mathrm{find}\:\int\:\mathrm{F}\left(\mathrm{x}\right)\mathrm{dx} \\ $$$$\left.\mathrm{2}\right)\mathrm{en}\:\mathrm{deduire}\:\mathrm{la}\:\mathrm{decomposition}\:\mathrm{de}\:\mathrm{F}\:\mathrm{en}\:\mathrm{element}\:\mathrm{simples} \\ $$

Question Number 147682 Answers: 0 Comments: 0

decompose F(x)=(1/((x^n −1)(x^2 +x+1))) dans C(x) puis dans R(x)

$$\mathrm{decompose}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\left(\mathrm{x}^{\mathrm{n}} −\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{x}+\mathrm{1}\right)}\:\mathrm{dans}\:\mathrm{C}\left(\mathrm{x}\right)\:\mathrm{puis}\:\mathrm{dans}\:\mathrm{R}\left(\mathrm{x}\right) \\ $$

Question Number 147680 Answers: 0 Comments: 2

find by residus ∫_0 ^∞ ((cos(2x))/((x^2 −x+1)^3 ))dx

$$\mathrm{find}\:\mathrm{by}\:\mathrm{residus}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx} \\ $$

Question Number 147678 Answers: 0 Comments: 0

roots of Υ_n (x)=sin(narcsinx) (n integr natural) deompose F(x)=(1/(Υ_n (x)))

$$\mathrm{roots}\:\mathrm{of}\:\:\Upsilon_{\mathrm{n}} \left(\mathrm{x}\right)=\mathrm{sin}\left(\mathrm{narcsinx}\right)\:\:\left(\mathrm{n}\:\mathrm{integr}\:\mathrm{natural}\right) \\ $$$$\mathrm{deompose}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\Upsilon_{\mathrm{n}} \left(\mathrm{x}\right)} \\ $$

Question Number 147673 Answers: 3 Comments: 0

Question Number 147670 Answers: 1 Comments: 0

Question Number 147654 Answers: 0 Comments: 1

Question Number 147651 Answers: 1 Comments: 2

tan 1°+tan 5°+tan 9°+...+tan 173°+tan 177°=?

$$\:\mathrm{tan}\:\mathrm{1}°+\mathrm{tan}\:\mathrm{5}°+\mathrm{tan}\:\mathrm{9}°+...+\mathrm{tan}\:\mathrm{173}°+\mathrm{tan}\:\mathrm{177}°=? \\ $$

Question Number 147643 Answers: 2 Comments: 0

tan (x+(π/4))+3(tan (π/9)+tan ((2π)/9))=tan (x+(π/4))tan (π/9)tan ((2π)/9)

$$\:\mathrm{tan}\:\left(\mathrm{x}+\frac{\pi}{\mathrm{4}}\right)+\mathrm{3}\left(\mathrm{tan}\:\frac{\pi}{\mathrm{9}}+\mathrm{tan}\:\frac{\mathrm{2}\pi}{\mathrm{9}}\right)=\mathrm{tan}\:\left(\mathrm{x}+\frac{\pi}{\mathrm{4}}\right)\mathrm{tan}\:\frac{\pi}{\mathrm{9}}\mathrm{tan}\:\frac{\mathrm{2}\pi}{\mathrm{9}} \\ $$

Question Number 147635 Answers: 0 Comments: 5

find the taylor series f(z)=cosz ,z=(π/4)

$${find}\:{the}\:{taylor}\:{series}\:{f}\left({z}\right)={cosz}\:\:,{z}=\frac{\pi}{\mathrm{4}} \\ $$$$ \\ $$$$ \\ $$

Question Number 147626 Answers: 2 Comments: 0

∫_( 0) ^( 3) (dx/(2 + cosx)) = ?

$$\underset{\:\mathrm{0}} {\overset{\:\mathrm{3}} {\int}}\:\frac{{dx}}{\mathrm{2}\:+\:{cosx}}\:=\:? \\ $$

Question Number 147624 Answers: 0 Comments: 0

The current in the windings on a toroid is 2.0A.There are 400 turns and the mean circumferential length is 40cm.With the aid of a search coil and charge measuring instrument the magnetic field is found to be 1.0T.calculate: i)magnetic density ii)magnetization iii) magnetic susceptibility

$$\mathrm{The}\:\mathrm{current}\:\mathrm{in}\:\mathrm{the}\:\mathrm{windings}\:\mathrm{on}\:\mathrm{a}\: \\ $$$$\mathrm{toroid}\:\mathrm{is}\:\mathrm{2}.\mathrm{0A}.\mathrm{There}\:\mathrm{are}\:\mathrm{400}\:\mathrm{turns} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{mean}\:\mathrm{circumferential}\:\mathrm{length} \\ $$$$\mathrm{is}\:\mathrm{40cm}.\mathrm{With}\:\mathrm{the}\:\mathrm{aid}\:\mathrm{of}\:\mathrm{a}\:\mathrm{search} \\ $$$$\:\mathrm{coil}\:\mathrm{and}\:\mathrm{charge}\:\mathrm{measuring}\:\mathrm{instrument} \\ $$$$\mathrm{the}\:\mathrm{magnetic}\:\mathrm{field}\:\mathrm{is}\:\mathrm{found}\:\mathrm{to}\:\mathrm{be} \\ $$$$\mathrm{1}.\mathrm{0T}.\mathrm{calculate}: \\ $$$$\left.\mathrm{i}\left.\right)\mathrm{magnetic}\:\mathrm{density}\:\mathrm{ii}\right)\mathrm{magnetization} \\ $$$$\left.\mathrm{iii}\right)\:\mathrm{magnetic}\:\mathrm{susceptibility} \\ $$

Pg 706 Pg 707 Pg 708 Pg 709 Pg 710 Pg 711 Pg 712 Pg 713 Pg 714 Pg 715

Contact: info@tinkutara.com