
AllQuestion and Answers: Page 674
Question Number 150661 Answers: 0 Comments: 1
$$\mathrm{sin9x}=\mathrm{sim5x}+\mathrm{sin3x}\:\:\:\mathrm{help}\: \\ $$
Question Number 150656 Answers: 1 Comments: 0

Question Number 150655 Answers: 1 Comments: 0

Question Number 150654 Answers: 1 Comments: 0

Question Number 150647 Answers: 1 Comments: 0

Question Number 150646 Answers: 1 Comments: 0
Question Number 150652 Answers: 0 Comments: 5
Question Number 150641 Answers: 2 Comments: 1
$$\mathrm{1}+\sqrt{\mathrm{3}^{\mathrm{x}} }=\mathrm{2}^{\mathrm{x}} \\ $$$$\mathrm{x}=? \\ $$
Question Number 150628 Answers: 1 Comments: 0
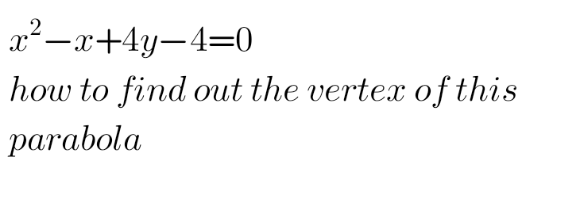
Question Number 150627 Answers: 1 Comments: 0
Question Number 150626 Answers: 1 Comments: 0
Question Number 150609 Answers: 0 Comments: 0
Question Number 150603 Answers: 1 Comments: 0
Question Number 150601 Answers: 1 Comments: 0

Question Number 150600 Answers: 3 Comments: 0
Question Number 150599 Answers: 1 Comments: 2
Question Number 150597 Answers: 1 Comments: 0

Question Number 150596 Answers: 2 Comments: 1
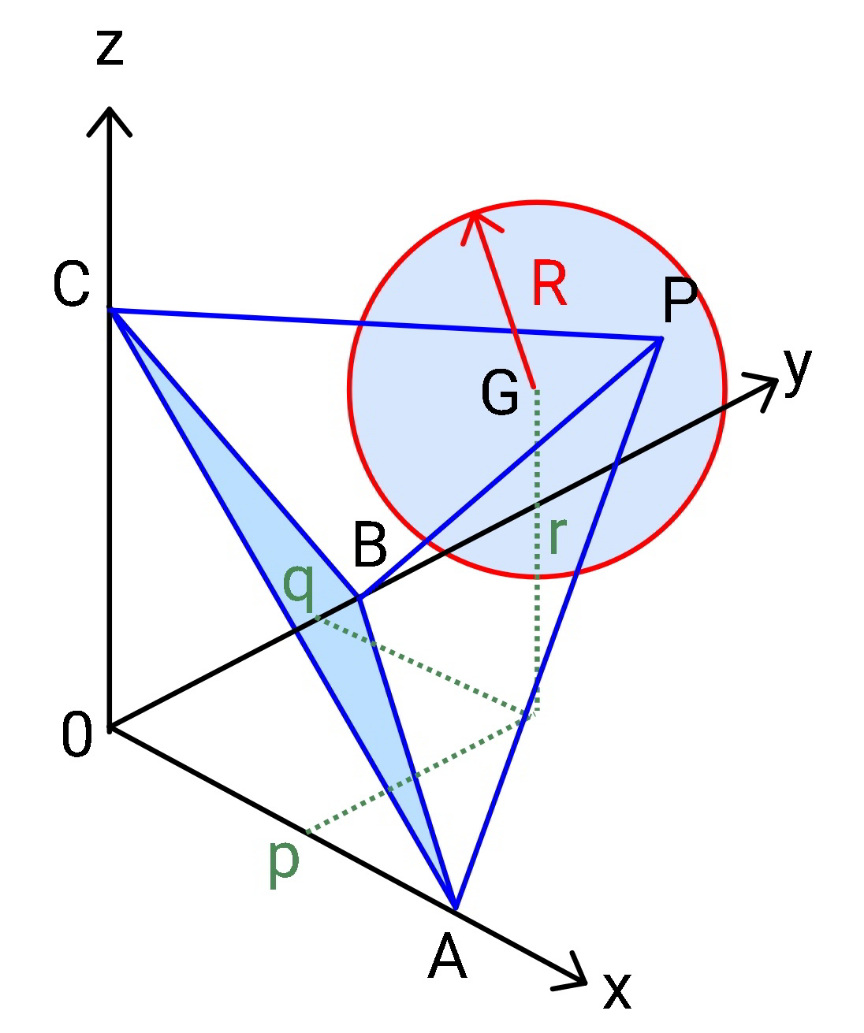
Question Number 150594 Answers: 1 Comments: 0
Question Number 150585 Answers: 0 Comments: 5
Question Number 150590 Answers: 1 Comments: 0
Question Number 150571 Answers: 3 Comments: 0
Question Number 150567 Answers: 4 Comments: 0

Question Number 150560 Answers: 3 Comments: 0

Question Number 150559 Answers: 1 Comments: 0
Question Number 150558 Answers: 1 Comments: 0
Pg 669 Pg 670 Pg 671 Pg 672 Pg 673 Pg 674 Pg 675 Pg 676 Pg 677 Pg 678
