
AllQuestion and Answers: Page 671
Question Number 150990 Answers: 1 Comments: 0
Question Number 150986 Answers: 1 Comments: 0
Question Number 150984 Answers: 0 Comments: 0
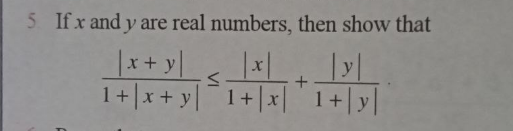
Question Number 151073 Answers: 2 Comments: 0
Question Number 151145 Answers: 1 Comments: 0

Question Number 151144 Answers: 1 Comments: 0

Question Number 150979 Answers: 1 Comments: 0
Question Number 150977 Answers: 1 Comments: 0
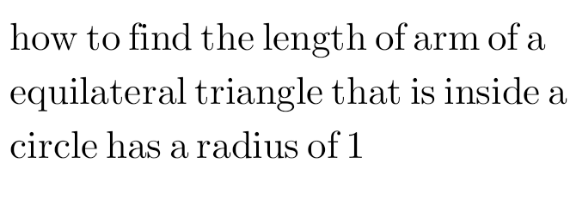
Question Number 150976 Answers: 0 Comments: 0

Question Number 150974 Answers: 0 Comments: 0

Question Number 150968 Answers: 1 Comments: 0

Question Number 150967 Answers: 1 Comments: 0
Question Number 150966 Answers: 0 Comments: 2
Question Number 150965 Answers: 1 Comments: 0
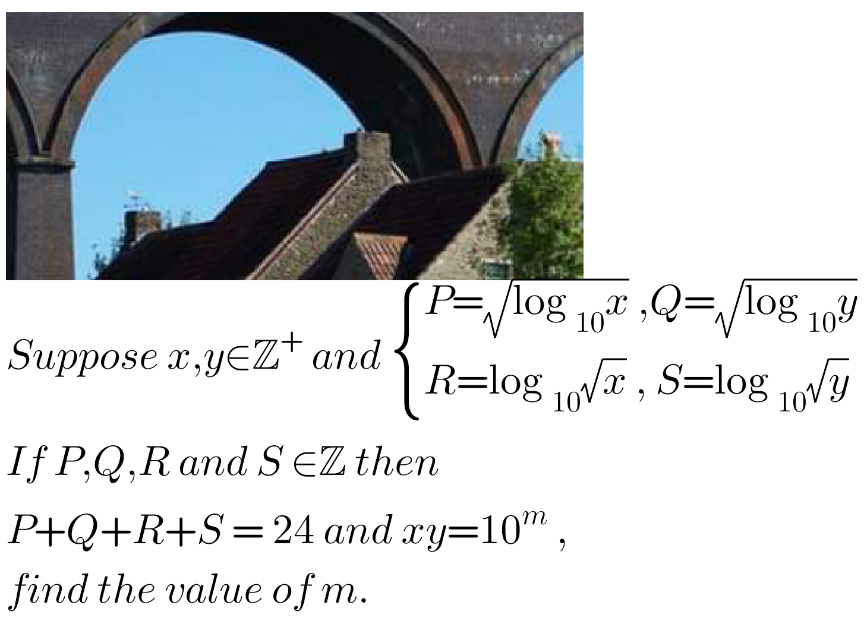
Question Number 150963 Answers: 1 Comments: 0
Question Number 150960 Answers: 1 Comments: 1
Question Number 150941 Answers: 2 Comments: 2
Question Number 150932 Answers: 1 Comments: 0
Question Number 150918 Answers: 2 Comments: 2

Question Number 150916 Answers: 1 Comments: 0
Question Number 150903 Answers: 1 Comments: 0
Question Number 150895 Answers: 1 Comments: 1
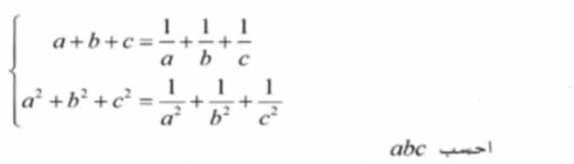
Question Number 150889 Answers: 1 Comments: 0

Question Number 150887 Answers: 1 Comments: 4

Question Number 150886 Answers: 0 Comments: 0
Question Number 150885 Answers: 0 Comments: 0

Pg 666 Pg 667 Pg 668 Pg 669 Pg 670 Pg 671 Pg 672 Pg 673 Pg 674 Pg 675
