
AllQuestion and Answers: Page 670
Question Number 150567 Answers: 4 Comments: 0

Question Number 150560 Answers: 3 Comments: 0

Question Number 150559 Answers: 1 Comments: 0
Question Number 150558 Answers: 1 Comments: 0
Question Number 150554 Answers: 1 Comments: 0
Question Number 150550 Answers: 0 Comments: 3

Question Number 150549 Answers: 0 Comments: 0
Question Number 150548 Answers: 0 Comments: 0
Question Number 150540 Answers: 0 Comments: 0
Question Number 150539 Answers: 1 Comments: 0
Question Number 150527 Answers: 1 Comments: 0
Question Number 150517 Answers: 0 Comments: 6
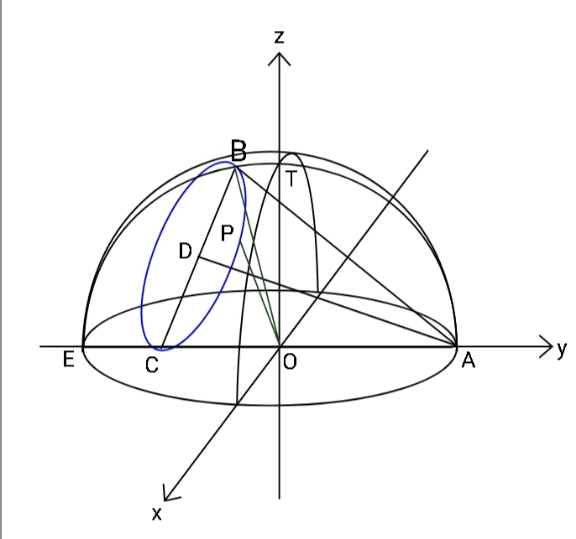
Question Number 150516 Answers: 1 Comments: 2
Question Number 150515 Answers: 2 Comments: 0

Question Number 150508 Answers: 1 Comments: 1

Question Number 150502 Answers: 0 Comments: 0

Question Number 150501 Answers: 0 Comments: 0
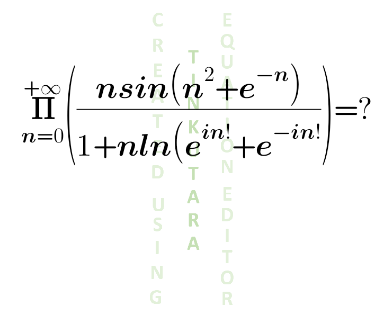
Question Number 150500 Answers: 1 Comments: 1
Question Number 150489 Answers: 1 Comments: 0
Question Number 150486 Answers: 0 Comments: 2
Question Number 150453 Answers: 0 Comments: 0
Question Number 150451 Answers: 2 Comments: 2
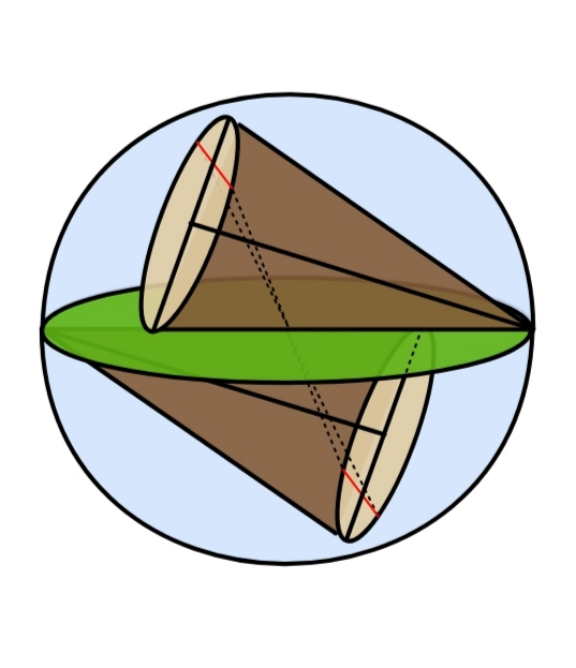
Question Number 150446 Answers: 0 Comments: 0
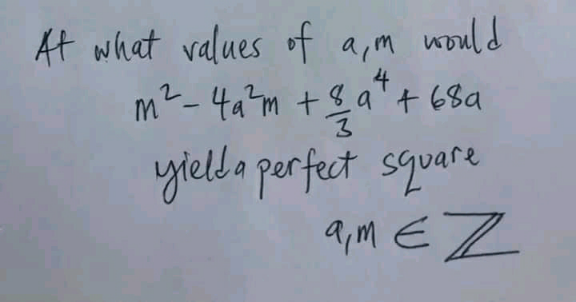
Question Number 150435 Answers: 1 Comments: 0
Question Number 150432 Answers: 1 Comments: 0
Question Number 150429 Answers: 1 Comments: 0
Pg 665 Pg 666 Pg 667 Pg 668 Pg 669 Pg 670 Pg 671 Pg 672 Pg 673 Pg 674
