
AllQuestion and Answers: Page 669
Question Number 151097 Answers: 2 Comments: 0
Question Number 151096 Answers: 0 Comments: 4
Question Number 151085 Answers: 1 Comments: 0

Question Number 151082 Answers: 0 Comments: 1
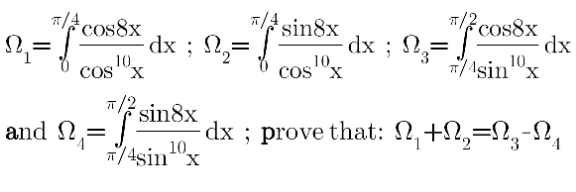
Question Number 151081 Answers: 3 Comments: 0
Question Number 151080 Answers: 2 Comments: 0
Question Number 151079 Answers: 1 Comments: 0

Question Number 151078 Answers: 0 Comments: 0
Question Number 151074 Answers: 1 Comments: 0
Question Number 151070 Answers: 0 Comments: 4
Question Number 151069 Answers: 0 Comments: 0
Question Number 151068 Answers: 0 Comments: 0
Question Number 151067 Answers: 0 Comments: 0
Question Number 151066 Answers: 0 Comments: 0
Question Number 151065 Answers: 0 Comments: 0
Question Number 151064 Answers: 0 Comments: 0
Question Number 151063 Answers: 0 Comments: 0
Question Number 151062 Answers: 0 Comments: 0
Question Number 151061 Answers: 0 Comments: 0
Question Number 151060 Answers: 0 Comments: 0
Question Number 151059 Answers: 0 Comments: 0
Question Number 151052 Answers: 1 Comments: 0
Question Number 151045 Answers: 0 Comments: 0

Question Number 151043 Answers: 2 Comments: 0
Question Number 151042 Answers: 0 Comments: 1
Question Number 151037 Answers: 1 Comments: 0
Pg 664 Pg 665 Pg 666 Pg 667 Pg 668 Pg 669 Pg 670 Pg 671 Pg 672 Pg 673
