
AllQuestion and Answers: Page 665
Question Number 151361 Answers: 1 Comments: 0
Question Number 151360 Answers: 0 Comments: 0
Question Number 151357 Answers: 1 Comments: 6

Question Number 151351 Answers: 1 Comments: 0

Question Number 151350 Answers: 0 Comments: 4
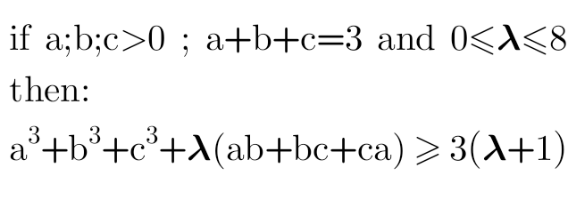
Question Number 151346 Answers: 1 Comments: 0
Question Number 151345 Answers: 1 Comments: 0
Question Number 151344 Answers: 2 Comments: 0
Question Number 151341 Answers: 1 Comments: 0
Question Number 151340 Answers: 1 Comments: 0
Question Number 151332 Answers: 1 Comments: 2
Question Number 151322 Answers: 1 Comments: 1
Question Number 151321 Answers: 0 Comments: 0

Question Number 151317 Answers: 2 Comments: 0
Question Number 151316 Answers: 1 Comments: 0
Question Number 151315 Answers: 1 Comments: 0
Question Number 151311 Answers: 0 Comments: 0

Question Number 151308 Answers: 0 Comments: 0

Question Number 151307 Answers: 2 Comments: 0
Question Number 151300 Answers: 3 Comments: 0
Question Number 151294 Answers: 1 Comments: 0

Question Number 151287 Answers: 0 Comments: 2
Question Number 151284 Answers: 1 Comments: 0
Question Number 151282 Answers: 0 Comments: 0

Question Number 151281 Answers: 0 Comments: 0

Question Number 151278 Answers: 1 Comments: 0
Pg 660 Pg 661 Pg 662 Pg 663 Pg 664 Pg 665 Pg 666 Pg 667 Pg 668 Pg 669
