
AllQuestion and Answers: Page 659
Question Number 152091 Answers: 1 Comments: 0
Question Number 152088 Answers: 2 Comments: 0
Question Number 152186 Answers: 1 Comments: 0
$$\int\mathrm{x}^{\mathrm{n}} \:\mathrm{cos}\left(\mathrm{nx}\right)\:\mathrm{dx} \\ $$
Question Number 152082 Answers: 1 Comments: 0
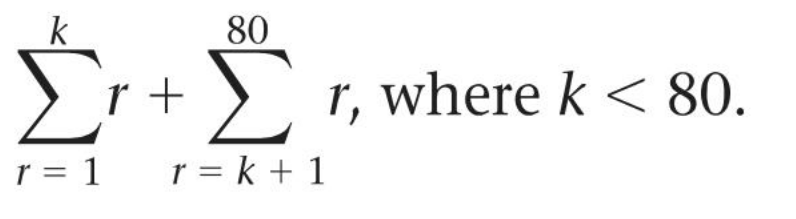
Question Number 152101 Answers: 1 Comments: 0
Question Number 152063 Answers: 2 Comments: 0
Question Number 152061 Answers: 1 Comments: 0
Question Number 152052 Answers: 1 Comments: 0
Question Number 152049 Answers: 0 Comments: 0
Question Number 152047 Answers: 0 Comments: 0
Question Number 152019 Answers: 4 Comments: 0
Question Number 152105 Answers: 1 Comments: 0
Question Number 152010 Answers: 1 Comments: 1

Question Number 152001 Answers: 1 Comments: 0
Question Number 152000 Answers: 0 Comments: 3
Question Number 151999 Answers: 2 Comments: 0
$$\int\frac{\mathrm{x}−\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{x}−\mathrm{12}} \\ $$
Question Number 152035 Answers: 1 Comments: 0
Question Number 152034 Answers: 2 Comments: 0
Question Number 152032 Answers: 0 Comments: 0

Question Number 152030 Answers: 1 Comments: 2

Question Number 152029 Answers: 1 Comments: 2

Question Number 152266 Answers: 1 Comments: 0

Question Number 151988 Answers: 0 Comments: 2
Question Number 151983 Answers: 1 Comments: 0
Question Number 151979 Answers: 3 Comments: 0
Question Number 151973 Answers: 1 Comments: 1

Pg 654 Pg 655 Pg 656 Pg 657 Pg 658 Pg 659 Pg 660 Pg 661 Pg 662 Pg 663
