
AllQuestion and Answers: Page 658
Question Number 151782 Answers: 1 Comments: 0
Question Number 151780 Answers: 1 Comments: 0

Question Number 151777 Answers: 0 Comments: 0
Question Number 151758 Answers: 2 Comments: 1
Question Number 151756 Answers: 0 Comments: 1

Question Number 151768 Answers: 1 Comments: 0
Question Number 151767 Answers: 3 Comments: 0
Question Number 151766 Answers: 0 Comments: 1

Question Number 151754 Answers: 0 Comments: 0

Question Number 151753 Answers: 0 Comments: 1

Question Number 151747 Answers: 1 Comments: 0
Question Number 151744 Answers: 1 Comments: 0
Question Number 151742 Answers: 1 Comments: 0
Question Number 151739 Answers: 0 Comments: 0
Question Number 151738 Answers: 1 Comments: 0
Question Number 151791 Answers: 1 Comments: 0

Question Number 151790 Answers: 1 Comments: 0

Question Number 151724 Answers: 0 Comments: 10

Question Number 151699 Answers: 2 Comments: 0
Question Number 151761 Answers: 1 Comments: 0
Question Number 151685 Answers: 1 Comments: 0
Question Number 151677 Answers: 1 Comments: 0
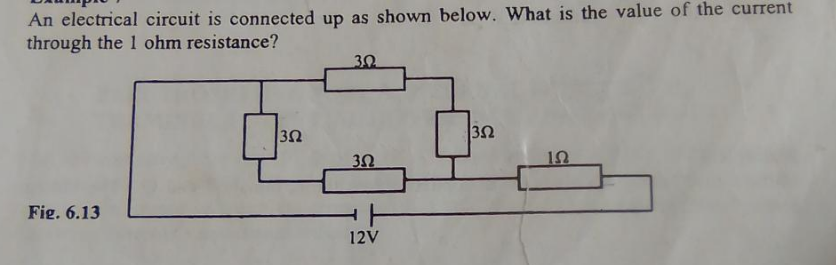
Question Number 151673 Answers: 1 Comments: 0

Question Number 151665 Answers: 2 Comments: 0
$$\mathrm{prove}\:\mathrm{4arccot5}−\mathrm{arccot239}=\frac{\pi}{\mathrm{4}} \\ $$
Question Number 151721 Answers: 0 Comments: 1

Question Number 151660 Answers: 1 Comments: 0
Pg 653 Pg 654 Pg 655 Pg 656 Pg 657 Pg 658 Pg 659 Pg 660 Pg 661 Pg 662
