Question and Answers Forum

AllQuestion and Answers: Page 657

Question Number 151894 Answers: 1 Comments: 1

∫_0 ^a x (√((a^2 −x^2 )/(a^2 +x^2 )))

$$\int_{\mathrm{0}} ^{\mathrm{a}} \:\:\:\mathrm{x}\:\sqrt{\frac{\mathrm{a}^{\mathrm{2}} −\mathrm{x}^{\mathrm{2}} }{\mathrm{a}^{\mathrm{2}} +\mathrm{x}^{\mathrm{2}} }} \\ $$

Question Number 151893 Answers: 1 Comments: 0

∫x(x^3 +a^3 )dx

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\int\mathrm{x}\left(\mathrm{x}^{\mathrm{3}} +\mathrm{a}^{\mathrm{3}} \right)\mathrm{dx} \\ $$

Question Number 151890 Answers: 1 Comments: 0

Question Number 151889 Answers: 1 Comments: 0

Compare: (((2020!)^3 ))^(1/(2020)) and 505∙2021^2

$$\mathrm{Compare}: \\ $$$$\sqrt[{\mathrm{2020}}]{\left(\mathrm{2020}!\right)^{\mathrm{3}} }\:\:\:\mathrm{and}\:\:\:\mathrm{505}\centerdot\mathrm{2021}^{\mathrm{2}} \\ $$

Question Number 151884 Answers: 2 Comments: 0

f(x)=(x-1)(x-2)...(x-2021) f^′ (2021) = ?

$$\mathrm{f}\left(\mathrm{x}\right)=\left(\mathrm{x}-\mathrm{1}\right)\left(\mathrm{x}-\mathrm{2}\right)...\left(\mathrm{x}-\mathrm{2021}\right) \\ $$$$\mathrm{f}\:^{'} \left(\mathrm{2021}\right)\:=\:? \\ $$

Question Number 151876 Answers: 1 Comments: 0

Question Number 151874 Answers: 0 Comments: 0

Question Number 151863 Answers: 2 Comments: 0

lim_(x−0) ((1−Π_(k=1) ^n cos(kx))/x^2 )=????

$$\: \\ $$$$\boldsymbol{{li}}\underset{\boldsymbol{{x}}−\mathrm{0}} {\boldsymbol{{m}}}\frac{\mathrm{1}−\underset{\boldsymbol{{k}}=\mathrm{1}} {\overset{\boldsymbol{{n}}} {\prod}}\boldsymbol{{cos}}\left(\boldsymbol{{kx}}\right)}{\boldsymbol{{x}}^{\mathrm{2}} }=???? \\ $$$$ \\ $$

Question Number 151851 Answers: 1 Comments: 0

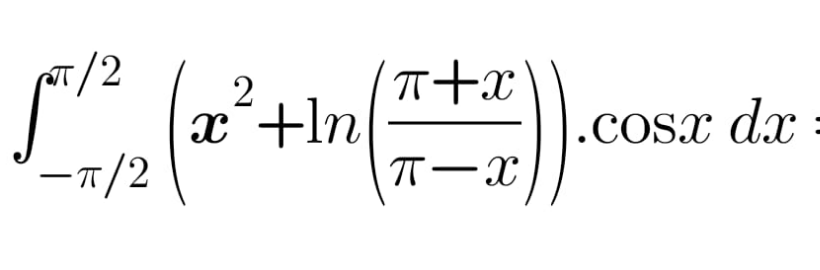
Question Number 151849 Answers: 0 Comments: 2

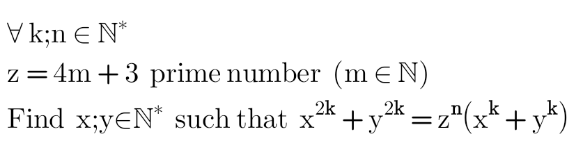
Question Number 151841 Answers: 0 Comments: 0

The volue of the limit: lim_(n→∞) (2^(−n^2 ) /(Σ_(k=n+1) ^∞ 2^(−k^2 ) )) ; (a)0 (b)some c∈(0;1) (c)1

$$\mathrm{The}\:\mathrm{volue}\:\mathrm{of}\:\mathrm{the}\:\mathrm{limit}:\: \\ $$$$\underset{\boldsymbol{\mathrm{n}}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{2}^{−\boldsymbol{\mathrm{n}}^{\mathrm{2}} } }{\underset{\boldsymbol{\mathrm{k}}=\boldsymbol{\mathrm{n}}+\mathrm{1}} {\overset{\infty} {\sum}}\mathrm{2}^{−\boldsymbol{\mathrm{k}}^{\mathrm{2}} } }\:\:\:;\:\:\:\left(\mathrm{a}\right)\mathrm{0}\:\:\left(\mathrm{b}\right)\mathrm{some}\:\mathrm{c}\in\left(\mathrm{0};\mathrm{1}\right)\:\:\left(\mathrm{c}\right)\mathrm{1} \\ $$

Question Number 151838 Answers: 0 Comments: 0

∫_0 ^( ∞) (((x^(log(⌊(⌊x⌋!)^((log(⌊x−1⌋!))^(−1) ) ⌋)+1) +1)^x )/(⌊x^(log(x^x )+1) ⌋!+1)) dx

$$\: \\ $$$$\int_{\mathrm{0}} ^{\:\infty} \:\frac{\left({x}^{\mathrm{log}\left(\lfloor\left(\lfloor{x}\rfloor!\right)^{\left(\mathrm{log}\left(\lfloor{x}−\mathrm{1}\rfloor!\right)\right)^{−\mathrm{1}} } \rfloor\right)+\mathrm{1}} +\mathrm{1}\right)^{{x}} }{\lfloor{x}^{\mathrm{log}\left({x}^{{x}} \right)+\mathrm{1}} \rfloor!+\mathrm{1}}\:{dx} \\ $$$$\: \\ $$

Question Number 151832 Answers: 3 Comments: 0

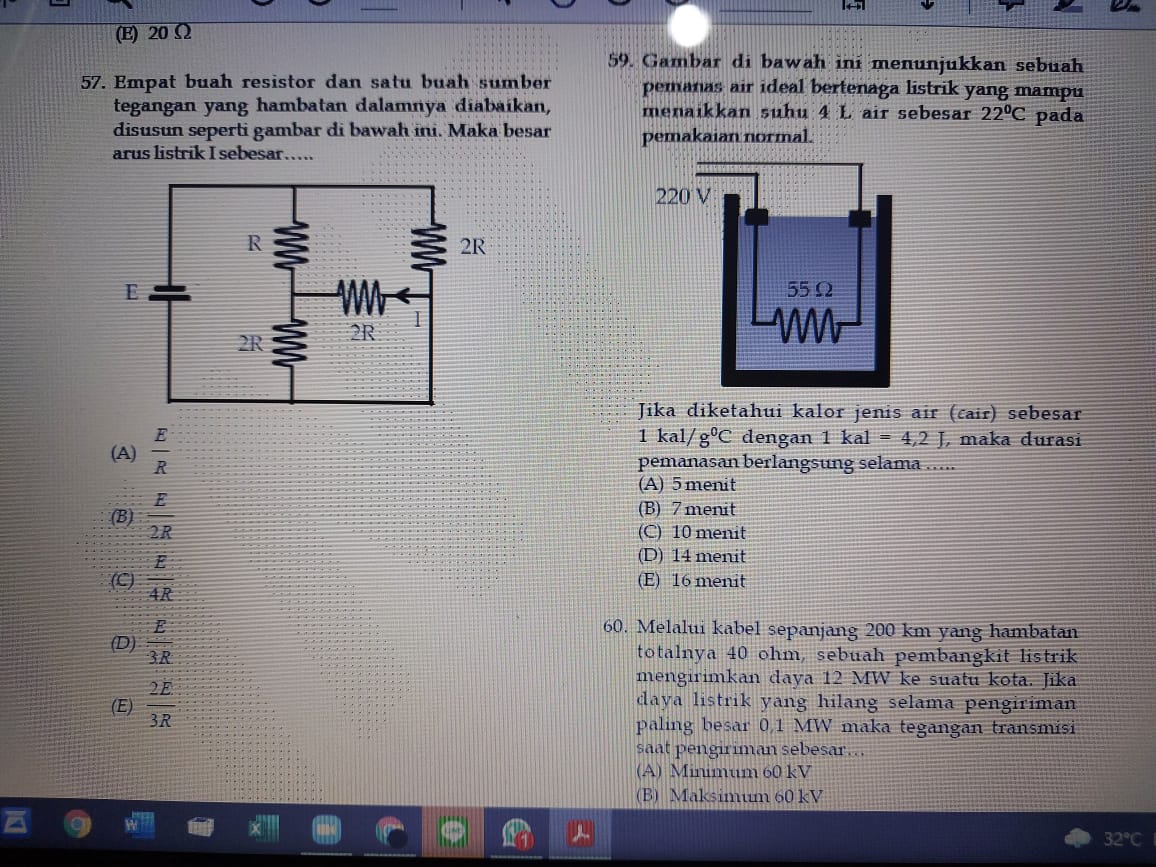
Question Number 151859 Answers: 0 Comments: 2

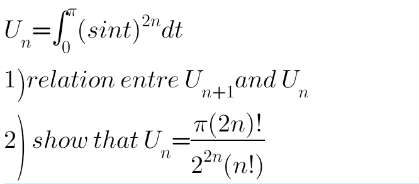
Question Number 151860 Answers: 1 Comments: 3

∫_1 ^5 ∣2−∣3−x∣∣dx

$$\underset{\mathrm{1}} {\overset{\mathrm{5}} {\int}}\mid\mathrm{2}−\mid\mathrm{3}−\boldsymbol{\mathrm{x}}\mid\mid\mathrm{dx} \\ $$

Question Number 151828 Answers: 2 Comments: 0

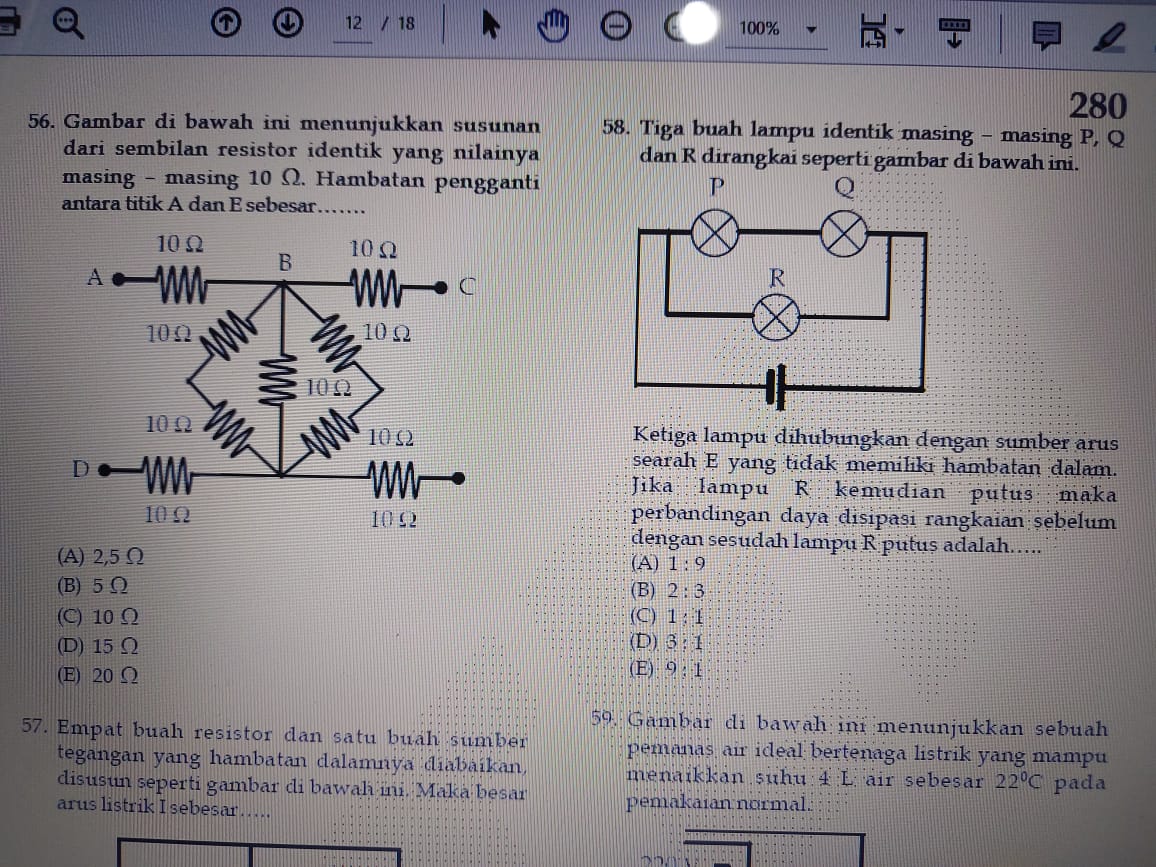
Question Number 151826 Answers: 0 Comments: 0

if x;y;z>0 prove that: (x^2 +2)(y^2 +2)(z^2 +2) ≥ 9(xy+yz+zx)

$$\mathrm{if}\:\:\mathrm{x};\mathrm{y};\mathrm{z}>\mathrm{0}\:\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\left(\mathrm{x}^{\mathrm{2}} +\mathrm{2}\right)\left(\mathrm{y}^{\mathrm{2}} +\mathrm{2}\right)\left(\mathrm{z}^{\mathrm{2}} +\mathrm{2}\right)\:\geqslant\:\mathrm{9}\left(\mathrm{xy}+\mathrm{yz}+\mathrm{zx}\right) \\ $$

Question Number 151823 Answers: 2 Comments: 0

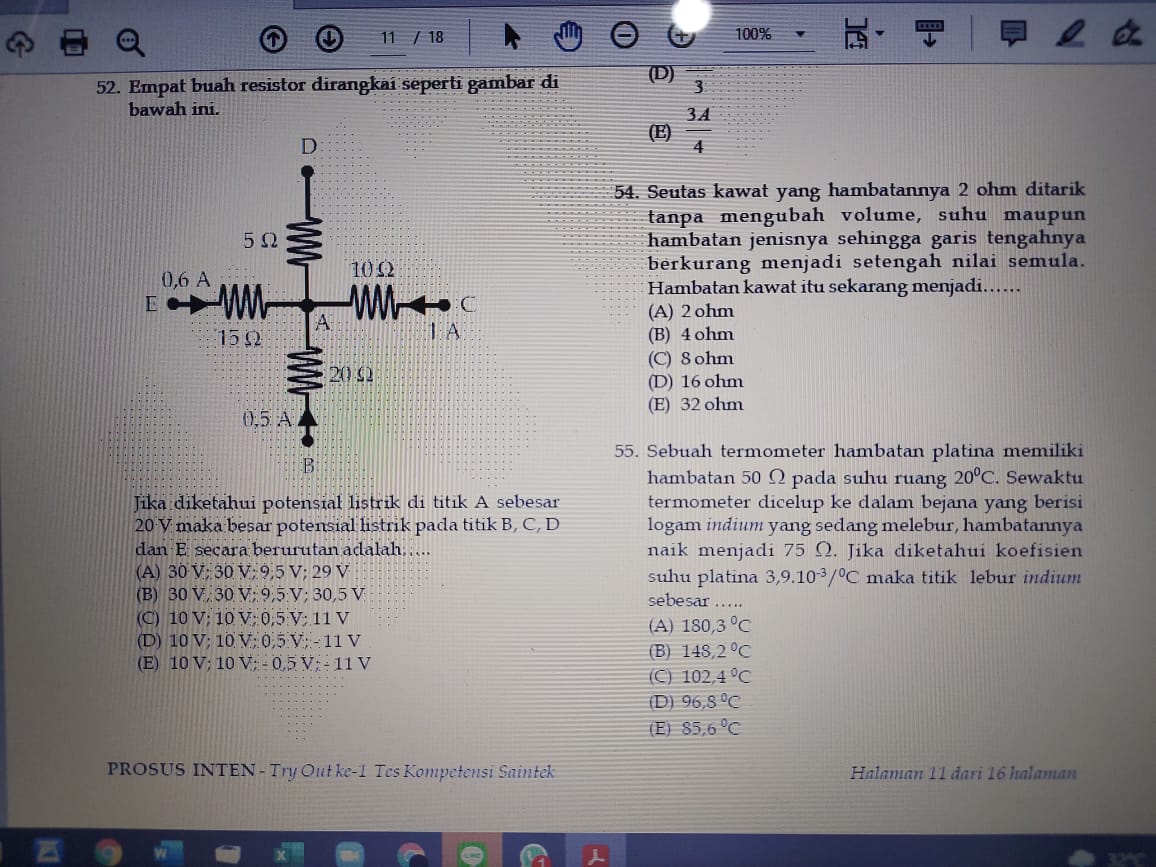
Question Number 151819 Answers: 1 Comments: 0

1. prove that : ∫_0 ^( ∞) (( e^( t) .ln(t ))/((1 + e^( t) )^( 2) )) dt=(1/2)(ln((π/2) )− γ )

$$ \\ $$$$\:\:\:\:\:\mathrm{1}.\:{prove}\:{that}\:: \\ $$$$\int_{\mathrm{0}} ^{\:\infty} \frac{\:{e}^{\:{t}} .{ln}\left({t}\:\right)}{\left(\mathrm{1}\:+\:{e}^{\:{t}} \right)^{\:\mathrm{2}} }\:{dt}=\frac{\mathrm{1}}{\mathrm{2}}\left({ln}\left(\frac{\pi}{\mathrm{2}}\:\right)−\:\gamma\:\right)\: \\ $$$$\:\:\: \\ $$

Question Number 151806 Answers: 1 Comments: 1

Question Number 151805 Answers: 0 Comments: 7

1.Let R be a relation on a set A={1,2,3,4,5,6} defined by R{(a,b):a+b≤9 then find A. R and R^(−1) B. domain and range of R and R^(−1) C.is R=R^(−1) ??

$$\mathrm{1}.\mathrm{Let}\:\mathrm{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{relation}\:\mathrm{on}\:\mathrm{a}\:\mathrm{set}\: \\ $$$$\mathrm{A}=\left\{\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4},\mathrm{5},\mathrm{6}\right\}\:\mathrm{defined}\:\mathrm{by}\: \\ $$$$\mathrm{R}\left\{\left(\mathrm{a},\mathrm{b}\right):\mathrm{a}+\mathrm{b}\leqslant\mathrm{9}\:\mathrm{then}\:\mathrm{find}\right. \\ $$$$\mathrm{A}.\:\:\mathrm{R}\:\mathrm{and}\:\mathrm{R}^{−\mathrm{1}} \\ $$$$\mathrm{B}.\:\:\mathrm{domain}\:\mathrm{and}\:\mathrm{range}\:\mathrm{of}\:\mathrm{R}\:\mathrm{and}\:\mathrm{R}^{−\mathrm{1}} \\ $$$$\mathrm{C}.\mathrm{is}\:\mathrm{R}=\mathrm{R}^{−\mathrm{1}} ?? \\ $$$$ \\ $$

Question Number 151804 Answers: 2 Comments: 0

4.Let f(x)=x^2 and g(x)=(√(x )) then find fog(x) and gof(x) and domain of(fog)(x)and(gof) are they they the same?explain. please help me???

$$\mathrm{4}.\mathrm{Let}\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \mathrm{and}\:\mathrm{g}\left(\mathrm{x}\right)=\sqrt{\mathrm{x}\:} \\ $$$$\mathrm{then}\:\mathrm{find}\:\mathrm{fog}\left(\mathrm{x}\right)\:\mathrm{and}\:\mathrm{gof}\left(\mathrm{x}\right)\:\mathrm{and} \\ $$$$\:\mathrm{domain}\:\mathrm{of}\left(\mathrm{fog}\right)\left(\mathrm{x}\right)\mathrm{and}\left(\mathrm{gof}\right) \\ $$$$\mathrm{are}\:\mathrm{they}\:\mathrm{they}\:\mathrm{the}\:\mathrm{same}?\mathrm{explain}. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{help}\:\mathrm{me}??? \\ $$$$ \\ $$

Question Number 151803 Answers: 0 Comments: 0

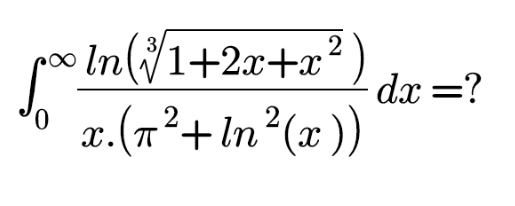
Question Number 151801 Answers: 0 Comments: 0

If a natural number of 3 digit (for example)N=abc such that N=100a+10b+c=a!+b!+c! Find all N of any number of digits. e.g. N=1, 2, 145, ...

$${If}\:{a}\:{natural}\:{number}\:{of}\:\mathrm{3}\:{digit} \\ $$$$\left({for}\:{example}\right){N}={abc}\:\:{such}\:{that} \\ $$$${N}=\mathrm{100}{a}+\mathrm{10}{b}+{c}={a}!+{b}!+{c}! \\ $$$${Find}\:{all}\:{N}\:{of}\:{any}\:{number}\:{of} \\ $$$${digits}.\: \\ $$$${e}.{g}.\:\:{N}=\mathrm{1},\:\mathrm{2},\:\mathrm{145},\:... \\ $$

Question Number 151858 Answers: 2 Comments: 0

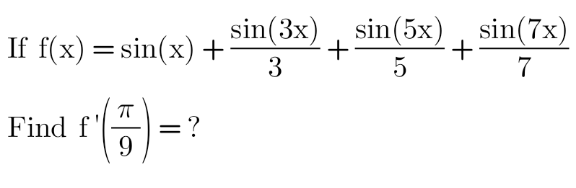
Question Number 151789 Answers: 1 Comments: 0

Pg 652 Pg 653 Pg 654 Pg 655 Pg 656 Pg 657 Pg 658 Pg 659 Pg 660 Pg 661

Contact: info@tinkutara.com