
AllQuestion and Answers: Page 655
Question Number 152533 Answers: 1 Comments: 0

Question Number 152532 Answers: 1 Comments: 0

Question Number 152522 Answers: 1 Comments: 2

Question Number 152791 Answers: 1 Comments: 0

Question Number 152513 Answers: 1 Comments: 1
$$\int\:\frac{\mathrm{1}}{\mathrm{2}{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{8}}\:{dx}\:=? \\ $$
Question Number 152502 Answers: 1 Comments: 0
Question Number 152500 Answers: 0 Comments: 0

Question Number 152498 Answers: 0 Comments: 3
Question Number 152496 Answers: 1 Comments: 0

Question Number 152494 Answers: 1 Comments: 0
Question Number 152543 Answers: 2 Comments: 0
Question Number 152486 Answers: 1 Comments: 0

Question Number 152544 Answers: 2 Comments: 1
Question Number 152481 Answers: 1 Comments: 1

Question Number 152478 Answers: 2 Comments: 0
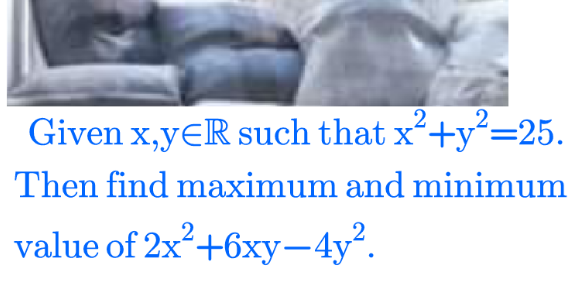
Question Number 152472 Answers: 1 Comments: 0
Question Number 152468 Answers: 1 Comments: 0
Question Number 152464 Answers: 1 Comments: 0
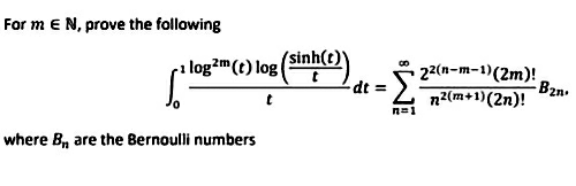
Question Number 152457 Answers: 2 Comments: 0
Question Number 152450 Answers: 1 Comments: 1

Question Number 152447 Answers: 1 Comments: 1

Question Number 152445 Answers: 0 Comments: 1

Question Number 152443 Answers: 0 Comments: 2
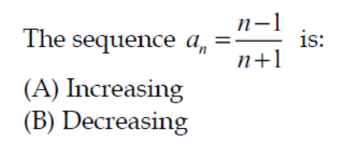
Question Number 152441 Answers: 3 Comments: 1

Question Number 152439 Answers: 0 Comments: 3

Question Number 152437 Answers: 1 Comments: 1
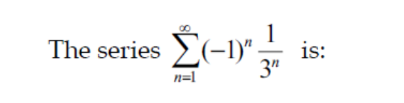
Pg 650 Pg 651 Pg 652 Pg 653 Pg 654 Pg 655 Pg 656 Pg 657 Pg 658 Pg 659
