Question and Answers Forum

AllQuestion and Answers: Page 653

Question Number 152753 Answers: 2 Comments: 12

Determine all triplets (a;b;c) of positive integers which satisfy: (1/a) + (1/b) + (1/c) = (1/2)

$$\mathrm{Determine}\:\mathrm{all}\:\mathrm{triplets}\:\left(\mathrm{a};\mathrm{b};\mathrm{c}\right)\:\mathrm{of}\:\mathrm{positive} \\ $$$$\mathrm{integers}\:\mathrm{which}\:\mathrm{satisfy}: \\ $$$$\frac{\mathrm{1}}{\mathrm{a}}\:+\:\frac{\mathrm{1}}{\mathrm{b}}\:+\:\frac{\mathrm{1}}{\mathrm{c}}\:=\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Question Number 152752 Answers: 0 Comments: 2

Given ((((x−2))^(1/3) +2))^(1/3) +((2−((x+2))^(1/3) ))^(1/3) =2 then (√(198x^4 −868x^3 −229x^2 +200x)) =?

$$\:{Given}\:\sqrt[{\mathrm{3}}]{\sqrt[{\mathrm{3}}]{{x}−\mathrm{2}}+\mathrm{2}}\:+\sqrt[{\mathrm{3}}]{\mathrm{2}−\sqrt[{\mathrm{3}}]{{x}+\mathrm{2}}}\:=\mathrm{2} \\ $$$${then}\:\sqrt{\mathrm{198}{x}^{\mathrm{4}} −\mathrm{868}{x}^{\mathrm{3}} −\mathrm{229}{x}^{\mathrm{2}} +\mathrm{200}{x}}\:=? \\ $$

Question Number 152751 Answers: 0 Comments: 0

∫_0 ^( ∞) (((cos(2cos(x)+1)+1)^(3/2) )/((ln((√(x^4 +1))−x))^4 )) dx

$$\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\infty} \:\frac{\left(\mathrm{cos}\left(\mathrm{2cos}\left({x}\right)+\mathrm{1}\right)+\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} }{\left(\mathrm{ln}\left(\sqrt{{x}^{\mathrm{4}} +\mathrm{1}}−{x}\right)\right)^{\mathrm{4}} }\:\:{dx} \\ $$$$\: \\ $$$$\: \\ $$

Question Number 152748 Answers: 1 Comments: 0

Question Number 152730 Answers: 1 Comments: 0

∫_0 ^(π/3) ((tanx)/( (√(2cosx−1))))dx

$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \frac{{tanx}}{\:\sqrt{\mathrm{2}{cosx}−\mathrm{1}}}{dx} \\ $$

Question Number 152723 Answers: 1 Comments: 0

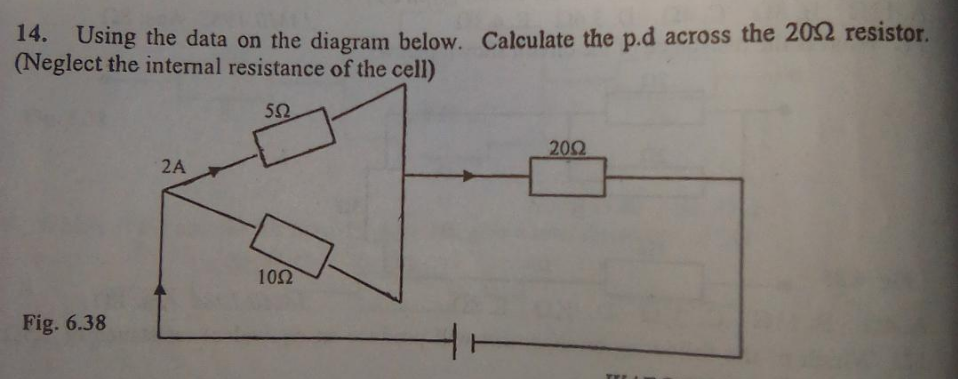
Question Number 152722 Answers: 1 Comments: 0

Question Number 152721 Answers: 0 Comments: 2

Question Number 152788 Answers: 0 Comments: 0

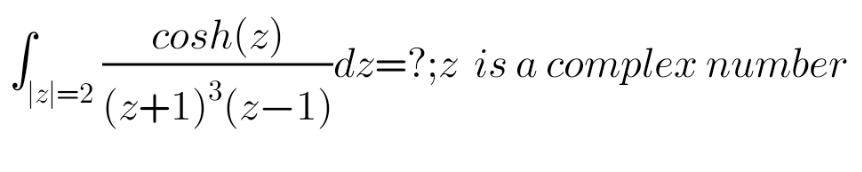
Question Number 152790 Answers: 0 Comments: 1

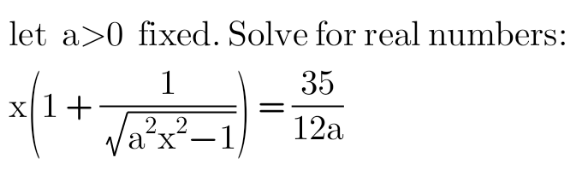
Question Number 152714 Answers: 0 Comments: 0

∫_0 ^( 1) ∫_0 ^( 1) ∫_0 ^( 1) ((x+y^2 +z^3 +1)/( (√(x+y+z))+1)) dxdydz

$$\: \\ $$$$\: \\ $$$$\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \:\:\:\frac{{x}+{y}^{\mathrm{2}} +{z}^{\mathrm{3}} +\mathrm{1}}{\:\sqrt{{x}+{y}+{z}}+\mathrm{1}}\:\:\:{dxdydz} \\ $$$$\: \\ $$$$\: \\ $$

Question Number 152797 Answers: 1 Comments: 0

nice..mathematics... Prove that... I= ∫_0 ^( ∞) (( cos (x ))/(cosh (x ))) dx=(π/( cosh ((π/2) ))) .......■ prepared :: m.n

$$ \\ $$$$\:\:\:{nice}..{mathematics}... \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\mathrm{Prove}\:\mathrm{that}... \\ $$$$\:\mathrm{I}=\:\int_{\mathrm{0}} ^{\:\infty} \frac{\:{cos}\:\left({x}\:\right)}{{cosh}\:\left({x}\:\right)}\:{dx}=\frac{\pi}{\:{cosh}\:\left(\frac{\pi}{\mathrm{2}}\:\right)}\:.......\blacksquare\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:{prepared}\:::\:\:{m}.{n} \\ $$$$ \\ $$

Question Number 152712 Answers: 0 Comments: 0

Question Number 152703 Answers: 2 Comments: 0

∫_0 ^( 1) ∫_0 ^( 1) ∫_0 ^( 1) ((x+y^2 +z^3 )/(x+y+z)) dxdydz

$$\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \:\:\frac{{x}+{y}^{\mathrm{2}} +{z}^{\mathrm{3}} }{{x}+{y}+{z}}\:\:\:{dxdydz} \\ $$$$\: \\ $$$$\: \\ $$

Question Number 152701 Answers: 0 Comments: 0

∫_(−∞) ^( ∞) (1/( (√(x^2 +1)))) dx

$$\: \\ $$$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{−\infty} ^{\:\infty} \:\frac{\mathrm{1}}{\:\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}}\:\:{dx} \\ $$$$\: \\ $$$$\: \\ $$

Question Number 152697 Answers: 0 Comments: 0

In △ABC prove that: Σ (((r_a +r_b )(r_a +r_c ))/(h_b +h_c )) ≥ ((9r)/2)

$$\mathrm{In}\:\:\bigtriangleup\mathrm{ABC}\:\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\Sigma\:\frac{\left(\mathrm{r}_{\boldsymbol{\mathrm{a}}} +\mathrm{r}_{\boldsymbol{\mathrm{b}}} \right)\left(\mathrm{r}_{\boldsymbol{\mathrm{a}}} +\mathrm{r}_{\boldsymbol{\mathrm{c}}} \right)}{\mathrm{h}_{\boldsymbol{\mathrm{b}}} +\mathrm{h}_{\boldsymbol{\mathrm{c}}} }\:\geqslant\:\frac{\mathrm{9r}}{\mathrm{2}} \\ $$

Question Number 152715 Answers: 0 Comments: 2

By eliminating θ, show that x^2 = − y^2 , if x sin^3 θ + y cos^3 θ = sinθ and x sinθ − y cosθ = 0

$$\mathrm{By}\:\mathrm{eliminating}\:\:\theta,\:\:\:\mathrm{show}\:\mathrm{that}\:\:\:\:\:\mathrm{x}^{\mathrm{2}} \:\:\:=\:\:\:−\:\:\:\mathrm{y}^{\mathrm{2}} ,\:\:\:\:\:\: \\ $$$$\mathrm{if}\:\:\:\:\:\mathrm{x}\:\mathrm{sin}^{\mathrm{3}} \theta\:\:\:+\:\:\:\mathrm{y}\:\mathrm{cos}^{\mathrm{3}} \theta\:\:\:\:=\:\:\:\:\mathrm{sin}\theta\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{and}\:\:\:\:\:\:\:\:\mathrm{x}\:\mathrm{sin}\theta\:\:\:\:−\:\:\:\mathrm{y}\:\mathrm{cos}\theta\:\:\:\:=\:\:\:\:\mathrm{0} \\ $$

Question Number 152692 Answers: 0 Comments: 0

If b and h are two integers with b>h, and b^2 +h^2 =b(a+h)+ah, find the value of b.

$$\mathrm{If}\:{b}\:\mathrm{and}\:{h}\:\mathrm{are}\:\mathrm{two}\:\mathrm{integers}\:\mathrm{with}\:{b}>{h}, \\ $$$$\mathrm{and}\:{b}^{\mathrm{2}} +{h}^{\mathrm{2}} ={b}\left({a}+{h}\right)+{ah}, \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{b}. \\ $$

Question Number 152691 Answers: 1 Comments: 0

If a,p,q are primes with a<p, and a+p=q, find the value of a.

$$\mathrm{If}\:{a},{p},{q}\:\mathrm{are}\:\mathrm{primes}\:\mathrm{with}\:{a}<{p},\:\mathrm{and} \\ $$$${a}+{p}={q},\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{a}. \\ $$

Question Number 152683 Answers: 1 Comments: 0

The probability that athlete will win a race is (1/6) and that he will be second and third are (1/4) and (1/3) respectively.what is the probability that he will not be first in the first three place! Please,help me out

$${The}\:{probability}\:{that}\:{athlete}\:{will}\:{win}\:{a}\:{race}\:{is}\:\frac{\mathrm{1}}{\mathrm{6}}\:{and}\:{that} \\ $$$${he}\:{will}\:{be}\:{second}\:{and}\:{third}\:{are}\:\frac{\mathrm{1}}{\mathrm{4}}\:{and}\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${respectively}.{what}\:{is}\:{the}\:{probability}\:{that}\:{he}\:{will}\:{not}\:{be}\:{first} \\ $$$${in}\:{the}\:{first}\:{three}\:{place}! \\ $$$${Please},{help}\:{me}\:{out} \\ $$

Question Number 152682 Answers: 1 Comments: 0

Question Number 152672 Answers: 1 Comments: 0

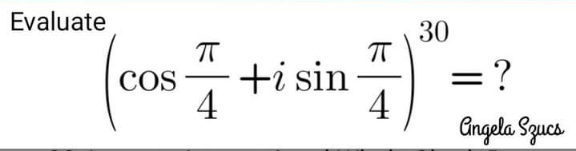
Question Number 152671 Answers: 3 Comments: 2

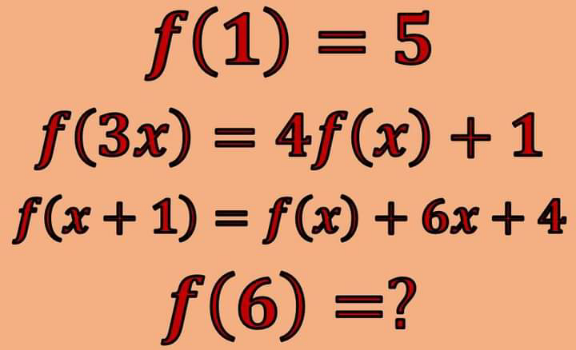
Question Number 152670 Answers: 1 Comments: 0

Question Number 152678 Answers: 2 Comments: 0

Find the sum of the real roots of equations: a^3 -6a^2 +15a-17=0 and a^3 -6a^2 +15a-11=0

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{real}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{equations}: \\ $$$$\mathrm{a}^{\mathrm{3}} -\mathrm{6a}^{\mathrm{2}} +\mathrm{15a}-\mathrm{17}=\mathrm{0}\:\:\:\mathrm{and} \\ $$$$\mathrm{a}^{\mathrm{3}} -\mathrm{6a}^{\mathrm{2}} +\mathrm{15a}-\mathrm{11}=\mathrm{0} \\ $$

Question Number 152663 Answers: 1 Comments: 0

If x^3 -x+3=0 has the roots a, b and c. determine the monic polynomial with the roots a^5 , b^5 and c^5 . [Q152396]

$$\mathrm{If}\:\:\mathrm{x}^{\mathrm{3}} -\mathrm{x}+\mathrm{3}=\mathrm{0}\:\mathrm{has}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{a},\:\mathrm{b}\:\mathrm{and}\:\mathrm{c}. \\ $$$$\mathrm{determine}\:\mathrm{the}\:\mathrm{monic}\:\mathrm{polynomial}\:\mathrm{with} \\ $$$$\mathrm{the}\:\mathrm{roots}\:\:\mathrm{a}^{\mathrm{5}} ,\:\mathrm{b}^{\mathrm{5}} \:\mathrm{and}\:\:\mathrm{c}^{\mathrm{5}} . \\ $$$$\left[{Q}\mathrm{152396}\right] \\ $$

Pg 648 Pg 649 Pg 650 Pg 651 Pg 652 Pg 653 Pg 654 Pg 655 Pg 656 Pg 657

Contact: info@tinkutara.com