
AllQuestion and Answers: Page 652
Question Number 152826 Answers: 1 Comments: 0
Question Number 152817 Answers: 4 Comments: 0
Question Number 152805 Answers: 2 Comments: 0

Question Number 152801 Answers: 0 Comments: 3
$${resouds} \\ $$$$\mid\mathrm{1}−{x}\mid{y}'+{xy}={x} \\ $$
Question Number 152799 Answers: 1 Comments: 1
Question Number 152795 Answers: 1 Comments: 0
Question Number 152779 Answers: 0 Comments: 4

Question Number 152778 Answers: 0 Comments: 0
Question Number 152772 Answers: 1 Comments: 2
Question Number 152771 Answers: 0 Comments: 2
Question Number 152770 Answers: 0 Comments: 1
Question Number 152769 Answers: 1 Comments: 1
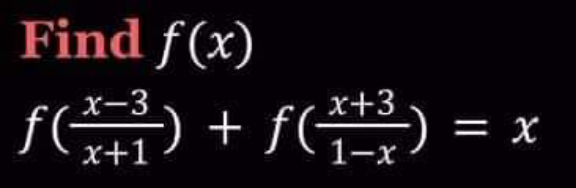
Question Number 152768 Answers: 0 Comments: 0
Question Number 152764 Answers: 3 Comments: 2
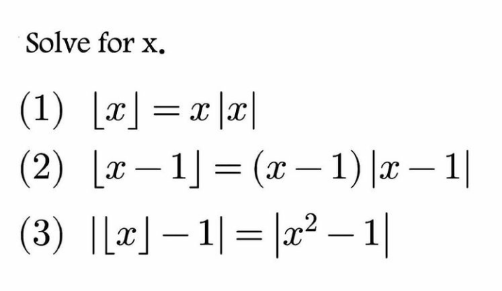
Question Number 152761 Answers: 0 Comments: 0
Question Number 152757 Answers: 1 Comments: 0
Question Number 152793 Answers: 0 Comments: 2
Question Number 152753 Answers: 2 Comments: 12
Question Number 152752 Answers: 0 Comments: 2
Question Number 152751 Answers: 0 Comments: 0
Question Number 152748 Answers: 1 Comments: 0

Question Number 152730 Answers: 1 Comments: 0
Question Number 152723 Answers: 1 Comments: 0
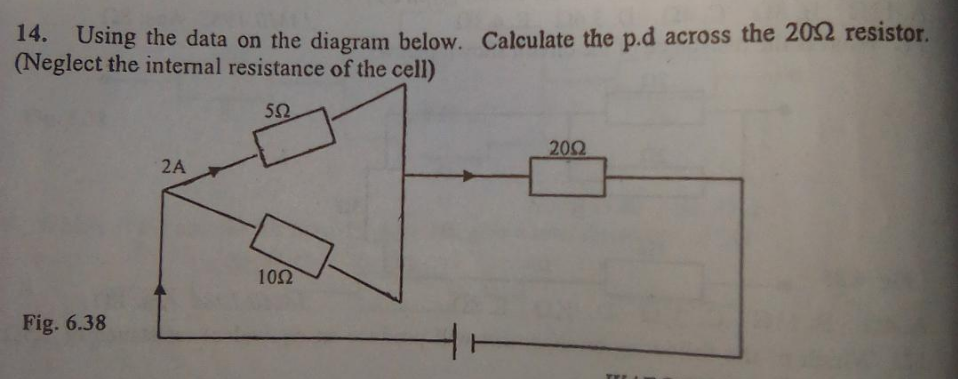
Question Number 152722 Answers: 1 Comments: 0

Question Number 152721 Answers: 0 Comments: 2

Question Number 152788 Answers: 0 Comments: 0
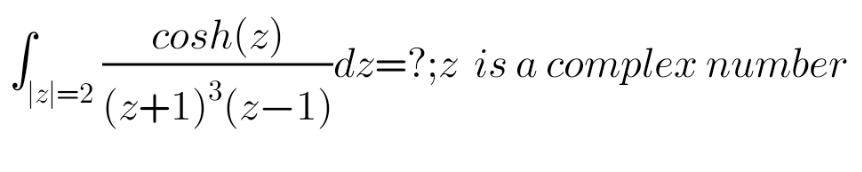
Pg 647 Pg 648 Pg 649 Pg 650 Pg 651 Pg 652 Pg 653 Pg 654 Pg 655 Pg 656
