Question and Answers Forum

AllQuestion and Answers: Page 650

Question Number 153973 Answers: 2 Comments: 0

Question Number 153972 Answers: 2 Comments: 0

soit:f→x^3 +3x+1 alors:(f^(_1) )^(′′) (5)=?

$${soit}:{f}\rightarrow{x}^{\mathrm{3}} +\mathrm{3}{x}+\mathrm{1} \\ $$$${alors}:\left({f}^{\_\mathrm{1}} \right)^{''} \left(\mathrm{5}\right)=? \\ $$

Question Number 153965 Answers: 1 Comments: 0

If 3x^2 −2xy+y^2 =1, prove that (d^2 y/dx^2 )=(2/((x−y)^3 ))

$$\mathrm{If}\:\mathrm{3}{x}^{\mathrm{2}} −\mathrm{2}{xy}+{y}^{\mathrm{2}} =\mathrm{1},\:\mathrm{prove}\:\mathrm{that}\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }=\frac{\mathrm{2}}{\left({x}−{y}\right)^{\mathrm{3}} } \\ $$

Question Number 153963 Answers: 2 Comments: 0

Given that f and g are differentiable functions such that f ′(x)=(1/( (√(1+(f(x))^2 )) )), and g=f^( −1) , find g′(x).

$$\mathrm{Given}\:\mathrm{that}\:{f}\:\mathrm{and}\:{g}\:\mathrm{are}\:\mathrm{differentiable} \\ $$$$\mathrm{functions}\:\mathrm{such}\:\mathrm{that}\:{f}\:'\left({x}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\left({f}\left({x}\right)\right)^{\mathrm{2}} }\:}, \\ $$$$\mathrm{and}\:{g}={f}^{\:−\mathrm{1}} \:,\:\mathrm{find}\:{g}'\left({x}\right). \\ $$

Question Number 153958 Answers: 4 Comments: 0

Question Number 153959 Answers: 1 Comments: 0

Question Number 153956 Answers: 2 Comments: 0

Max & min value of function f(x)=(√(6−x)) +(√(12+x)) .

$$\:{Max}\:\&\:{min}\:{value}\:{of}\:{function} \\ $$$$\:{f}\left({x}\right)=\sqrt{\mathrm{6}−{x}}\:+\sqrt{\mathrm{12}+{x}}\:. \\ $$

Question Number 153950 Answers: 1 Comments: 0

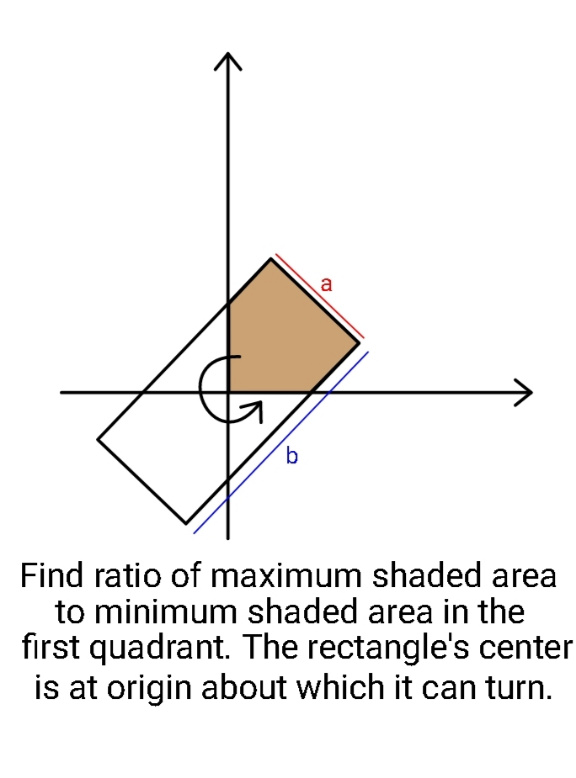
Question Number 153949 Answers: 1 Comments: 0

∫_0 ^( ∞) sin(x^2 )cos(x^3 )dx

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\infty} \:\mathrm{sin}\left({x}^{\mathrm{2}} \right)\mathrm{cos}\left({x}^{\mathrm{3}} \right){dx} \\ $$$$\: \\ $$

Question Number 154089 Answers: 2 Comments: 0

Question Number 154090 Answers: 0 Comments: 0

$$ \\ $$

Question Number 153946 Answers: 0 Comments: 0

show whether ∫_0 ^( ∞) sin(x^2 )cos((√x))dx is solvable

$$\: \\ $$$$\:\:\mathrm{show}\:\mathrm{whether} \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\infty} \:\mathrm{sin}\left({x}^{\mathrm{2}} \right)\mathrm{cos}\left(\sqrt{{x}}\right){dx} \\ $$$$\:\:\mathrm{is}\:\mathrm{solvable} \\ $$$$\: \\ $$

Question Number 153934 Answers: 0 Comments: 0

Question Number 153977 Answers: 1 Comments: 0

Question Number 153924 Answers: 2 Comments: 0

∫ ((1+sin x)/(sin x(1+cos x))) dx =?

$$\int\:\frac{\mathrm{1}+\mathrm{sin}\:{x}}{\mathrm{sin}\:{x}\left(\mathrm{1}+\mathrm{cos}\:{x}\right)}\:{dx}\:=? \\ $$

Question Number 153920 Answers: 0 Comments: 0

Question Number 153918 Answers: 1 Comments: 0

the base of an object is in the form of a circle with radius 1. suppose that all section of the object are perpendicular to a diameter of a square. determine the volume of the object?

$$\mathrm{the}\:\mathrm{base}\:\mathrm{of}\:\mathrm{an}\:\mathrm{object}\:\mathrm{is}\:\mathrm{in}\:\mathrm{the}\:\mathrm{form}\:\mathrm{of}\:\mathrm{a}\:\mathrm{circle}\:\mathrm{with} \\ $$$$\mathrm{radius}\:\mathrm{1}.\:\mathrm{suppose}\:\mathrm{that}\:\mathrm{all}\:\mathrm{section}\:\mathrm{of}\:\mathrm{the}\:\mathrm{object}\:\mathrm{are} \\ $$$$\mathrm{perpendicular}\:\mathrm{to}\:\mathrm{a}\:\mathrm{diameter}\:\mathrm{of}\:\mathrm{a}\:\mathrm{square}.\:\mathrm{determine} \\ $$$$\mathrm{the}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{the}\:\mathrm{object}? \\ $$

Question Number 153916 Answers: 0 Comments: 0

The value of Σ_(n=0) ^∞ (((3_n )(2_n )x^n )/((1_n )n!)) β(2,n+1) is a. (1/2)Σ_(n=0) ^∞ (2_n )(x^n /(n!)) b. (1/2)Σ_(n=0) ^∞ (((3_n )(2_n ))/((1_n ))) (x^n /(n!)) c. (1/2)Σ_(n=0) ^∞ (((2_n )x^n )/((1_n )n!)) d. (1/3)Σ_(n=0) ^∞ (((3_n )x^n )/((1_n )n!))

$${The}\:{value}\:{of}\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\:\frac{\left(\mathrm{3}_{{n}} \right)\left(\mathrm{2}_{{n}} \right){x}^{{n}} }{\left(\mathrm{1}_{{n}} \right){n}!}\:\beta\left(\mathrm{2},{n}+\mathrm{1}\right)\:{is} \\ $$$${a}.\:\frac{\mathrm{1}}{\mathrm{2}}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{2}_{{n}} \right)\frac{{x}^{{n}} }{{n}!} \\ $$$${b}.\:\frac{\mathrm{1}}{\mathrm{2}}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{3}_{{n}} \right)\left(\mathrm{2}_{{n}} \right)}{\left(\mathrm{1}_{{n}} \right)}\:\frac{{x}^{{n}} }{{n}!} \\ $$$${c}.\:\frac{\mathrm{1}}{\mathrm{2}}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{2}_{{n}} \right){x}^{{n}} }{\left(\mathrm{1}_{{n}} \right){n}!} \\ $$$${d}.\:\frac{\mathrm{1}}{\mathrm{3}}\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(\mathrm{3}_{{n}} \right){x}^{{n}} }{\left(\mathrm{1}_{{n}} \right){n}!} \\ $$

Question Number 153915 Answers: 1 Comments: 0

Question Number 153912 Answers: 2 Comments: 0

Question Number 153905 Answers: 2 Comments: 1

Question Number 153903 Answers: 0 Comments: 0

Question Number 153901 Answers: 2 Comments: 1

Question Number 153899 Answers: 0 Comments: 0

Determine whether there exists 2016 distinct prime numbers p_1 ,p_2 ,...,p_(2016) and positive integer n such that: Σ_(i=1) ^(2016) (1/(p_i ^2 + 1)) = (1/n^2 )

$$\mathrm{Determine}\:\mathrm{whether}\:\mathrm{there}\:\mathrm{exists}\:\:\mathrm{2016} \\ $$$$\mathrm{distinct}\:\mathrm{prime}\:\mathrm{numbers}\:\:\mathrm{p}_{\mathrm{1}} ,\mathrm{p}_{\mathrm{2}} ,...,\mathrm{p}_{\mathrm{2016}} \\ $$$$\mathrm{and}\:\mathrm{positive}\:\mathrm{integer}\:\:\boldsymbol{\mathrm{n}}\:\:\mathrm{such}\:\mathrm{that}: \\ $$$$\underset{\boldsymbol{\mathrm{i}}=\mathrm{1}} {\overset{\mathrm{2016}} {\sum}}\:\frac{\mathrm{1}}{\mathrm{p}_{\boldsymbol{\mathrm{i}}} ^{\mathrm{2}} \:+\:\mathrm{1}}\:=\:\frac{\mathrm{1}}{\mathrm{n}^{\mathrm{2}} } \\ $$

Question Number 153898 Answers: 1 Comments: 0

Find all functions f:Q→Q satisfying these followong conditions for all x∈Q 1. f(x + 1) = f(x) + 1 2. f(x^3 ) = f^( 3) (x)

$$\mathrm{Find}\:\mathrm{all}\:\mathrm{functions}\:\:\mathrm{f}:\mathrm{Q}\rightarrow\mathrm{Q}\:\:\mathrm{satisfying} \\ $$$$\mathrm{these}\:\mathrm{followong}\:\mathrm{conditions}\:\mathrm{for}\:\mathrm{all}\:\boldsymbol{\mathrm{x}}\in\mathrm{Q} \\ $$$$\mathrm{1}.\:\mathrm{f}\left(\mathrm{x}\:+\:\mathrm{1}\right)\:=\:\mathrm{f}\left(\mathrm{x}\right)\:+\:\mathrm{1} \\ $$$$\mathrm{2}.\:\mathrm{f}\left(\mathrm{x}^{\mathrm{3}} \right)\:=\:\mathrm{f}^{\:\mathrm{3}} \left(\mathrm{x}\right) \\ $$

Question Number 153896 Answers: 1 Comments: 0

Pg 645 Pg 646 Pg 647 Pg 648 Pg 649 Pg 650 Pg 651 Pg 652 Pg 653 Pg 654

Contact: info@tinkutara.com