Question and Answers Forum

AllQuestion and Answers: Page 645

Question Number 154309 Answers: 1 Comments: 0

Question Number 154306 Answers: 0 Comments: 1

Question Number 154303 Answers: 1 Comments: 1

Solve in R ((z^9 - 81z - 62)/z^3 ) = 18 ((3z + 2))^(1/3)

$$\mathrm{Solve}\:\mathrm{in}\:\mathbb{R} \\ $$$$\frac{\mathrm{z}^{\mathrm{9}} \:-\:\mathrm{81z}\:-\:\mathrm{62}}{\mathrm{z}^{\mathrm{3}} }\:=\:\mathrm{18}\:\sqrt[{\mathrm{3}}]{\mathrm{3z}\:+\:\mathrm{2}} \\ $$

Question Number 154301 Answers: 1 Comments: 0

of the integers 101 to 400 (including 101 and 400 themselves) how many are not divisible by 3 or 5?

$$\mathrm{of}\:\mathrm{the}\:\mathrm{integers}\:\mathrm{101}\:\mathrm{to}\:\mathrm{400}\:\left(\mathrm{including}\:\mathrm{101}\:\mathrm{and}\:\mathrm{400}\:\right. \\ $$$$\left.\mathrm{themselves}\right)\:\mathrm{how}\:\mathrm{many}\:\mathrm{are}\:\mathrm{not}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{3}\:\mathrm{or}\:\mathrm{5}? \\ $$

Question Number 154296 Answers: 0 Comments: 0

Question Number 154292 Answers: 2 Comments: 0

Question Number 154291 Answers: 2 Comments: 0

Among the integers 101−400,how many numbers are divisible by 3 but no divisible by 5 or 7

$$\mathrm{Among}\:\mathrm{the}\:\mathrm{integers}\:\mathrm{101}−\mathrm{400},\mathrm{how}\:\mathrm{many}\:\mathrm{numbers} \\ $$$$\mathrm{are}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{3}\:\mathrm{but}\:\mathrm{no}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{5}\:\mathrm{or}\:\mathrm{7} \\ $$

Question Number 154289 Answers: 0 Comments: 0

solve ∫4x^(5x) dx=?

$${solve}\: \\ $$$$\int\mathrm{4}{x}^{\mathrm{5}{x}} {dx}=? \\ $$

Question Number 154287 Answers: 0 Comments: 0

Question Number 154285 Answers: 0 Comments: 0

Question Number 154286 Answers: 1 Comments: 5

h = ((52−47i))^(1/3) +((52+47i))^(1/3) find h^2 .

$$\:{h}\:=\:\sqrt[{\mathrm{3}}]{\mathrm{52}−\mathrm{47}{i}}\:+\sqrt[{\mathrm{3}}]{\mathrm{52}+\mathrm{47}{i}}\: \\ $$$$\:{find}\:{h}^{\mathrm{2}} . \\ $$

Question Number 154281 Answers: 0 Comments: 0

∫_1 ^3 ⌊x−3⌋dx

$$\int_{\mathrm{1}} ^{\mathrm{3}} \lfloor{x}−\mathrm{3}\rfloor{dx} \\ $$

Question Number 154280 Answers: 0 Comments: 0

∫_0 ^( 1) ∫_0 ^( z^2 ) ∫_0 ^( 3) y cos (z^5 )dxdydz =?

$$\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:{z}^{\mathrm{2}} } \int_{\mathrm{0}} ^{\:\mathrm{3}} {y}\:\mathrm{cos}\:\left({z}^{\mathrm{5}} \right){dxdydz}\:=? \\ $$

Question Number 154275 Answers: 0 Comments: 1

Question Number 154274 Answers: 0 Comments: 1

Solve the equation: x^2 ∙2^x (x^4 ∙4^x + 3∙15^x ) = 125^x - 27^x

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{equation}: \\ $$$$\mathrm{x}^{\mathrm{2}} \centerdot\mathrm{2}^{\boldsymbol{\mathrm{x}}} \left(\mathrm{x}^{\mathrm{4}} \centerdot\mathrm{4}^{\boldsymbol{\mathrm{x}}} \:+\:\mathrm{3}\centerdot\mathrm{15}^{\boldsymbol{\mathrm{x}}} \right)\:=\:\mathrm{125}^{\boldsymbol{\mathrm{x}}} \:-\:\mathrm{27}^{\boldsymbol{\mathrm{x}}} \\ $$

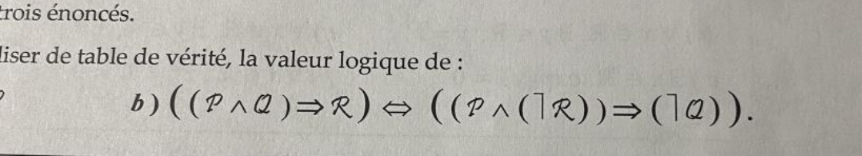
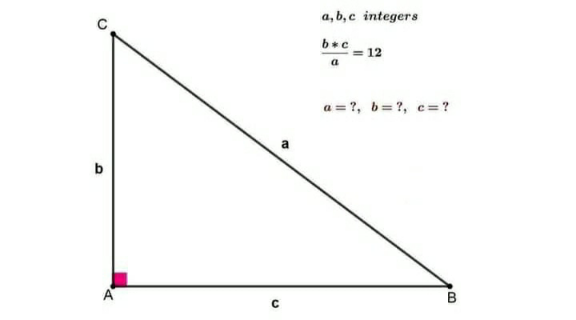
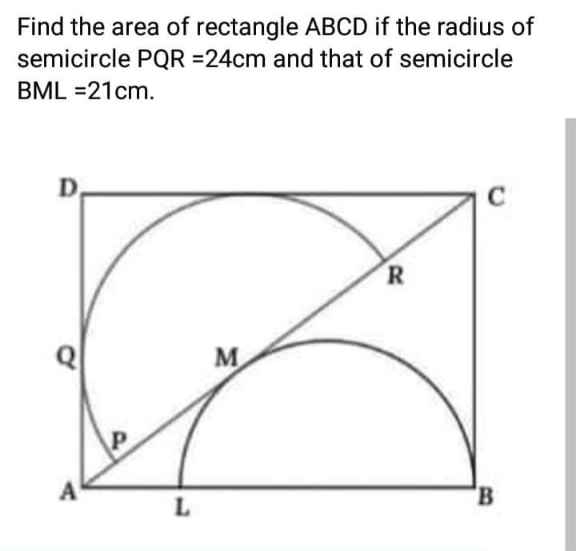
Question Number 154273 Answers: 1 Comments: 0

Question Number 154270 Answers: 0 Comments: 0

Question Number 154268 Answers: 0 Comments: 3

Question Number 154267 Answers: 0 Comments: 0

if x;y;z>0 and xy+yz+zx=3xyz then: (Σ_(cyc) (1/(x+y)))(Σ_(cyc) (1/(2x+y+z))) ≤ (9/8)

$$\mathrm{if}\:\:\mathrm{x};\mathrm{y};\mathrm{z}>\mathrm{0}\:\mathrm{and}\:\mathrm{xy}+\mathrm{yz}+\mathrm{zx}=\mathrm{3xyz}\:\:\mathrm{then}: \\ $$$$\left(\underset{\boldsymbol{\mathrm{cyc}}} {\sum}\:\frac{\mathrm{1}}{\mathrm{x}+\mathrm{y}}\right)\left(\underset{\boldsymbol{\mathrm{cyc}}} {\sum}\:\frac{\mathrm{1}}{\mathrm{2x}+\mathrm{y}+\mathrm{z}}\right)\:\leqslant\:\frac{\mathrm{9}}{\mathrm{8}} \\ $$

Question Number 154262 Answers: 1 Comments: 0

Question Number 154258 Answers: 1 Comments: 0

Question Number 154255 Answers: 1 Comments: 0

(5/(3^2 .7^2 ))+(9/(7^2 .11^2 ))+((13)/(11^2 .15^2 ))+…=?

$$\:\frac{\mathrm{5}}{\mathrm{3}^{\mathrm{2}} .\mathrm{7}^{\mathrm{2}} }+\frac{\mathrm{9}}{\mathrm{7}^{\mathrm{2}} .\mathrm{11}^{\mathrm{2}} }+\frac{\mathrm{13}}{\mathrm{11}^{\mathrm{2}} .\mathrm{15}^{\mathrm{2}} }+\ldots=? \\ $$

Question Number 154254 Answers: 0 Comments: 0

form a PDE from Z(x,y)=xf_1 (x−y)+2xf_2 (2x+y)

$${form}\:{a}\:{PDE}\:{from}\:{Z}\left({x},{y}\right)={xf}_{\mathrm{1}} \left({x}−{y}\right)+\mathrm{2}{xf}_{\mathrm{2}} \left(\mathrm{2}{x}+{y}\right) \\ $$

Question Number 154252 Answers: 0 Comments: 0

The {x_n } sequence is specified by the conditions { ((x_1 =1982)),((x_(n+1) =(1/(4−x_n )),n≥0)) :} find lim_(n→∞) x_n .

$$\:\:{The}\:\left\{{x}_{{n}} \right\}\:{sequence}\:{is}\:{specified} \\ $$$$\:{by}\:{the}\:{conditions}\: \\ $$$$\:\:\begin{cases}{{x}_{\mathrm{1}} =\mathrm{1982}}\\{{x}_{{n}+\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{4}−{x}_{{n}} },{n}\geqslant\mathrm{0}}\end{cases} \\ $$$$\:{find}\:\underset{{n}\rightarrow\infty} {\mathrm{lim}}{x}_{{n}} . \\ $$

Question Number 154250 Answers: 0 Comments: 0

Question Number 154240 Answers: 1 Comments: 0

{ ((tg(a+b)=7)),((tg(a−b)=5)) :} tg(a)=? easy question

$$\begin{cases}{{tg}\left({a}+{b}\right)=\mathrm{7}}\\{{tg}\left({a}−{b}\right)=\mathrm{5}}\end{cases}\:\:{tg}\left({a}\right)=?\:\:\:{easy}\:{question} \\ $$

Pg 640 Pg 641 Pg 642 Pg 643 Pg 644 Pg 645 Pg 646 Pg 647 Pg 648 Pg 649

Contact: info@tinkutara.com