Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 642

Question Number 152791 Answers: 1 Comments: 0

Question Number 152513 Answers: 1 Comments: 1

∫ (1/(2x^2 +3x+8)) dx =?

$$\int\:\frac{\mathrm{1}}{\mathrm{2}{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{8}}\:{dx}\:=? \\ $$

Question Number 152502 Answers: 1 Comments: 0

∫ 9x^2 (4x^2 + 3)^(10) dx

$$\int\:\mathrm{9x}^{\mathrm{2}} \left(\mathrm{4x}^{\mathrm{2}} \:\:+\:\:\mathrm{3}\right)^{\mathrm{10}} \:\mathrm{dx} \\ $$

Question Number 152500 Answers: 0 Comments: 0

Question Number 152498 Answers: 0 Comments: 3

if ((√5) + 2)^6 < x find min(x) = ?

$$\mathrm{if}\:\:\left(\sqrt{\mathrm{5}}\:+\:\mathrm{2}\right)^{\mathrm{6}} \:<\:\mathrm{x} \\ $$$$\mathrm{find}\:\:\mathrm{min}\left(\mathrm{x}\right)\:=\:? \\ $$

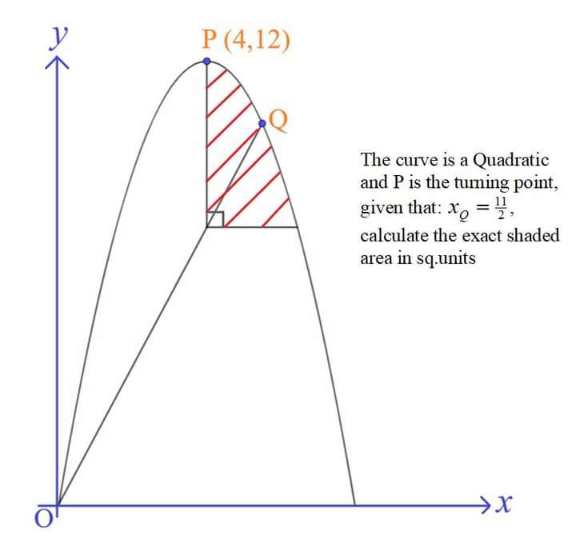
Question Number 152496 Answers: 1 Comments: 0

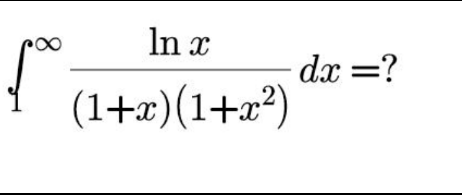
Question Number 152494 Answers: 1 Comments: 0

prove that :: Ω := ∫_(0 ) ^( ∞) (( e^( −x) .ln ((( 1)/( x)) ) sin ( x ))/(x )) dx = (( π)/( 8)) ( 2 γ +ln (2 ) ) ...■ m.n

$$ \\ $$$$\:\:\:\:{prove}\:{that}\::: \\ $$$$\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\Omega\::=\:\int_{\mathrm{0}\:} ^{\:\infty} \frac{\:\:{e}^{\:−{x}} .\mathrm{ln}\:\left(\frac{\:\mathrm{1}}{\:{x}}\:\right)\:{sin}\:\left(\:{x}\:\right)}{{x}\:}\:{dx}\:=\:\frac{\:\pi}{\:\mathrm{8}}\:\left(\:\mathrm{2}\:\gamma\:+\mathrm{ln}\:\left(\mathrm{2}\:\right)\:\right)\:...\blacksquare\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:{m}.{n} \\ $$$$ \\ $$

Question Number 152543 Answers: 2 Comments: 0

Solve the equation x^3 −3x=(√(x+2))

$$\:\:\mathrm{Solve}\:\mathrm{the}\:\mathrm{equation}\: \\ $$$$\:\mathrm{x}^{\mathrm{3}} −\mathrm{3x}=\sqrt{\mathrm{x}+\mathrm{2}} \\ $$

Question Number 152486 Answers: 1 Comments: 0

Question Number 152544 Answers: 2 Comments: 1

Find the real zeros of the polynomial P_a (x)=(x^2 +1)(x−1)^2 −ax^2 where a is a given real number

$$\:\mathrm{Find}\:\mathrm{the}\:\mathrm{real}\:\mathrm{zeros}\:\mathrm{of}\:\mathrm{the}\:\mathrm{polynomial} \\ $$$$\:\mathrm{P}_{\mathrm{a}} \left(\mathrm{x}\right)=\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{ax}^{\mathrm{2}} \\ $$$$\mathrm{where}\:\mathrm{a}\:\mathrm{is}\:\mathrm{a}\:\mathrm{given}\:\mathrm{real}\:\mathrm{number} \\ $$

Question Number 152481 Answers: 1 Comments: 1

Question Number 152478 Answers: 2 Comments: 0

Question Number 152472 Answers: 1 Comments: 0

Γ((3/4)) = exp(− ((3γ)/4) + ∫_0 ^( 1) f(x)dx) find f(x)

$$\: \\ $$$$\:\:\:\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)\:=\:\mathrm{exp}\left(−\:\frac{\mathrm{3}\gamma}{\mathrm{4}}\:+\:\int_{\mathrm{0}} ^{\:\mathrm{1}} {f}\left({x}\right){dx}\right) \\ $$$$\: \\ $$$$\:\:\:\:\mathrm{find}\:{f}\left({x}\right) \\ $$$$\: \\ $$

Question Number 152468 Answers: 1 Comments: 0

∫_(−∞) ^( ∞) ((ln((√(x^4 +1))))/( (√(x^4 +1)))) dx

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{−\infty} ^{\:\infty} \:\frac{\mathrm{ln}\left(\sqrt{{x}^{\mathrm{4}} +\mathrm{1}}\right)}{\:\sqrt{{x}^{\mathrm{4}} +\mathrm{1}}}\:\:{dx} \\ $$$$\: \\ $$

Question Number 152464 Answers: 1 Comments: 0

Question Number 152457 Answers: 2 Comments: 0

Kofi is 20% heavier than Afia. If Kofi weighs 60 kg what is Afia′s weight?

$$ \\ $$$$\mathrm{Kofi}\:\mathrm{is}\:\mathrm{20\%}\:\:\mathrm{heavier}\:\mathrm{than}\:\mathrm{Afia}.\:\mathrm{If}\:\mathrm{Kofi} \\ $$$$\mathrm{weighs}\:\mathrm{60}\:\mathrm{kg}\:\mathrm{what}\:\mathrm{is}\:\mathrm{Afia}'\mathrm{s}\:\mathrm{weight}? \\ $$

Question Number 152450 Answers: 1 Comments: 1

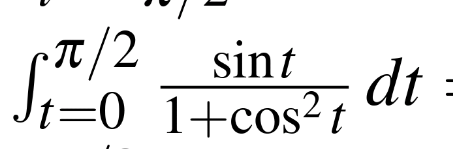
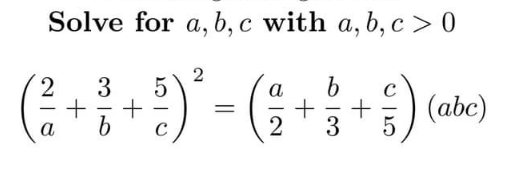
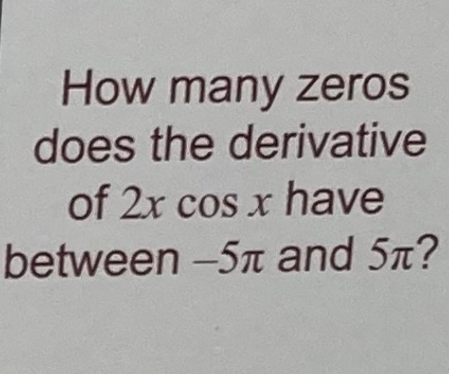
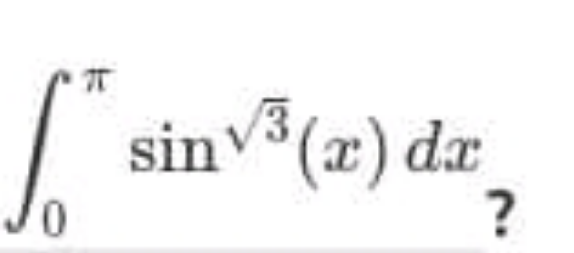
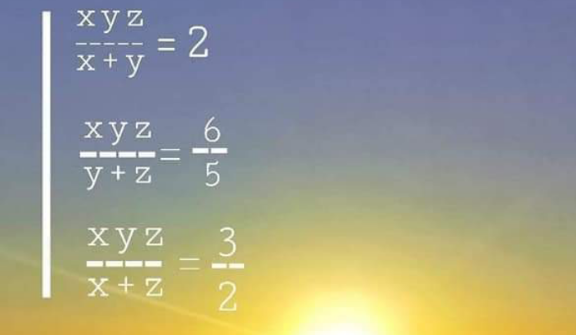
Question Number 152447 Answers: 1 Comments: 1

Question Number 152445 Answers: 0 Comments: 1

Question Number 152443 Answers: 0 Comments: 2

Question Number 152441 Answers: 3 Comments: 1

Question Number 152439 Answers: 0 Comments: 3

Question Number 152437 Answers: 1 Comments: 1

Question Number 152435 Answers: 1 Comments: 1

Question Number 152433 Answers: 1 Comments: 1

Question Number 152431 Answers: 0 Comments: 3

Pg 637 Pg 638 Pg 639 Pg 640 Pg 641 Pg 642 Pg 643 Pg 644 Pg 645 Pg 646

Terms of Service

Contact: info@tinkutara.com