
AllQuestion and Answers: Page 612
Question Number 157087 Answers: 0 Comments: 0
Question Number 157083 Answers: 0 Comments: 0

Question Number 157080 Answers: 3 Comments: 2

Question Number 157074 Answers: 2 Comments: 0
Question Number 157071 Answers: 0 Comments: 0
Question Number 157065 Answers: 0 Comments: 1
Question Number 157061 Answers: 2 Comments: 0
Question Number 157055 Answers: 1 Comments: 1
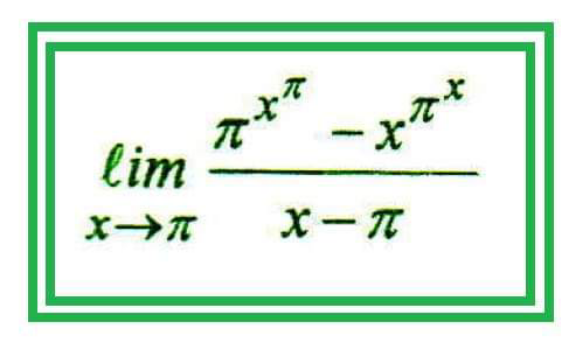
Question Number 157053 Answers: 0 Comments: 2
Question Number 157046 Answers: 0 Comments: 1
Question Number 157045 Answers: 1 Comments: 1
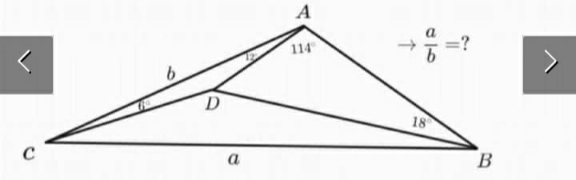
Question Number 157044 Answers: 1 Comments: 0
Question Number 157035 Answers: 1 Comments: 4

Question Number 157033 Answers: 3 Comments: 1
Question Number 157031 Answers: 0 Comments: 0
Question Number 157057 Answers: 1 Comments: 2
Question Number 157021 Answers: 1 Comments: 0
Question Number 157016 Answers: 1 Comments: 0
Question Number 157010 Answers: 0 Comments: 3
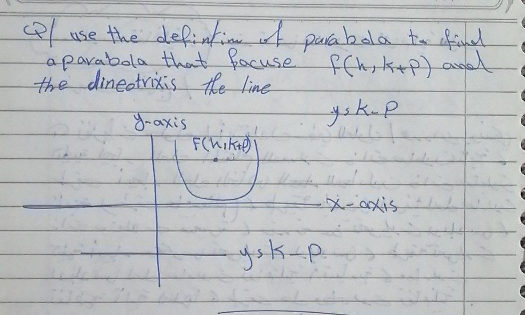
Question Number 157007 Answers: 1 Comments: 1

Question Number 157006 Answers: 1 Comments: 0
Question Number 156992 Answers: 1 Comments: 1

Question Number 156991 Answers: 1 Comments: 0
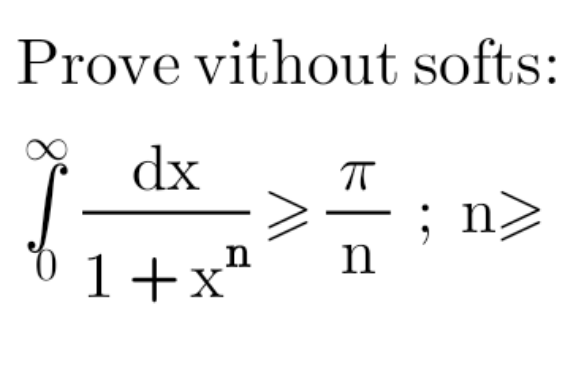
Question Number 156979 Answers: 2 Comments: 2

Question Number 156977 Answers: 0 Comments: 0
Question Number 156973 Answers: 0 Comments: 1

Pg 607 Pg 608 Pg 609 Pg 610 Pg 611 Pg 612 Pg 613 Pg 614 Pg 615 Pg 616
