Question and Answers Forum

AllQuestion and Answers: Page 611

Question Number 151294 Answers: 1 Comments: 0

Question Number 151287 Answers: 0 Comments: 2

I = ∫_(x=a) ^( x=b) (√(u^2 + v^2 x^2 − 2uvwx)) dx = ?

$${I}\:=\:\int_{{x}={a}} ^{\:{x}={b}} \sqrt{{u}^{\mathrm{2}} \:+\:{v}^{\mathrm{2}} {x}^{\mathrm{2}} \:−\:\mathrm{2}{uvwx}}\:{dx}\:=\:? \\ $$

Question Number 151284 Answers: 1 Comments: 0

if (a/3^(x-1) ) = (b/3^(y+2) ) = (c/3^(z-1) ) = (1/5) find abc = ?

$$\mathrm{if}\:\:\frac{\mathrm{a}}{\mathrm{3}^{\boldsymbol{\mathrm{x}}-\mathrm{1}} }\:=\:\frac{\mathrm{b}}{\mathrm{3}^{\boldsymbol{\mathrm{y}}+\mathrm{2}} }\:=\:\frac{\mathrm{c}}{\mathrm{3}^{\boldsymbol{\mathrm{z}}-\mathrm{1}} }\:=\:\frac{\mathrm{1}}{\mathrm{5}} \\ $$$$\mathrm{find}\:\:\mathrm{abc}\:=\:? \\ $$

Question Number 151282 Answers: 0 Comments: 0

Question Number 151281 Answers: 0 Comments: 0

Question Number 151278 Answers: 1 Comments: 0

∫ (e^(√(x - 1)) /( (√(x - 1)))) dx = ?

$$\int\:\frac{\boldsymbol{\mathrm{e}}^{\sqrt{\boldsymbol{\mathrm{x}}\:-\:\mathrm{1}}} }{\:\sqrt{\boldsymbol{\mathrm{x}}\:-\:\mathrm{1}}}\:\mathrm{dx}\:=\:? \\ $$

Question Number 151276 Answers: 2 Comments: 2

∫_0 ^(2π) cos^(−1) (cos x)dx

$$\int_{\mathrm{0}} ^{\mathrm{2}\pi} \mathrm{cos}^{−\mathrm{1}} \left(\mathrm{cos}\:\mathrm{x}\right)\mathrm{dx} \\ $$

Question Number 151272 Answers: 0 Comments: 1

Question Number 151269 Answers: 0 Comments: 1

Question Number 151268 Answers: 0 Comments: 0

Σ_(r=1) ^∞ (((−1)^(r−1) )/r)[ψ((r/2)+(1/4))−ψ((r/2)−(1/4))]

$$\underset{{r}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{r}−\mathrm{1}} }{{r}}\left[\psi\left(\frac{{r}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{4}}\right)−\psi\left(\frac{{r}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{4}}\right)\right] \\ $$

Question Number 151265 Answers: 1 Comments: 3

Question Number 151256 Answers: 2 Comments: 0

A_n =2^n +3^n +4^n +5^n B_n =100^n +101^n +102^n +103^n 1)find values of n while 7∣A_n 2) show that B_n ≡A_n [7 ]

$$\:{A}_{{n}} =\mathrm{2}^{{n}} +\mathrm{3}^{{n}} +\mathrm{4}^{{n}} +\mathrm{5}^{{n}} \\ $$$${B}_{{n}} =\mathrm{100}^{{n}} +\mathrm{101}^{{n}} +\mathrm{102}^{{n}} +\mathrm{103}^{{n}} \\ $$$$\left.\mathrm{1}\right)\boldsymbol{{find}}\:\boldsymbol{{values}}\:\boldsymbol{{of}}\:\boldsymbol{{n}}\:\boldsymbol{{while}}\:\mathrm{7}\mid\boldsymbol{{A}}_{\boldsymbol{{n}}} \\ $$$$\left.\mathrm{2}\right)\:\boldsymbol{{show}}\:\boldsymbol{{that}}\:\boldsymbol{{B}}_{\boldsymbol{{n}}} \equiv\boldsymbol{{A}}_{\boldsymbol{{n}}} \left[\mathrm{7}\:\right] \\ $$

Question Number 151248 Answers: 1 Comments: 0

Question Number 151247 Answers: 1 Comments: 0

(1/5) + (1/(5^2 ∙2)) + (1/(5^3 ∙3)) + (1/(5^4 ∙4)) + ... = ?

$$\frac{\mathrm{1}}{\mathrm{5}}\:+\:\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{2}} \centerdot\mathrm{2}}\:+\:\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{3}} \centerdot\mathrm{3}}\:+\:\frac{\mathrm{1}}{\mathrm{5}^{\mathrm{4}} \centerdot\mathrm{4}}\:+\:...\:=\:? \\ $$

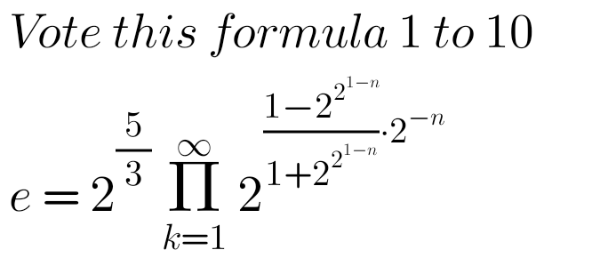
Question Number 151246 Answers: 1 Comments: 0

find I=∫_0 ^(π/4) ln(cosx)dx and J=∫_0 ^(π/4) ln(sinx)dx

$$\mathrm{find}\:\mathrm{I}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{cosx}\right)\mathrm{dx}\:\mathrm{and}\:\mathrm{J}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{sinx}\right)\mathrm{dx} \\ $$

Question Number 151241 Answers: 1 Comments: 0

Question Number 151230 Answers: 4 Comments: 2

prove: ∫_0 ^( ∞) (( ln ( 1+x^( 2) ))/(x^( 2) (1+x^( 2) )))dx= π ln((e/2) ) ..

$$ \\ $$$$\:\:\:\:{prove}: \\ $$$$\:\:\:\int_{\mathrm{0}} ^{\:\infty} \frac{\:{ln}\:\left(\:\mathrm{1}+{x}^{\:\mathrm{2}} \right)}{{x}^{\:\mathrm{2}} \left(\mathrm{1}+{x}^{\:\mathrm{2}} \right)}{dx}=\:\pi\:{ln}\left(\frac{{e}}{\mathrm{2}}\:\right)\:.. \\ $$

Question Number 151226 Answers: 0 Comments: 0

Question Number 151224 Answers: 1 Comments: 0

Question Number 151221 Answers: 1 Comments: 2

∫((sin x)/(sin (x−a)))dx

$$\int\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{sin}\:\left(\mathrm{x}−\mathrm{a}\right)}\mathrm{dx} \\ $$

Question Number 151220 Answers: 3 Comments: 0

show that ∫_0 ^(π/2) ((cos x)/(cos x+sin x+1))dx=(1/4)(π−2ln 2)

$$\mathrm{show}\:\mathrm{that}\: \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{cos}\:\mathrm{x}+\mathrm{sin}\:\mathrm{x}+\mathrm{1}}\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{4}}\left(\pi−\mathrm{2ln}\:\mathrm{2}\right) \\ $$

Question Number 151218 Answers: 0 Comments: 2

Question Number 151216 Answers: 1 Comments: 0

Solve for real number ((x+3))^(1/7) +((6−x))^(1/7) = (9)^(1/7)

$$\:\:\:\mathrm{Solve}\:\mathrm{for}\:\mathrm{real}\:\mathrm{number}\: \\ $$$$\:\:\sqrt[{\mathrm{7}}]{\mathrm{x}+\mathrm{3}}\:+\sqrt[{\mathrm{7}}]{\mathrm{6}−\mathrm{x}}\:=\:\sqrt[{\mathrm{7}}]{\mathrm{9}}\: \\ $$

Question Number 151215 Answers: 1 Comments: 0

if x;y;z>0 ; x+y+z=1 and λ≥(1/6) then: 𝛌 Σ ((y + z)/x) + 3 Σ yz ≥ 6𝛌 + 1

$$\mathrm{if}\:\:\mathrm{x};\mathrm{y};\mathrm{z}>\mathrm{0}\:;\:\mathrm{x}+\mathrm{y}+\mathrm{z}=\mathrm{1}\:\mathrm{and}\:\lambda\geqslant\frac{\mathrm{1}}{\mathrm{6}}\:\mathrm{then}: \\ $$$$\boldsymbol{\lambda}\:\Sigma\:\frac{\mathrm{y}\:+\:\mathrm{z}}{\mathrm{x}}\:+\:\mathrm{3}\:\Sigma\:\mathrm{yz}\:\geqslant\:\mathrm{6}\boldsymbol{\lambda}\:+\:\mathrm{1} \\ $$

Question Number 151212 Answers: 1 Comments: 0

if a_1 ,a_2 ,...a_n >1 then: (√(((a_1 -1)(a_2 -1)...(a_n -1))/((a_1 +1)(a_2 +1)...(a_n +1)))) ≤ ((a_1 a_2 ...a_n )/2^n )

$$\mathrm{if}\:\:\:\mathrm{a}_{\mathrm{1}} ,\mathrm{a}_{\mathrm{2}} ,...\mathrm{a}_{\boldsymbol{\mathrm{n}}} >\mathrm{1}\:\:\mathrm{then}: \\ $$$$\sqrt{\frac{\left(\mathrm{a}_{\mathrm{1}} -\mathrm{1}\right)\left(\mathrm{a}_{\mathrm{2}} -\mathrm{1}\right)...\left(\mathrm{a}_{\boldsymbol{\mathrm{n}}} -\mathrm{1}\right)}{\left(\mathrm{a}_{\mathrm{1}} +\mathrm{1}\right)\left(\mathrm{a}_{\mathrm{2}} +\mathrm{1}\right)...\left(\mathrm{a}_{\boldsymbol{\mathrm{n}}} +\mathrm{1}\right)}}\:\leqslant\:\frac{\mathrm{a}_{\mathrm{1}} \mathrm{a}_{\mathrm{2}} ...\mathrm{a}_{\boldsymbol{\mathrm{n}}} }{\mathrm{2}^{\boldsymbol{\mathrm{n}}} } \\ $$

Question Number 151211 Answers: 1 Comments: 0

Find the coefficient of x^9 from expression (1+x)(1+2x^2 )(1+3x^3 )(1+4x^4 )(1+5x^5 )...(1+10x^(10) )

$$\:{Find}\:{the}\:{coefficient}\:{of}\:{x}^{\mathrm{9}} \: \\ $$$${from}\:{expression}\: \\ $$$$\:\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\mathrm{2}{x}^{\mathrm{2}} \right)\left(\mathrm{1}+\mathrm{3}{x}^{\mathrm{3}} \right)\left(\mathrm{1}+\mathrm{4}{x}^{\mathrm{4}} \right)\left(\mathrm{1}+\mathrm{5}{x}^{\mathrm{5}} \right)...\left(\mathrm{1}+\mathrm{10}{x}^{\mathrm{10}} \right) \\ $$

Pg 606 Pg 607 Pg 608 Pg 609 Pg 610 Pg 611 Pg 612 Pg 613 Pg 614 Pg 615

Contact: info@tinkutara.com