Question and Answers Forum

AllQuestion and Answers: Page 610

Question Number 155977 Answers: 0 Comments: 0

Question Number 155972 Answers: 0 Comments: 0

Question Number 155973 Answers: 1 Comments: 2

f(x)=x^3 −3x^2 +4x−1 find a=? whenever f(a)=f^(−1) (a)

$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{3}} −\mathrm{3x}^{\mathrm{2}} +\mathrm{4x}−\mathrm{1} \\ $$$$\mathrm{find}\:\mathrm{a}=? \\ $$$$\mathrm{whenever}\:\:\:\:\mathrm{f}\left(\mathrm{a}\right)=\mathrm{f}^{−\mathrm{1}} \left(\mathrm{a}\right) \\ $$$$ \\ $$

Question Number 155969 Answers: 1 Comments: 0

Question Number 155965 Answers: 1 Comments: 0

(x sin (y/x)−y cos (y/x))dx+x cos (y/x) dy=0

$$\left({x}\:\mathrm{sin}\:\frac{{y}}{{x}}−{y}\:\mathrm{cos}\:\frac{{y}}{{x}}\right){dx}+{x}\:\mathrm{cos}\:\frac{{y}}{{x}}\:{dy}=\mathrm{0} \\ $$

Question Number 155959 Answers: 1 Comments: 0

quel est le changement de variable qui permet de passer de l′equation differentielle : x^2 y′′−3xy′+4y=0 a une equation lineaire d′ordre 2 coefficient comstant en z

$${quel}\:{est}\:{le}\:{changement}\:{de}\:{variable}\:{qui}\:{permet}\:{de}\:{passer} \\ $$$${de}\:{l}'{equation}\:{differentielle}\:: \\ $$$${x}^{\mathrm{2}} {y}''−\mathrm{3}{xy}'+\mathrm{4}{y}=\mathrm{0} \\ $$$${a}\:{une}\:{equation}\:{lineaire}\:{d}'{ordre}\:\mathrm{2}\:\:{coefficient}\:{comstant}\:\:{en}\:{z} \\ $$

Question Number 159716 Answers: 1 Comments: 0

Show that ▽r^n =nr^(n−2) r

$${Show}\:{that}\:\bigtriangledown{r}^{{n}} ={nr}^{{n}−\mathrm{2}} {r} \\ $$

Question Number 155951 Answers: 1 Comments: 0

solve : ⌊ (1/x) ⌋ + ⌊ (3/x) ⌋= 4

$$ \\ $$$$\:\:\:\:\:\:\:{solve}\:\:: \\ $$$$ \\ $$$$\:\:\:\:\:\lfloor\:\frac{\mathrm{1}}{{x}}\:\rfloor\:+\:\lfloor\:\frac{\mathrm{3}}{{x}}\:\rfloor=\:\mathrm{4}\: \\ $$$$ \\ $$

Question Number 155945 Answers: 1 Comments: 1

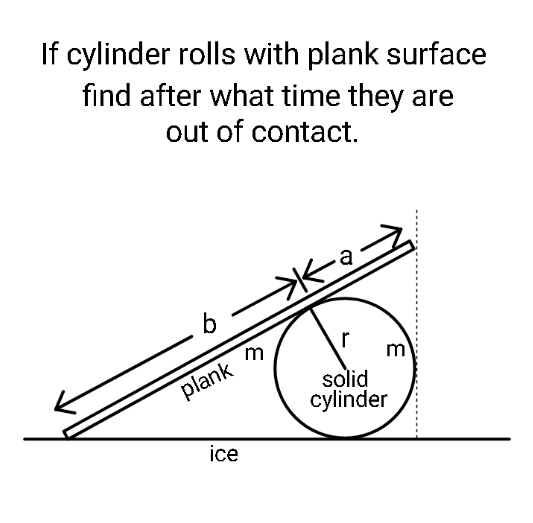
Question Number 155937 Answers: 0 Comments: 1

lim_(a→0) ((1−((cos 3a))^(1/3) (√(cos 2a)) cos a)/(a sin a cos 2a)) =?

$$\:\underset{\mathrm{a}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\sqrt[{\mathrm{3}}]{\mathrm{cos}\:\mathrm{3a}}\:\sqrt{\mathrm{cos}\:\mathrm{2a}}\:\mathrm{cos}\:\mathrm{a}}{\mathrm{a}\:\mathrm{sin}\:\mathrm{a}\:\mathrm{cos}\:\mathrm{2a}}\:=? \\ $$

Question Number 155933 Answers: 0 Comments: 1

∫_(−∞) ^( ∞) (((√(1+ (1/(x^2 +1)) )) )/(x^2 + x +1)) dx

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{−\infty} ^{\:\infty} \:\frac{\sqrt{\mathrm{1}+\:\frac{\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{1}}\:}\:}{{x}^{\mathrm{2}} +\:{x}\:+\mathrm{1}}\:{dx} \\ $$$$\: \\ $$

Question Number 155930 Answers: 1 Comments: 2

∫_0 ^( 1) ((sin(x)cos(x))/(sin^3 (x)+cos^3 (x))) dx

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \:\:\frac{\mathrm{sin}\left({x}\right)\mathrm{cos}\left({x}\right)}{\mathrm{sin}^{\mathrm{3}} \left({x}\right)+\mathrm{cos}^{\mathrm{3}} \left({x}\right)}\:{dx} \\ $$$$\: \\ $$

Question Number 155929 Answers: 0 Comments: 0

∫_1 ^( 2) ((√x^2 )/(ln(x^2 ))) dx

$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{\mathrm{1}} ^{\:\mathrm{2}} \:\frac{\sqrt{{x}^{\mathrm{2}} }}{\mathrm{ln}\left({x}^{\mathrm{2}} \right)}\:{dx} \\ $$$$\: \\ $$

Question Number 155928 Answers: 1 Comments: 0

Question Number 155927 Answers: 0 Comments: 1

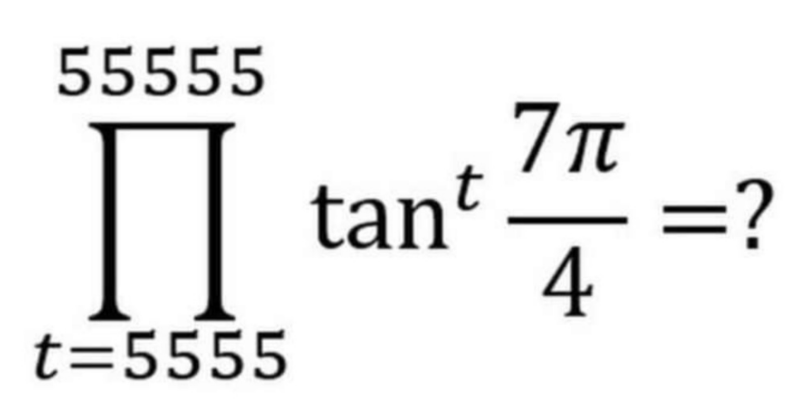
Question Number 155919 Answers: 1 Comments: 0

Question Number 155918 Answers: 0 Comments: 1

Can you evaluate this sum? Σ_(n=1) ^∞ 2^(−n) tan (2^(−n) )

$$\mathrm{Can}\:\mathrm{you}\:\mathrm{evaluate}\:\mathrm{this}\:\mathrm{sum}? \\ $$$$\underset{\mathrm{n}=\mathrm{1}} {\overset{\infty} {\sum}}\mathrm{2}^{−\mathrm{n}} \mathrm{tan}\:\left(\mathrm{2}^{−\mathrm{n}} \right) \\ $$

Question Number 155914 Answers: 0 Comments: 0

Draw the Newman projection formula for the chair conformation of cyclohexanol

$${D}\mathrm{raw}\:\mathrm{the}\:\mathrm{Newman}\:\mathrm{projection}\:\mathrm{formula} \\ $$$$\mathrm{for}\:\mathrm{the}\:\mathrm{chair}\:\mathrm{conformation}\:\:\mathrm{of}\:\mathrm{cyclohexanol} \\ $$

Question Number 155913 Answers: 0 Comments: 0

Question Number 155912 Answers: 0 Comments: 0

Determine the triangle with dimensions a;b;c∈N , a+b+c=even and A=P∈N. With maximum area. We denoted A=area and P=perimetr.

$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{triangle}\:\mathrm{with}\:\mathrm{dimensions} \\ $$$$\mathrm{a};\mathrm{b};\mathrm{c}\in\mathbb{N}\:,\:\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{even}\:\:\mathrm{and}\:\:\mathrm{A}=\mathrm{P}\in\mathbb{N}. \\ $$$$\mathrm{With}\:\mathrm{maximum}\:\mathrm{area}.\:\mathrm{We}\:\mathrm{denoted} \\ $$$$\mathrm{A}=\mathrm{area}\:\:\mathrm{and}\:\:\:\mathrm{P}=\mathrm{perimetr}. \\ $$

Question Number 155908 Answers: 0 Comments: 0

Solve for x 3^(x+1) +100=7^(x−1) 3^x +3^x^2 =2^x +4^x^2

$$\mathrm{Solve}\:\mathrm{for}\:{x} \\ $$$$\mathrm{3}^{{x}+\mathrm{1}} +\mathrm{100}=\mathrm{7}^{{x}−\mathrm{1}} \\ $$$$\mathrm{3}^{{x}} +\mathrm{3}^{{x}^{\mathrm{2}} } =\mathrm{2}^{{x}} +\mathrm{4}^{{x}^{\mathrm{2}} } \\ $$

Question Number 155910 Answers: 0 Comments: 0

Question Number 159719 Answers: 1 Comments: 0

F(x)= 3cos x + 4sin x , F^((101)) ((π/2))=?

$$\:\:\:\:\:{F}\left({x}\right)=\:\mathrm{3cos}\:{x}\:+\:\mathrm{4sin}\:{x}\:,\:{F}^{\left(\mathrm{101}\right)} \left(\frac{\pi}{\mathrm{2}}\right)=? \\ $$

Question Number 159717 Answers: 0 Comments: 0

Question Number 155897 Answers: 1 Comments: 0

∫ x(arctan x)^2 dx=?

$$\:\int\:\mathrm{x}\left(\mathrm{arctan}\:\mathrm{x}\right)^{\mathrm{2}} \:\mathrm{dx}=? \\ $$

Question Number 155893 Answers: 0 Comments: 0

Two Spheres are charged by +3μC and −3μC respectively.The charges are equally distributed in the serface of the sphere .The distance between two spheres is 100cm . Now if we Connect these two spheres with a conducting wire , a)What will be the potential difference between two ends of that wire? b)Find the amount of current flow in the wire if the resistance of that wire is 100μΩ .

$$\: \\ $$$$\:\:\mathrm{Two}\:\mathrm{Spheres}\:\mathrm{are}\:\mathrm{charged}\:\mathrm{by}\:+\mathrm{3}\mu\mathrm{C}\:\mathrm{and} \\ $$$$−\mathrm{3}\mu\mathrm{C}\:\mathrm{respectively}.\mathrm{The}\:\mathrm{charges}\:\mathrm{are}\: \\ $$$$\:\:\mathrm{equally}\:\mathrm{distributed}\:\mathrm{in}\:\mathrm{the}\:\mathrm{serface}\:\mathrm{of}\:\mathrm{the}\: \\ $$$$\:\:\mathrm{sphere}\:.\mathrm{The}\:\mathrm{distance}\:\mathrm{between}\:\mathrm{two}\: \\ $$$$\:\:\mathrm{spheres}\:\mathrm{is}\:\mathrm{100cm}\:.\:\mathrm{Now}\:\mathrm{if}\:\mathrm{we}\:\mathrm{Connect} \\ $$$$\:\:\mathrm{these}\:\mathrm{two}\:\mathrm{spheres}\:\mathrm{with}\:\mathrm{a}\:\mathrm{conducting}\: \\ $$$$\:\:\mathrm{wire}\:, \\ $$$$\left.\:\:\mathrm{a}\right)\mathrm{What}\:\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{potential}\:\mathrm{difference} \\ $$$$\:\mathrm{between}\:\mathrm{two}\:\mathrm{ends}\:\mathrm{of}\:\mathrm{that}\:\mathrm{wire}? \\ $$$$\left.\:\mathrm{b}\right)\mathrm{Find}\:\mathrm{the}\:\mathrm{amount}\:\mathrm{of}\:\mathrm{current}\:\mathrm{flow}\:\mathrm{in} \\ $$$$\:\mathrm{the}\:\mathrm{wire}\:\mathrm{if}\:\mathrm{the}\:\mathrm{resistance}\:\mathrm{of}\:\mathrm{that}\:\mathrm{wire}\: \\ $$$$\:\mathrm{is}\:\mathrm{100}\mu\Omega\:. \\ $$$$\: \\ $$

Pg 605 Pg 606 Pg 607 Pg 608 Pg 609 Pg 610 Pg 611 Pg 612 Pg 613 Pg 614

Contact: info@tinkutara.com