Question and Answers Forum

AllQuestion and Answers: Page 606

Question Number 158118 Answers: 0 Comments: 0

Question Number 158035 Answers: 1 Comments: 0

Question Number 158030 Answers: 1 Comments: 1

solve equation 5#+((√(((5!+5)/(5!!+5!!!))+!5))−5)$=2^x

$$\:\:\:\:{solve}\:{equation}\: \\ $$$$\:\:\:\mathrm{5}#+\left(\sqrt{\frac{\mathrm{5}!+\mathrm{5}}{\mathrm{5}!!+\mathrm{5}!!!}+!\mathrm{5}}−\mathrm{5}\right)\$=\mathrm{2}^{{x}} \\ $$

Question Number 158017 Answers: 0 Comments: 1

Question Number 158014 Answers: 4 Comments: 0

$$ \\ $$$$ \\ $$

Question Number 158009 Answers: 2 Comments: 0

$$ \\ $$$$ \\ $$

Question Number 158005 Answers: 0 Comments: 3

Question Number 158003 Answers: 0 Comments: 3

Question Number 157995 Answers: 0 Comments: 0

Given the following tetra− hedral figure. The length of the rib is 10 cm. Find the area of triangle DEF.

$$\mathrm{Given}\:\mathrm{the}\:\mathrm{following}\:\mathrm{tetra}− \\ $$$$\mathrm{hedral}\:\mathrm{figure}.\:\mathrm{The}\:\mathrm{length}\:\mathrm{of}\: \\ $$$$\mathrm{the}\:\mathrm{rib}\:\mathrm{is}\:\mathrm{10}\:\mathrm{cm}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{area} \\ $$$$\:\mathrm{of}\:\:\mathrm{triangle}\:\mathrm{DEF}. \\ $$$$ \\ $$$$ \\ $$

Question Number 157991 Answers: 1 Comments: 0

Length side of hexagonal above is =12 cm . Find it′s total area

$$ \\ $$$$ \\ $$$$\mathrm{Length}\:\mathrm{side}\:\mathrm{of}\:\mathrm{hexagonal}\:\mathrm{above}\:\mathrm{is}\:=\mathrm{12}\: \\ $$$$\mathrm{cm}\:.\:\mathrm{Find}\:\mathrm{it}'\mathrm{s}\:\mathrm{total}\:\mathrm{area} \\ $$$$ \\ $$

Question Number 157989 Answers: 0 Comments: 2

find the value of : Max_( x∈ R) ( (sin(x)+(√3) cos(x)+1)^( 2) =?

$$\: \\ $$$${find}\:{the}\:{value}\:{of}\:: \\ $$$$\: \\ $$$$\:\:\mathrm{Max}_{\:{x}\in\:\mathbb{R}} \:\left(\:\left(\mathrm{sin}\left({x}\right)+\sqrt{\mathrm{3}}\:{cos}\left({x}\right)+\mathrm{1}\right)^{\:\mathrm{2}} =?\right. \\ $$$$ \\ $$

Question Number 157984 Answers: 1 Comments: 0

Question Number 157982 Answers: 0 Comments: 0

(3x^2 −4xy+y^2 −3y)dx+(2xy−2x^2 +6y^2 +3x)dy=0

$$\:\left(\mathrm{3}{x}^{\mathrm{2}} −\mathrm{4}{xy}+{y}^{\mathrm{2}} −\mathrm{3}{y}\right){dx}+\left(\mathrm{2}{xy}−\mathrm{2}{x}^{\mathrm{2}} +\mathrm{6}{y}^{\mathrm{2}} +\mathrm{3}{x}\right){dy}=\mathrm{0} \\ $$

Question Number 157981 Answers: 1 Comments: 0

How much the long of the diagonal space , if total area of a cube is 216 cm^2 .

$$\mathrm{How}\:\mathrm{much}\:\mathrm{the}\:\mathrm{long}\:\mathrm{of}\:\mathrm{the}\:\mathrm{diagonal} \\ $$$$\:\mathrm{space}\:,\:\mathrm{if}\:\:\mathrm{total}\:\mathrm{area}\:\mathrm{of}\:\mathrm{a}\:\mathrm{cube}\:\mathrm{is} \\ $$$$\:\mathrm{216}\:\mathrm{cm}^{\mathrm{2}} . \\ $$

Question Number 157980 Answers: 2 Comments: 0

Calculate this problem below a). ((7!)/((5−1)!)) =...... b). ((5!×3!)/(4!)) =...... c). 6! 4! =... d). ((7!)/(3!))×((2!)/(5!)) =......

$$\mathrm{Calculate}\:\mathrm{this}\:\mathrm{problem}\:\mathrm{below} \\ $$$$\left.\:\:\:\:\:\:\:\:\mathrm{a}\right).\:\:\frac{\mathrm{7}!}{\left(\mathrm{5}−\mathrm{1}\right)!}\:=...... \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\mathrm{b}\right).\:\:\frac{\mathrm{5}!×\mathrm{3}!}{\mathrm{4}!}\:=...... \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\:\mathrm{c}\right).\:\:\mathrm{6}!\:\mathrm{4}!\:=...\:\:\:\:\:\:\:\: \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\:\mathrm{d}\right).\:\:\frac{\mathrm{7}!}{\mathrm{3}!}×\frac{\mathrm{2}!}{\mathrm{5}!}\:=...... \\ $$

Question Number 157977 Answers: 1 Comments: 0

∫ (dx/((1+(x)^(1/4) )(√x))) =?

$$\int\:\frac{{dx}}{\left(\mathrm{1}+\sqrt[{\mathrm{4}}]{{x}}\:\right)\sqrt{{x}}}\:=? \\ $$

Question Number 157974 Answers: 0 Comments: 4

which one do you prefer? sin^(−1) (x) or arcsin(x)

$$\mathrm{which}\:\mathrm{one}\:\mathrm{do}\:\mathrm{you}\:\mathrm{prefer}? \\ $$$$ \\ $$$$\mathrm{sin}^{−\mathrm{1}} \left({x}\right)\:\:\:\:\:\:\:\mathrm{or}\:\:\:\:\:\:\:\mathrm{arcsin}\left({x}\right) \\ $$

Question Number 157972 Answers: 1 Comments: 6

given 2x=3y and 4y=3z find ((3x−4y)/(2x−y+5z))

$$\:{given}\:\:\mathrm{2}{x}=\mathrm{3}{y}\:\:{and}\:\:\mathrm{4}{y}=\mathrm{3}{z} \\ $$$$\:{find}\:\:\:\frac{\mathrm{3}{x}−\mathrm{4}{y}}{\mathrm{2}{x}−{y}+\mathrm{5}{z}} \\ $$

Question Number 158037 Answers: 0 Comments: 0

(1) lim_(z→− i) ((z − i)/(z^2 + 1)) (2) lim_(z→− i) ((z + i)/(z^2 + 1)) (3) lim_(z→i) ((2z^2 − zi + 1)/(z − i))

$$\left(\mathrm{1}\right)\:\:\:\:\:\:\:\:\:\underset{\mathrm{z}\rightarrow−\:\mathrm{i}} {\mathrm{lim}}\:\:\frac{\mathrm{z}\:\:\:−\:\:\:\mathrm{i}}{\mathrm{z}^{\mathrm{2}} \:\:+\:\:\:\mathrm{1}} \\ $$$$\left(\mathrm{2}\right)\:\:\:\:\:\:\:\:\:\underset{\mathrm{z}\rightarrow−\:\mathrm{i}} {\mathrm{lim}}\:\:\frac{\mathrm{z}\:\:\:+\:\:\:\mathrm{i}}{\mathrm{z}^{\mathrm{2}} \:\:+\:\:\:\mathrm{1}} \\ $$$$\left(\mathrm{3}\right)\:\:\:\:\:\:\:\:\:\underset{\mathrm{z}\rightarrow\mathrm{i}} {\mathrm{lim}}\:\:\frac{\mathrm{2z}^{\mathrm{2}} \:\:\:−\:\:\:\mathrm{zi}\:\:\:+\:\:\:\mathrm{1}}{\mathrm{z}\:\:−\:\:\mathrm{i}} \\ $$

Question Number 157967 Answers: 0 Comments: 0

Prove that 7^(n+1) divide 3^7^n + 5^7^n - 1 for any positive integers n

$$\mathrm{Prove}\:\mathrm{that}\:\:\mathrm{7}^{\boldsymbol{\mathrm{n}}+\mathrm{1}} \:\:\mathrm{divide}\:\:\mathrm{3}^{\mathrm{7}^{\boldsymbol{\mathrm{n}}} } \:+\:\mathrm{5}^{\mathrm{7}^{\boldsymbol{\mathrm{n}}} } \:-\:\mathrm{1} \\ $$$$\mathrm{for}\:\mathrm{any}\:\mathrm{positive}\:\mathrm{integers}\:\:\boldsymbol{\mathrm{n}} \\ $$

Question Number 157965 Answers: 1 Comments: 1

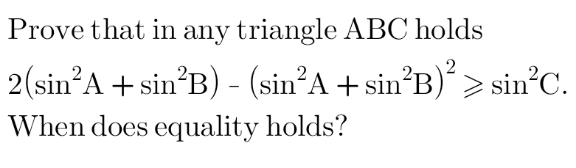
Question Number 157964 Answers: 0 Comments: 1

Solve for real numbers: 4tan(x) + ((sin(5x))/(cos^5 (x))) = 0

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{real}\:\mathrm{numbers}: \\ $$$$\mathrm{4tan}\left(\mathrm{x}\right)\:+\:\frac{\mathrm{sin}\left(\mathrm{5x}\right)}{\mathrm{cos}^{\mathrm{5}} \left(\mathrm{x}\right)}\:=\:\mathrm{0} \\ $$$$ \\ $$

Question Number 157961 Answers: 2 Comments: 0

prove that: I=∫_0 ^( ∞) x^( 2) tanh(x).e^( −x) dx=(π^( 3) /8) −2

$$ \\ $$$$\:\:\:\:\:\:\:{prove}\:{that}: \\ $$$$ \\ $$$$\mathrm{I}=\int_{\mathrm{0}} ^{\:\infty} {x}^{\:\mathrm{2}} {tanh}\left({x}\right).{e}^{\:−{x}} {dx}=\frac{\pi^{\:\mathrm{3}} }{\mathrm{8}}\:−\mathrm{2}\:\:\:\:\:\:\: \\ $$$$ \\ $$

Question Number 157957 Answers: 1 Comments: 3

Question Number 157958 Answers: 0 Comments: 0

Find: Ω_n =Σ_(n=1) ^∞ (1/n)(Σ_(k=1) ^n ((k^3 + k^2 - 3k - 2)/((k + 2)!)))

$$\mathrm{Find}: \\ $$$$\Omega_{\boldsymbol{\mathrm{n}}} =\underset{\boldsymbol{\mathrm{n}}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\mathrm{n}}\left(\underset{\boldsymbol{\mathrm{k}}=\mathrm{1}} {\overset{\boldsymbol{\mathrm{n}}} {\sum}}\frac{\mathrm{k}^{\mathrm{3}} \:+\:\mathrm{k}^{\mathrm{2}} \:-\:\mathrm{3k}\:-\:\mathrm{2}}{\left(\mathrm{k}\:+\:\mathrm{2}\right)!}\right) \\ $$$$ \\ $$

Question Number 157952 Answers: 1 Comments: 0

f(x)=x^(2014) +2x^(2013) +3x^(2012) +4x^(2011) +...+2014x+2015 min f(x)=?

$$\:{f}\left({x}\right)={x}^{\mathrm{2014}} +\mathrm{2}{x}^{\mathrm{2013}} +\mathrm{3}{x}^{\mathrm{2012}} +\mathrm{4}{x}^{\mathrm{2011}} +...+\mathrm{2014}{x}+\mathrm{2015} \\ $$$$\:{min}\:{f}\left({x}\right)=? \\ $$

Pg 601 Pg 602 Pg 603 Pg 604 Pg 605 Pg 606 Pg 607 Pg 608 Pg 609 Pg 610

Contact: info@tinkutara.com