Question and Answers Forum

AllQuestion and Answers: Page 605

Question Number 159447 Answers: 1 Comments: 1

#calculate# Ω := ∫_0 ^( 1) ∫_0 ^( 1) (( x^( (t/2)) −x^( t) )/(1 − x)) dx dt = ? −−−m.n−−−

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:#{calculate}# \\ $$$$\:\:\:\:\:\Omega\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \int_{\mathrm{0}} ^{\:\mathrm{1}} \:\frac{\:{x}^{\:\frac{{t}}{\mathrm{2}}} −{x}^{\:{t}} }{\mathrm{1}\:−\:{x}}\:{dx}\:{dt}\:=\:? \\ $$$$\:\:\:\:\:\:\:\:\:−−−{m}.{n}−−− \\ $$

Question Number 159428 Answers: 1 Comments: 0

(dy/dx)=cos(x+y)+sin(x+y)

$$\frac{{dy}}{{dx}}={cos}\left({x}+{y}\right)+{sin}\left({x}+{y}\right) \\ $$

Question Number 159425 Answers: 0 Comments: 0

Question Number 159421 Answers: 0 Comments: 4

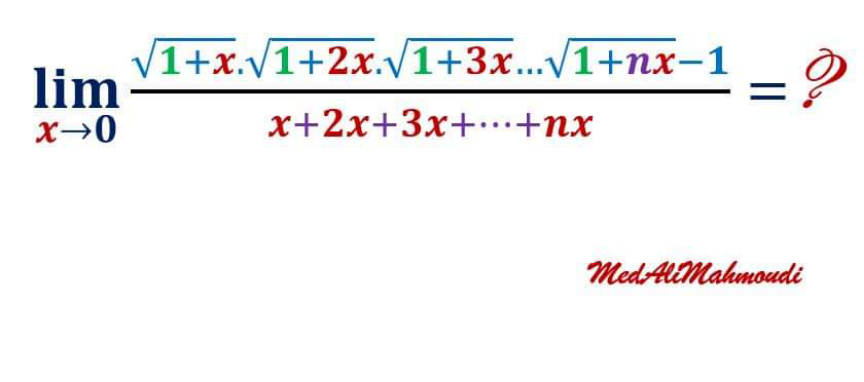
Question Number 159405 Answers: 1 Comments: 0

lim_(x→+0) (((Σ_(k=1) ^(2021) k^x )/(2021)))^(1/x) =?

$$\underset{\mathrm{x}\rightarrow+\mathrm{0}} {\mathrm{lim}}\left(\frac{\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{2021}} {\sum}}\mathrm{k}^{\mathrm{x}} }{\mathrm{2021}}\right)^{\frac{\mathrm{1}}{\mathrm{x}}} =? \\ $$

Question Number 159403 Answers: 0 Comments: 1

U_(n+1) =(1/2)(u_n +(a/u_n )) with u_1 >0, a>0 Prove that (u_(n+1) /u_n )≤1

$${U}_{{n}+\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}\left({u}_{{n}} +\frac{{a}}{{u}_{{n}} }\right)\:{with}\:{u}_{\mathrm{1}} >\mathrm{0},\:\:{a}>\mathrm{0} \\ $$$${Prove}\:{that}\:\:\frac{{u}_{{n}+\mathrm{1}} }{{u}_{{n}} }\leqslant\mathrm{1} \\ $$

Question Number 159396 Answers: 1 Comments: 0

Question Number 159395 Answers: 2 Comments: 0

lim_(x→0) ((1−((cos 2x))^(1/n) )/x^2 ) = (1/3) n=?

$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\sqrt[{{n}}]{\mathrm{cos}\:\mathrm{2}{x}}}{{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\:{n}=? \\ $$

Question Number 159394 Answers: 2 Comments: 2

if a^3 −b^3 =513, ab=54 than, a−b = ?

$$\mathrm{if}\:\mathrm{a}^{\mathrm{3}} −\mathrm{b}^{\mathrm{3}} =\mathrm{513},\:\mathrm{ab}=\mathrm{54} \\ $$$$\:\mathrm{than},\:\mathrm{a}−\mathrm{b}\:=\:? \\ $$

Question Number 159392 Answers: 1 Comments: 1

Question Number 159390 Answers: 1 Comments: 0

Find the relation between x and y if log_4 x +3=log_(27) y

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{relation}\:\mathrm{between}\:{x}\:\mathrm{and}\:{y}\:\mathrm{if} \\ $$$$\mathrm{log}_{\mathrm{4}} {x}\:+\mathrm{3}=\mathrm{log}_{\mathrm{27}} {y} \\ $$

Question Number 159388 Answers: 0 Comments: 0

lim_(x→∞) ((1/(x^2 +1))+(2/(x^2 +4))+(3/(x^2 +9))+…)=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{\mathrm{1}}{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{2}}{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\mathrm{4}}+\frac{\mathrm{3}}{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\mathrm{9}}+\ldots\right)=? \\ $$

Question Number 159386 Answers: 0 Comments: 0

Question Number 159379 Answers: 2 Comments: 0

let S(x) =Σ_(n=0) ^∞ (3x)^(n+2) using the sum above find: Σ_(n=0) ^∞ (((-1)^(n+1) )/(3^(n+1) (n + 3)))

$$\mathrm{let}\:\:\boldsymbol{\mathrm{S}}\left(\mathrm{x}\right)\:=\underset{\boldsymbol{\mathrm{n}}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{3x}\right)^{\boldsymbol{\mathrm{n}}+\mathrm{2}} \\ $$$$\mathrm{using}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{above}\:\mathrm{find}: \\ $$$$\underset{\boldsymbol{\mathrm{n}}=\mathrm{0}} {\overset{\infty} {\sum}}\:\frac{\left(-\mathrm{1}\right)^{\boldsymbol{\mathrm{n}}+\mathrm{1}} }{\mathrm{3}^{\boldsymbol{\mathrm{n}}+\mathrm{1}} \left(\mathrm{n}\:+\:\mathrm{3}\right)}\: \\ $$

Question Number 159378 Answers: 1 Comments: 2

let x;y>0 such that x^3 + y^3 = 2 find the minimum value of the following expression: P = 2020x + 2021y

$$\mathrm{let}\:\:\mathrm{x};\mathrm{y}>\mathrm{0}\:\:\mathrm{such}\:\mathrm{that}\:\:\mathrm{x}^{\mathrm{3}} \:+\:\mathrm{y}^{\mathrm{3}} \:=\:\mathrm{2} \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{minimum}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{following}\:\mathrm{expression}: \\ $$$$\mathrm{P}\:=\:\mathrm{2020}\boldsymbol{\mathrm{x}}\:+\:\mathrm{2021}\boldsymbol{\mathrm{y}} \\ $$

Question Number 159358 Answers: 0 Comments: 0

List all the assymptotes. List the domain and the x and y intercepts of f(x)= ((x^3 −x^2 +x−4)/(x^2 +2x−1))

$$\mathrm{List}\:\mathrm{all}\:\mathrm{the}\:\mathrm{assymptotes}. \\ $$$$\mathrm{List}\:\mathrm{the}\:\mathrm{domain}\:\mathrm{and}\:\mathrm{the}\:\mathrm{x}\:\mathrm{and}\:\mathrm{y}\:\mathrm{intercepts} \\ $$$$\mathrm{of}\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)=\:\frac{{x}^{\mathrm{3}} −{x}^{\mathrm{2}} +{x}−\mathrm{4}}{{x}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{1}} \\ $$

Question Number 159355 Answers: 1 Comments: 0

Question Number 159353 Answers: 0 Comments: 0

Question Number 159349 Answers: 1 Comments: 1

lim_(x→0) ((8sec x−8+tan^4 x−4tan^2 x)/x^6 ) =?

$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{8sec}\:{x}−\mathrm{8}+\mathrm{tan}\:^{\mathrm{4}} {x}−\mathrm{4tan}\:^{\mathrm{2}} {x}}{{x}^{\mathrm{6}} }\:=? \\ $$

Question Number 159346 Answers: 1 Comments: 0

xy′′+2(x+1)y′+(x+2)y=0

$${xy}''+\mathrm{2}\left({x}+\mathrm{1}\right){y}'+\left({x}+\mathrm{2}\right){y}=\mathrm{0} \\ $$

Question Number 159338 Answers: 1 Comments: 0

define increasing and decreasing function with example?

$${define}\:{increasing} \\ $$$${and}\:{decreasing}\:{function}\:{with}\:{example}? \\ $$

Question Number 159332 Answers: 0 Comments: 1

Question Number 159330 Answers: 1 Comments: 0

how to think from 1+2+3+...+n=((n(n+1))/2) 1^2 +2^2 +3^2 +...+n^2 =((n(n+1)(2n+1))/6) 1^3 +2^3 +3^3 +...+n^3 =(((n(n+1))/2))^2

$${how}\:{to}\:{think}\:{from}\: \\ $$$$\mathrm{1}+\mathrm{2}+\mathrm{3}+...+{n}=\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}} \\ $$$$\mathrm{1}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} +...+{n}^{\mathrm{2}} =\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{6}} \\ $$$$\mathrm{1}^{\mathrm{3}} +\mathrm{2}^{\mathrm{3}} +\mathrm{3}^{\mathrm{3}} +...+{n}^{\mathrm{3}} =\left(\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$

Question Number 159327 Answers: 4 Comments: 0

Question Number 159325 Answers: 1 Comments: 1

# Trigonometry# solve ( Equation) sin((x/2) ) − 2sin ((x/3) )= 0

$$ \\ $$$$\:\:\:\:\:\:#\:\mathrm{T}{rigonometry}# \\ $$$$\:\:\:\:\:\:\:{solve}\:\left(\:\:\:\mathscr{E}{quation}\right) \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:{sin}\left(\frac{{x}}{\mathrm{2}}\:\right)\:−\:\mathrm{2}{sin}\:\left(\frac{{x}}{\mathrm{3}}\:\right)=\:\mathrm{0}\:\:\:\:\:\:\:\:\: \\ $$$$ \\ $$$$ \\ $$

Question Number 159322 Answers: 1 Comments: 0

P(z)=(1+i(√3))z^2 −(−4+4i)z+2icos((π/5))−2sin((π/5)) Let S denote the sum of roots of P(z) a) Express S in algebraic form then in exponential form. b. Deduce the exact values of cos(((5π)/(12))) and sin(((5π)/(12))).

$$\mathrm{P}\left(\mathrm{z}\right)=\left(\mathrm{1}+{i}\sqrt{\mathrm{3}}\right){z}^{\mathrm{2}} −\left(−\mathrm{4}+\mathrm{4}{i}\right){z}+\mathrm{2}{i}\mathrm{cos}\left(\frac{\pi}{\mathrm{5}}\right)−\mathrm{2sin}\left(\frac{\pi}{\mathrm{5}}\right) \\ $$$$\mathrm{Let}\:{S}\:\mathrm{denote}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{P}\left({z}\right) \\ $$$$\left.\mathrm{a}\right)\:\mathrm{Express}\:{S}\:\mathrm{in}\:\mathrm{algebraic}\:\mathrm{form}\:\mathrm{then}\:\mathrm{in}\:\mathrm{exponential}\:\mathrm{form}. \\ $$$$\mathrm{b}.\:\mathrm{Deduce}\:\mathrm{the}\:\mathrm{exact}\:\mathrm{values}\:\mathrm{of}\:\mathrm{cos}\left(\frac{\mathrm{5}\pi}{\mathrm{12}}\right)\:\mathrm{and}\:\mathrm{sin}\left(\frac{\mathrm{5}\pi}{\mathrm{12}}\right). \\ $$

Pg 600 Pg 601 Pg 602 Pg 603 Pg 604 Pg 605 Pg 606 Pg 607 Pg 608 Pg 609

Contact: info@tinkutara.com