Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 604

Question Number 157631 Answers: 0 Comments: 0

what is higher order derivatives? discuss its importants.

$${what}\:{is}\:{higher}\:{order}\:{derivatives}? \\ $$$${discuss}\:{its}\:{importants}. \\ $$

Question Number 157630 Answers: 1 Comments: 1

Question Number 157652 Answers: 0 Comments: 4

Question Number 157628 Answers: 1 Comments: 0

find (C_0 ^(100) )^2 +(C_2 ^(100) )^2 +(C_4 ^(100) )^2 +(C_6 ^(100) )^2 +...+(C_(100) ^(100) )^2 =?

$${find} \\ $$$$\left({C}_{\mathrm{0}} ^{\mathrm{100}} \right)^{\mathrm{2}} +\left({C}_{\mathrm{2}} ^{\mathrm{100}} \right)^{\mathrm{2}} +\left({C}_{\mathrm{4}} ^{\mathrm{100}} \right)^{\mathrm{2}} +\left({C}_{\mathrm{6}} ^{\mathrm{100}} \right)^{\mathrm{2}} +...+\left({C}_{\mathrm{100}} ^{\mathrm{100}} \right)^{\mathrm{2}} =? \\ $$

Question Number 157611 Answers: 1 Comments: 0

Given g(x) = (1/(1 + 3^((1/2) − x) )) g((1/(2017))) + g((2/(2017))) + g((3/(2017))) + … + g(((2016)/(2017))) = ?

$${Given}\:\:{g}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{1}\:+\:\mathrm{3}^{\frac{\mathrm{1}}{\mathrm{2}}\:−\:{x}} } \\ $$$${g}\left(\frac{\mathrm{1}}{\mathrm{2017}}\right)\:+\:{g}\left(\frac{\mathrm{2}}{\mathrm{2017}}\right)\:+\:{g}\left(\frac{\mathrm{3}}{\mathrm{2017}}\right)\:+\:\ldots\:+\:{g}\left(\frac{\mathrm{2016}}{\mathrm{2017}}\right)\:\:=\:\:? \\ $$

Question Number 157604 Answers: 1 Comments: 3

Question find the” minimum” value of: f (x):= ∣1+x∣+∣ 2+x∣ + ∣4 +2x∣

$$ \\ $$$$\:\:\:\:\:\:\:\:\mathrm{Q}{uestion}\: \\ $$$$\:{find}\:{the}''\:{minimum}''\:{value}\:{of}: \\ $$$$ \\ $$$${f}\:\left({x}\right):=\:\mid\mathrm{1}+{x}\mid+\mid\:\mathrm{2}+{x}\mid\:+\:\mid\mathrm{4}\:+\mathrm{2}{x}\mid \\ $$$$ \\ $$

Question Number 157598 Answers: 1 Comments: 0

Given a,b,c nonnegative numbers which satisfy a+b+c = 3. Prove that (1/(2ab^2 + 1)) + (1/(2bc^2 +1)) + (1/(2ac^2 + 1)) ≥ 1 .

$${Given}\:\:{a},{b},{c}\:\:{nonnegative}\:\:{numbers}\:\:{which}\:\:{satisfy}\:\:\:{a}+{b}+{c}\:=\:\mathrm{3}. \\ $$$${Prove}\:\:{that}\:\: \\ $$$$\frac{\mathrm{1}}{\mathrm{2}{ab}^{\mathrm{2}} \:+\:\mathrm{1}}\:+\:\frac{\mathrm{1}}{\mathrm{2}{bc}^{\mathrm{2}} +\mathrm{1}}\:+\:\frac{\mathrm{1}}{\mathrm{2}{ac}^{\mathrm{2}} \:+\:\mathrm{1}}\:\geqslant\:\mathrm{1}\:. \\ $$

Question Number 157599 Answers: 1 Comments: 0

Find the number of x ∈ [1, 2016 ] , x ∈ N which making the expression 4x^6 + x^3 + 5 is divided by 11 .

$${Find}\:\:{the}\:\:{number}\:\:{of}\:\:{x}\:\in\:\left[\mathrm{1},\:\mathrm{2016}\:\right]\:\:,\:\:{x}\:\in\:\mathbb{N} \\ $$$${which}\:\:{making}\:\:{the}\:\:{expression}\:\:\mathrm{4}{x}^{\mathrm{6}} \:+\:\:{x}^{\mathrm{3}} \:+\:\mathrm{5}\:\:\:{is}\:\:{divided}\:\:\:{by}\:\:\mathrm{11}\:. \\ $$

Question Number 157593 Answers: 0 Comments: 0

let f:C→R z→min(y−[y],[y+1]−y) , y=Im(z) let w=e^(i((2π)/n)) , n∈N^∗ evaluate S_n =Σ_(0≤k<n) f(w^k )

$${let}\:{f}:{C}\rightarrow{R} \\ $$$$\:\:\:\:\:\:\:\:\:\:{z}\rightarrow{min}\left({y}−\left[{y}\right],\left[{y}+\mathrm{1}\right]−{y}\right)\:,\:{y}={Im}\left({z}\right) \\ $$$${let}\:{w}={e}^{{i}\frac{\mathrm{2}\pi}{{n}}} ,\:{n}\in{N}^{\ast} \\ $$$${evaluate}\:{S}_{{n}} =\underset{\mathrm{0}\leqslant{k}<{n}} {\sum}{f}\left({w}^{{k}} \right) \\ $$

Question Number 157592 Answers: 0 Comments: 0

let A={0^. ,1^. ,2^. } prove that every application from A to A is a 2nd degree polynom

$${let}\:{A}=\left\{\overset{.} {\mathrm{0}},\overset{.} {\mathrm{1}},\overset{.} {\mathrm{2}}\right\} \\ $$$${prove}\:{that}\:{every}\:{application}\:{from}\:{A}\: \\ $$$${to}\:{A}\:{is}\:{a}\:\mathrm{2}{nd}\:{degree}\:{polynom} \\ $$

Question Number 157591 Answers: 1 Comments: 0

lim_(x→π) ((x−π)^2 cos ((1/(x−π)))+x^4 +sin^3 x)=?

$$\:\underset{{x}\rightarrow\pi} {\mathrm{lim}}\:\left(\left({x}−\pi\right)^{\mathrm{2}} \mathrm{cos}\:\left(\frac{\mathrm{1}}{{x}−\pi}\right)+{x}^{\mathrm{4}} +\mathrm{sin}\:^{\mathrm{3}} {x}\right)=? \\ $$

Question Number 157585 Answers: 1 Comments: 0

if 0<a≤b≤c<(π/2) then: (5/(tana)) + (3/(tanb)) + (1/(tanc)) ≥ ((27)/(tana + tanb + tanc))

$$\mathrm{if}\:\:\:\mathrm{0}<\mathrm{a}\leqslant\mathrm{b}\leqslant\mathrm{c}<\frac{\pi}{\mathrm{2}}\:\:\:\mathrm{then}: \\ $$$$\frac{\mathrm{5}}{\mathrm{tan}\boldsymbol{\mathrm{a}}}\:+\:\frac{\mathrm{3}}{\mathrm{tan}\boldsymbol{\mathrm{b}}}\:+\:\frac{\mathrm{1}}{\mathrm{tan}\boldsymbol{\mathrm{c}}}\:\geqslant\:\frac{\mathrm{27}}{\mathrm{tan}\boldsymbol{\mathrm{a}}\:+\:\mathrm{tan}\boldsymbol{\mathrm{b}}\:+\:\mathrm{tan}\boldsymbol{\mathrm{c}}} \\ $$

Question Number 157584 Answers: 3 Comments: 0

suppose the ratio of Jim to Rohn is 2:1 and the ratio of Rohn to Bill is 3:4, how do i find for the ratio of Jim to Bill.....???

$${suppose}\: \\ $$$${the}\:{ratio}\:{of}\:{Jim}\:{to}\:{Rohn}\:{is}\:\mathrm{2}:\mathrm{1} \\ $$$$\:{and}\:{the}\:{ratio}\:{of}\:{Rohn}\:{to}\:{Bill}\:{is} \\ $$$$\:\mathrm{3}:\mathrm{4},\:{how}\:{do}\:{i}\:{find}\:{for}\:{the}\:{ratio} \\ $$$${of}\:{Jim}\:{to}\:{Bill}.....??? \\ $$

Question Number 157576 Answers: 1 Comments: 2

Question Number 157575 Answers: 1 Comments: 3

(√((1/2)−(1/2)(√((1/2)+(1/2)cos 𝛂)))) (Π<𝛂<2Π)

$$ \\ $$$$\sqrt{\frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}\:\boldsymbol{\alpha}}} \\ $$$$\left(\Pi<\boldsymbol{\alpha}<\mathrm{2}\Pi\right) \\ $$

Question Number 157565 Answers: 0 Comments: 0

Question Number 157562 Answers: 2 Comments: 0

Question Number 157560 Answers: 0 Comments: 0

Question Number 157561 Answers: 0 Comments: 0

if a;b;c and a+b+c≥3 prove that: Σ (a^3 /(b + kbc)) ≥ (3/(1 + k)) ; k>0

$$\mathrm{if}\:\:\mathrm{a};\mathrm{b};\mathrm{c}\:\:\mathrm{and}\:\:\mathrm{a}+\mathrm{b}+\mathrm{c}\geqslant\mathrm{3}\:\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\Sigma\:\frac{\mathrm{a}^{\mathrm{3}} }{\mathrm{b}\:+\:\mathrm{kbc}}\:\geqslant\:\frac{\mathrm{3}}{\mathrm{1}\:+\:\mathrm{k}}\:\:;\:\:\mathrm{k}>\mathrm{0}\: \\ $$

Question Number 157546 Answers: 1 Comments: 5

( _1 ^(2002) ) + ( _4 ^(2002) ) + ( _7 ^(2002) ) + …+ ( _(2002) ^(2002) ) = ? Thank you so much .

$$\left(\underset{\mathrm{1}} {\overset{\mathrm{2002}} {\:}}\right)\:+\:\left(\underset{\mathrm{4}} {\overset{\mathrm{2002}} {\:}}\right)\:+\:\left(\underset{\mathrm{7}} {\overset{\mathrm{2002}} {\:}}\right)\:+\:\ldots+\:\left(\underset{\mathrm{2002}} {\overset{\mathrm{2002}} {\:}}\right)\:\:=\:? \\ $$$${Thank}\:\:{you}\:\:{so}\:\:{much}\:. \\ $$

Question Number 157538 Answers: 1 Comments: 1

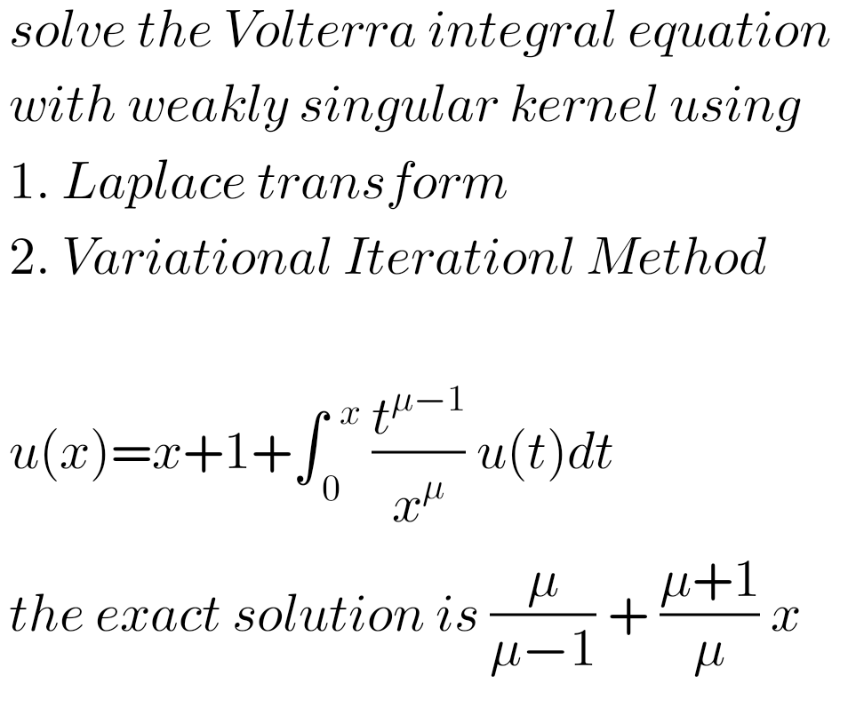
Question Number 157531 Answers: 2 Comments: 0

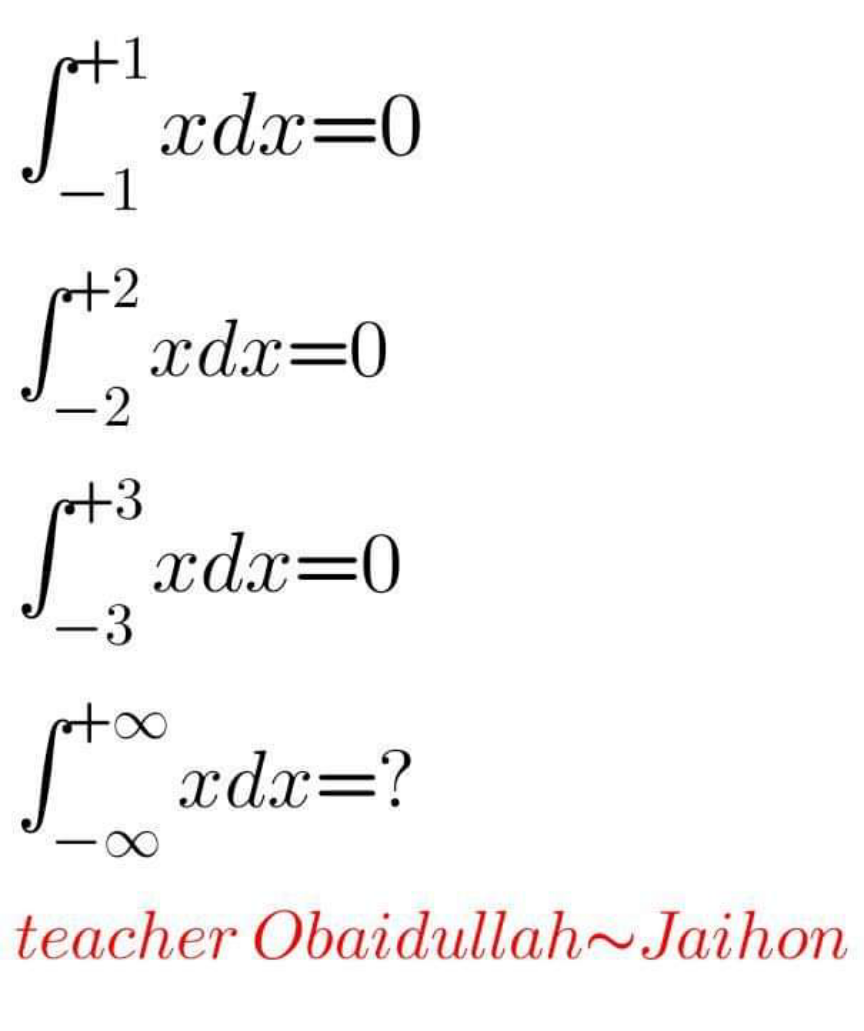
Question Number 157515 Answers: 0 Comments: 0

∫ ((x sin x)/(16x+9)) dx

$$\:\:\:\:\:\int\:\frac{{x}\:\mathrm{sin}\:{x}}{\mathrm{16}{x}+\mathrm{9}}\:{dx}\: \\ $$

Question Number 157508 Answers: 0 Comments: 1

Question Number 157494 Answers: 1 Comments: 0

Question Number 157487 Answers: 2 Comments: 0

Find the equation of the tangents drawn from the point (1,3) to the parabola y^2 =−16x.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{tangents}\:\mathrm{drawn} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{point}\:\left(\mathrm{1},\mathrm{3}\right)\:\mathrm{to}\:\mathrm{the}\:\mathrm{parabola} \\ $$$${y}^{\mathrm{2}} =−\mathrm{16}{x}. \\ $$

Pg 599 Pg 600 Pg 601 Pg 602 Pg 603 Pg 604 Pg 605 Pg 606 Pg 607 Pg 608

Contact: info@tinkutara.com