Question and Answers Forum

AllQuestion and Answers: Page 570

Question Number 160833 Answers: 1 Comments: 0

Σ_(k=1) ^∞ ((cos (ln k))/( (√k))) divergespnt or convergent?

$$\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{cos}\:\left(\mathrm{ln}\:{k}\right)}{\:\sqrt{{k}}} \\ $$$$\mathrm{divergespnt}\:\mathrm{or}\:\mathrm{convergent}? \\ $$

Question Number 160832 Answers: 1 Comments: 0

(√(2021−2(√(2021−2(√(2021−2x)))))) = x x=?

$$\:\sqrt{\mathrm{2021}−\mathrm{2}\sqrt{\mathrm{2021}−\mathrm{2}\sqrt{\mathrm{2021}−\mathrm{2x}}}}\:=\:\mathrm{x} \\ $$$$\:\mathrm{x}=? \\ $$

Question Number 160831 Answers: 0 Comments: 4

simplify ((2+(√5)))^(1/3)

$$\mathrm{simplify}\:\:\sqrt[{\mathrm{3}}]{\mathrm{2}+\sqrt{\mathrm{5}}} \\ $$

Question Number 160829 Answers: 0 Comments: 1

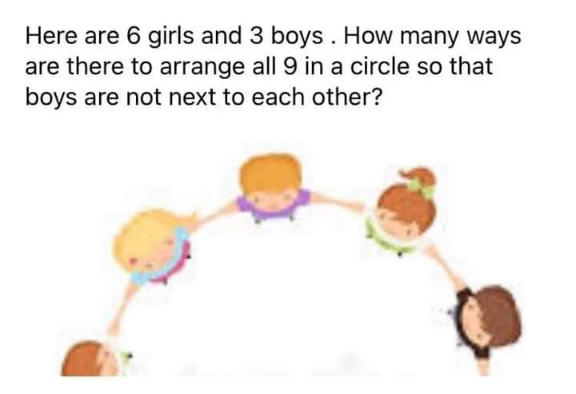
Question Number 160825 Answers: 1 Comments: 0

lim_(n→∞) [∫_0 ^1 (1+sin ((πt)/2))^n dt]^(1/n) =?

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\left[\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}+\mathrm{sin}\:\frac{\pi\mathrm{t}}{\mathrm{2}}\right)^{\mathrm{n}} \mathrm{dt}\right]^{\frac{\mathrm{1}}{\mathrm{n}}} =? \\ $$

Question Number 160823 Answers: 0 Comments: 1

Question Number 160822 Answers: 0 Comments: 0

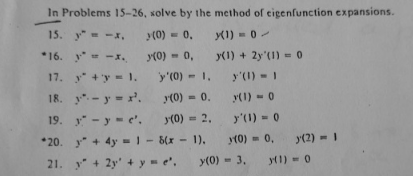
Question Number 160821 Answers: 1 Comments: 1

Question Number 160816 Answers: 0 Comments: 1

Question Number 160815 Answers: 1 Comments: 1

sec (3x)−6cos (3x)=4sin (3x) find the solution

$$\:\:\:\:\mathrm{sec}\:\left(\mathrm{3x}\right)−\mathrm{6cos}\:\left(\mathrm{3x}\right)=\mathrm{4sin}\:\left(\mathrm{3x}\right) \\ $$$$\:\:\:\:\mathrm{find}\:\mathrm{the}\:\mathrm{solution} \\ $$

Question Number 160796 Answers: 1 Comments: 0

lim_(n→∞) (√n)∫_(−∞) ^(+∞) ((cos x)/((1+x^2 )^n ))dx=?

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\sqrt{\mathrm{n}}\int_{−\infty} ^{+\infty} \frac{\mathrm{cos}\:\mathrm{x}}{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{n}} }\mathrm{dx}=? \\ $$

Question Number 160798 Answers: 0 Comments: 0

Question Number 160793 Answers: 2 Comments: 0

lim_(x→0) ((2^(cos x) − 2)/x^2 ) =?

$$\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2}^{\mathrm{cos}\:\mathrm{x}} \:−\:\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\:=? \\ $$

Question Number 160792 Answers: 2 Comments: 0

∫ ((sec x)/( (√(1+2sec x)))) (√((cosec x−cot x)/(cosec x+cot x))) dx =?

$$\:\:\:\int\:\frac{\mathrm{sec}\:\mathrm{x}}{\:\sqrt{\mathrm{1}+\mathrm{2sec}\:\mathrm{x}}}\:\sqrt{\frac{\mathrm{cosec}\:\mathrm{x}−\mathrm{cot}\:\mathrm{x}}{\mathrm{cosec}\:\mathrm{x}+\mathrm{cot}\:\mathrm{x}}}\:\mathrm{dx}\:=? \\ $$

Question Number 160777 Answers: 3 Comments: 3

3x^3 + x^2 + (m + 2)∙x + 4 = 0 equation root x_1 ; x_2 ; x_3 and x_1 = (1/x^2 ) + (1/x^3 ) find m=?

$$\mathrm{3x}^{\mathrm{3}} \:+\:\mathrm{x}^{\mathrm{2}} \:+\:\left(\mathrm{m}\:+\:\mathrm{2}\right)\centerdot\mathrm{x}\:+\:\mathrm{4}\:=\:\mathrm{0} \\ $$$$\mathrm{equation}\:\mathrm{root}\:\:\mathrm{x}_{\mathrm{1}} \:;\:\mathrm{x}_{\mathrm{2}} \:;\:\mathrm{x}_{\mathrm{3}} \\ $$$$\mathrm{and}\:\:\mathrm{x}_{\mathrm{1}} \:=\:\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{3}} } \\ $$$$\mathrm{find}\:\:\boldsymbol{\mathrm{m}}=? \\ $$

Question Number 160768 Answers: 0 Comments: 1

x^3 −3x−18 = 0 x∈R , x=?

$$\:\:\mathrm{x}^{\mathrm{3}} −\mathrm{3x}−\mathrm{18}\:=\:\mathrm{0}\: \\ $$$$\:\mathrm{x}\in\mathbb{R}\:,\:\mathrm{x}=? \\ $$

Question Number 160767 Answers: 1 Comments: 0

∫_( 0) ^( (π/2)) ((cos^2 x)/(cos^2 x+4sin^2 x)) dx =?

$$\:\:\:\:\int_{\:\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}}{\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}+\mathrm{4sin}\:^{\mathrm{2}} \mathrm{x}}\:\mathrm{dx}\:=?\: \\ $$

Question Number 160764 Answers: 0 Comments: 1

If log(((x^3 −y^3 )/(x^3 +y^3 ))), then (dy/dx)=?

$$\mathrm{If}\:\:\:\:\mathrm{log}\left(\frac{{x}^{\mathrm{3}} −{y}^{\mathrm{3}} }{{x}^{\mathrm{3}} +{y}^{\mathrm{3}} }\right),\:\mathrm{then}\:\frac{{dy}}{{dx}}=? \\ $$

Question Number 160762 Answers: 0 Comments: 5

sin 10+sin 20+sin 30+sin 40+∙∙∙∙+sin 360=?

$$\mathrm{sin}\:\mathrm{10}+\mathrm{sin}\:\mathrm{20}+\mathrm{sin}\:\mathrm{30}+\mathrm{sin}\:\mathrm{40}+\centerdot\centerdot\centerdot\centerdot+\mathrm{sin}\:\mathrm{360}=? \\ $$

Question Number 160746 Answers: 1 Comments: 0

Question Number 160744 Answers: 1 Comments: 1

(x^2 +x−12)^3 +(x^2 +3x−18)^2 = 9(x^2 −9)^2 x=?

$$\:\:\:\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}−\mathrm{12}\right)^{\mathrm{3}} +\left(\mathrm{x}^{\mathrm{2}} +\mathrm{3x}−\mathrm{18}\right)^{\mathrm{2}} =\:\mathrm{9}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{9}\right)^{\mathrm{2}} \\ $$$$\:\mathrm{x}=?\: \\ $$

Question Number 160739 Answers: 0 Comments: 3

nature de cette integrale quequ′en soit le reel α ∫_1 ^(+oo) t^α e^(−t) dt

$$\:{nature}\:{de}\:{cette}\:{integrale}\:{quequ}'{en}\:{soit}\:{le}\:{reel}\:\alpha \\ $$$$\int_{\mathrm{1}} ^{+{oo}} {t}^{\alpha} {e}^{−{t}} {dt} \\ $$

Question Number 160747 Answers: 1 Comments: 1

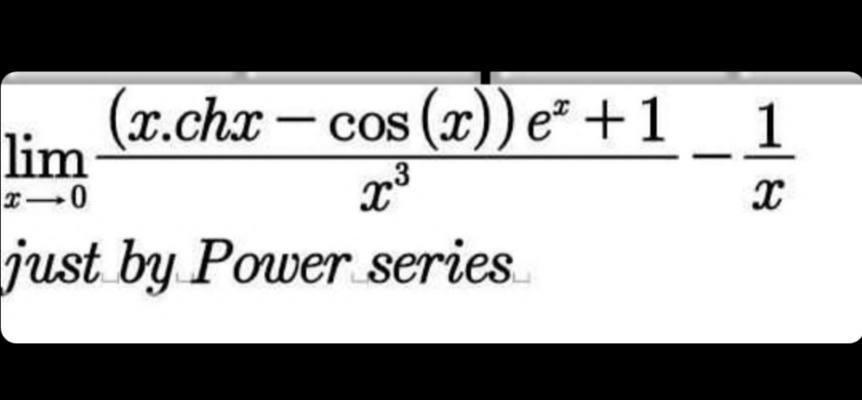
Question Number 160734 Answers: 0 Comments: 1

# Advanced Calculus # Φ = ∫_0 ^( 1) (((ln^ ( (1/(1− x)) ))/x) )^( 3) dx =^? 3 ( ζ (2 ) + ζ (3 )) −−−− solution−−−− Φ =^(I.B.P) [ (( 1)/(2x^( 2) )) ln^( 3) ( 1−x)]_0 ^1 +(3/2) ∫_0 ^( 1) (( ln^( 2) (1− x ))/(x^( 2) (1 − x ))) dx = (1/2) lim_( ξ →1^(− ) ) ((ln^( 3) ( 1− ξ ))/ξ^( 2) ) +(3/2)[∫_0 ^( 1) (( ln^( 2) ( 1− x ))/x)dx = 2 ζ (3)] + (3/2)[∫_0 ^( 1) (( ln^( 2) ( 1−x))/x^( 2) ) dx = (π^( 2) /3) = 2ζ (2 )] +(3/2)∫_0 ^( 1) (( ln^( 2) (1− x))/(1−x)) dx} =(1/2) lim_( ξ →1^( −) ) {((ln^( 3) ( 1−ξ ))/ξ^( 2) ) −ln^( 3) (1− ξ ) } +(3/2) (2ζ (3 )) +(3/2) ( 2ζ (2 )) = 3( ζ (3 ) + 3ζ (2 ) ) ■ m.n

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:#\:\mathrm{Advanced}\:\:\:\mathrm{Calculus}\:# \\ $$$$\:\:\:\:\:\:\:\:\Phi\:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \left(\frac{{ln}^{\:} \:\left(\:\frac{\mathrm{1}}{\mathrm{1}−\:{x}}\:\:\right)}{{x}}\:\right)^{\:\mathrm{3}} {dx}\:\overset{?} {=}\:\mathrm{3}\:\left(\:\zeta\:\left(\mathrm{2}\:\right)\:+\:\zeta\:\left(\mathrm{3}\:\right)\right) \\ $$$$\:\:\:\:\:\:−−−−\:\:{solution}−−−− \\ $$$$\:\:\:\:\:\:\:\:\Phi\:\overset{\mathrm{I}.\mathrm{B}.\mathrm{P}} {=}\:\left[\:\frac{\:\mathrm{1}}{\mathrm{2}{x}^{\:\mathrm{2}} }\:{ln}^{\:\mathrm{3}} \left(\:\mathrm{1}−{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} +\frac{\mathrm{3}}{\mathrm{2}}\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:{ln}^{\:\mathrm{2}} \:\left(\mathrm{1}−\:{x}\:\right)}{{x}^{\:\mathrm{2}} \:\left(\mathrm{1}\:−\:{x}\:\right)}\:{dx} \\ $$$$\:\:\:\:\:\:\:\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:{lim}_{\:\xi\:\rightarrow\mathrm{1}^{−\:} } \:\frac{{ln}^{\:\mathrm{3}} \left(\:\mathrm{1}−\:\xi\:\right)}{\xi^{\:\mathrm{2}} }\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:+\frac{\mathrm{3}}{\mathrm{2}}\left[\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:{ln}^{\:\mathrm{2}} \left(\:\mathrm{1}−\:{x}\:\right)}{{x}}{dx}\:=\:\mathrm{2}\:\zeta\:\left(\mathrm{3}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:+\:\frac{\mathrm{3}}{\mathrm{2}}\left[\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:{ln}^{\:\mathrm{2}} \left(\:\mathrm{1}−{x}\right)}{{x}^{\:\mathrm{2}} }\:{dx}\:=\:\frac{\pi^{\:\mathrm{2}} }{\mathrm{3}}\:=\:\mathrm{2}\zeta\:\left(\mathrm{2}\:\right)\right]\: \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:\:\:+\frac{\mathrm{3}}{\mathrm{2}}\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\:{ln}^{\:\mathrm{2}} \left(\mathrm{1}−\:{x}\right)}{\mathrm{1}−{x}}\:{dx}\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\:{lim}_{\:\xi\:\rightarrow\mathrm{1}^{\:−} } \left\{\frac{{ln}^{\:\mathrm{3}} \left(\:\mathrm{1}−\xi\:\right)}{\xi^{\:\mathrm{2}} }\:\:−{ln}^{\:\mathrm{3}} \left(\mathrm{1}−\:\xi\:\right)\:\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:+\frac{\mathrm{3}}{\mathrm{2}}\:\left(\mathrm{2}\zeta\:\left(\mathrm{3}\:\right)\right)\:\:+\frac{\mathrm{3}}{\mathrm{2}}\:\left(\:\mathrm{2}\zeta\:\left(\mathrm{2}\:\right)\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\:\:\mathrm{3}\left(\:\:\:\zeta\:\left(\mathrm{3}\:\right)\:+\:\mathrm{3}\zeta\:\left(\mathrm{2}\:\right)\:\:\right)\:\:\:\:\:\:\:\blacksquare\:\:\:{m}.{n}\:\:\: \\ $$$$ \\ $$

Question Number 160733 Answers: 0 Comments: 0

Question Number 160755 Answers: 0 Comments: 0

Pg 565 Pg 566 Pg 567 Pg 568 Pg 569 Pg 570 Pg 571 Pg 572 Pg 573 Pg 574

Contact: info@tinkutara.com