
AllQuestion and Answers: Page 568
Question Number 160938 Answers: 1 Comments: 0
Question Number 160937 Answers: 1 Comments: 0
Question Number 160933 Answers: 1 Comments: 0
Question Number 160931 Answers: 1 Comments: 0
$$\:\:{x}\:=\:\mathrm{2}^{\mathrm{log}\:_{\mathrm{5}} \left({x}+\mathrm{3}\right)} \:;\:{x}=? \\ $$
Question Number 160928 Answers: 1 Comments: 0
Question Number 160924 Answers: 1 Comments: 1

Question Number 160923 Answers: 0 Comments: 1
Question Number 160922 Answers: 1 Comments: 0
Question Number 160921 Answers: 0 Comments: 0
Question Number 160917 Answers: 1 Comments: 0
Question Number 160912 Answers: 1 Comments: 0
Question Number 160910 Answers: 1 Comments: 0

Question Number 160909 Answers: 2 Comments: 0
Question Number 160908 Answers: 0 Comments: 1
Question Number 160906 Answers: 0 Comments: 0
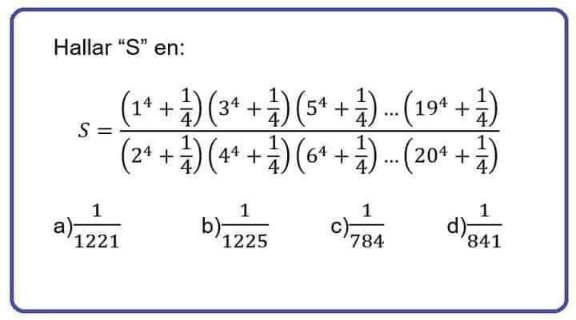
Question Number 160903 Answers: 2 Comments: 0
Question Number 160902 Answers: 1 Comments: 0
Question Number 160900 Answers: 0 Comments: 2
Question Number 160895 Answers: 1 Comments: 0
Question Number 160894 Answers: 1 Comments: 0

Question Number 160886 Answers: 0 Comments: 0
Question Number 160885 Answers: 0 Comments: 0
Question Number 160883 Answers: 1 Comments: 0
Question Number 160875 Answers: 1 Comments: 0
Question Number 160873 Answers: 2 Comments: 0

Question Number 160871 Answers: 1 Comments: 0
Pg 563 Pg 564 Pg 565 Pg 566 Pg 567 Pg 568 Pg 569 Pg 570 Pg 571 Pg 572
