
AllQuestion and Answers: Page 564
Question Number 161664 Answers: 1 Comments: 0
Question Number 161660 Answers: 3 Comments: 0
Question Number 161656 Answers: 1 Comments: 3
Question Number 161652 Answers: 0 Comments: 0
Question Number 161703 Answers: 2 Comments: 1
Question Number 161646 Answers: 0 Comments: 0

Question Number 161644 Answers: 0 Comments: 2
Question Number 161629 Answers: 0 Comments: 0

Question Number 161626 Answers: 3 Comments: 1

Question Number 161668 Answers: 1 Comments: 0
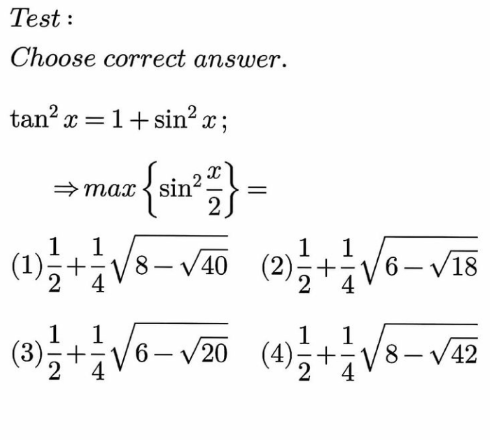
Question Number 161623 Answers: 2 Comments: 0
Question Number 161622 Answers: 1 Comments: 0
Question Number 161617 Answers: 2 Comments: 0

Question Number 161612 Answers: 2 Comments: 0
Question Number 161609 Answers: 0 Comments: 0
Question Number 161590 Answers: 2 Comments: 0
Question Number 161586 Answers: 2 Comments: 2
Question Number 161583 Answers: 3 Comments: 1
Question Number 161578 Answers: 1 Comments: 1
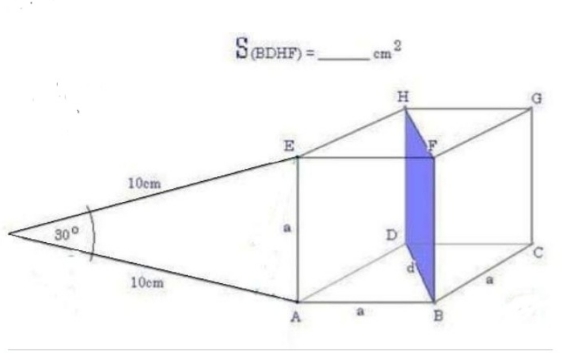
Question Number 161579 Answers: 1 Comments: 1
Question Number 161575 Answers: 1 Comments: 0
$$\int_{\mathrm{0}} ^{\mathrm{9}} \frac{\sqrt{{x}}}{\mathrm{1}−{x}}{dx}=? \\ $$$$ \\ $$
Question Number 161764 Answers: 2 Comments: 5

Question Number 161567 Answers: 2 Comments: 0
Question Number 161566 Answers: 0 Comments: 0
Question Number 161564 Answers: 1 Comments: 1
Question Number 161563 Answers: 1 Comments: 0
Pg 559 Pg 560 Pg 561 Pg 562 Pg 563 Pg 564 Pg 565 Pg 566 Pg 567 Pg 568
