Question and Answers Forum

AllQuestion and Answers: Page 562

Question Number 153714 Answers: 2 Comments: 0

How many three−digit numbers can be formed using the digits 2,3,5,7,8 if the number is odd and no digit is repeted?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{three}−\mathrm{digit}\:\mathrm{numbers}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{formed}\:\mathrm{using}\:\mathrm{the}\:\mathrm{digits}\:\mathrm{2},\mathrm{3},\mathrm{5},\mathrm{7},\mathrm{8}\:\mathrm{if}\: \\ $$$$\mathrm{the}\:\mathrm{number}\:\mathrm{is}\:\mathrm{odd}\:\mathrm{and}\:\mathrm{no}\:\mathrm{digit}\:\mathrm{is}\:\mathrm{repeted}? \\ $$

Question Number 153708 Answers: 2 Comments: 0

Question Number 153704 Answers: 1 Comments: 1

Question Number 153696 Answers: 1 Comments: 0

Question Number 153867 Answers: 0 Comments: 2

Question Number 153698 Answers: 2 Comments: 0

solve in R { ((x^3 −y^3 =19)),((xy=6)) :}

$${solve}\:{in}\:\mathbb{R} \\ $$$$\begin{cases}{{x}^{\mathrm{3}} −{y}^{\mathrm{3}} =\mathrm{19}}\\{{xy}=\mathrm{6}}\end{cases} \\ $$

Question Number 153685 Answers: 3 Comments: 2

Question Number 153682 Answers: 1 Comments: 0

((i)^(1/i) )^(xi) = i^x x=?

$$\:\left(\sqrt[{{i}}]{{i}}\:\right)^{{xi}} \:=\:{i}^{{x}} \: \\ $$$$\:\:{x}=?\: \\ $$

Question Number 153681 Answers: 1 Comments: 0

24^(log _(10) (x)) −26^(log _(10) (x)) =1 x=?

$$\:\:\mathrm{24}^{\mathrm{log}\:_{\mathrm{10}} \left({x}\right)} −\mathrm{26}^{\mathrm{log}\:_{\mathrm{10}} \left({x}\right)} =\mathrm{1} \\ $$$$\:{x}=? \\ $$

Question Number 153679 Answers: 1 Comments: 0

Find the constant of polynom P(11x−2) if given the equation 3P(x+2)−P(2x+3)=−4x^2 −x+3

$${Find}\:{the}\:{constant}\:{of}\:{polynom} \\ $$$$\:{P}\left(\mathrm{11}{x}−\mathrm{2}\right)\:{if}\:{given}\:{the}\:{equation} \\ $$$$\mathrm{3}{P}\left({x}+\mathrm{2}\right)−{P}\left(\mathrm{2}{x}+\mathrm{3}\right)=−\mathrm{4}{x}^{\mathrm{2}} −{x}+\mathrm{3} \\ $$

Question Number 153676 Answers: 2 Comments: 0

Question Number 153671 Answers: 0 Comments: 1

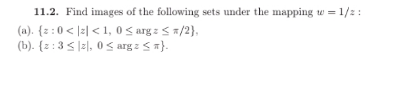
Question Number 153668 Answers: 0 Comments: 0

probability question let T_(V ) be the volume of tetrahedron T that is in a cone C with volume C_V find P(T_V ≥ (1/8) C_V )

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\underline{\mathrm{probability}\:\mathrm{question}} \\ $$$$\: \\ $$$$\:\mathrm{let}\:{T}_{{V}\:} \:\mathrm{be}\:\mathrm{the}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{tetrahedron}\:{T}\:\:\: \\ $$$$\:\mathrm{that}\:\mathrm{is}\:\mathrm{in}\:\mathrm{a}\:\mathrm{cone}\:{C}\:\mathrm{with}\:\mathrm{volume}\:{C}_{{V}} \\ $$$$\:\mathrm{find}\:\mathrm{P}\left({T}_{{V}} \:\:\geqslant\:\frac{\mathrm{1}}{\mathrm{8}}\:{C}_{{V}} \right) \\ $$$$\: \\ $$

Question Number 153657 Answers: 0 Comments: 2

let d(n) denote the sum of the digits of n. i.e d(1000) = 1 , d(999) = 27 find minimum k such that d(d(....._(k times) d(5^(10^(100) ) ).....) ≪ 10

$$\: \\ $$$$\:\mathrm{let}\:{d}\left({n}\right)\:\mathrm{denote}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{digits}\:\: \\ $$$$\:\mathrm{of}\:{n}. \\ $$$$\:\mathrm{i}.\mathrm{e}\:\:\:\:{d}\left(\mathrm{1000}\right)\:=\:\mathrm{1}\:,\:\:\:\:\:{d}\left(\mathrm{999}\right)\:=\:\mathrm{27} \\ $$$$\: \\ $$$$\:\mathrm{find}\:\mathrm{minimum}\:{k}\:\mathrm{such}\:\mathrm{that} \\ $$$$\:\underset{{k}\:\mathrm{times}} {\underbrace{{d}\left({d}\left(.....}{d}}\left(\mathrm{5}^{\mathrm{10}^{\mathrm{100}} } \right).....\right)\:\ll\:\mathrm{10}\right. \\ $$$$\: \\ $$

Question Number 153651 Answers: 2 Comments: 0

Question Number 153638 Answers: 1 Comments: 2

Question Number 153629 Answers: 0 Comments: 3

Question Number 153628 Answers: 1 Comments: 0

A ball is thrown vertically upwards. Its hight(h)meters at time( t)seconds is given by h= 5+30t−5t^2 . Find the maximum hight reached by the ball.

$$\mathrm{A}\:\mathrm{ball}\:\mathrm{is}\:\mathrm{thrown}\:\mathrm{vertically}\:\mathrm{upwards}. \\ $$$$\mathrm{Its}\:\mathrm{hight}\left(\mathrm{h}\right)\mathrm{meters}\:\mathrm{at}\:\mathrm{time}\left(\:\mathrm{t}\right)\mathrm{seconds} \\ $$$$\mathrm{is}\:\mathrm{given}\:\mathrm{by}\:\mathrm{h}=\:\mathrm{5}+\mathrm{30t}−\mathrm{5t}^{\mathrm{2}} .\:\mathrm{Find}\:\mathrm{the} \\ $$$$\mathrm{maximum}\:\mathrm{hight}\:\mathrm{reached}\:\mathrm{by}\:\mathrm{the}\:\mathrm{ball}. \\ $$

Question Number 153626 Answers: 1 Comments: 0

Ten eggs are picked at random without replacement from a lot containing 20% defective eggs. Find the probability that there are at least four defective eggs

$$\mathrm{Ten}\:\mathrm{eggs}\:\mathrm{are}\:\mathrm{picked}\:\mathrm{at}\:\mathrm{random}\:\mathrm{without} \\ $$$$\mathrm{replacement}\:\mathrm{from}\:\mathrm{a}\:\mathrm{lot}\:\mathrm{containing}\: \\ $$$$\mathrm{20\%}\:\mathrm{defective}\:\mathrm{eggs}.\: \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{that}\:\mathrm{there}\:\mathrm{are}\: \\ $$$$\mathrm{at}\:\mathrm{least}\:\mathrm{four}\:\mathrm{defective}\:\mathrm{eggs} \\ $$

Question Number 153616 Answers: 0 Comments: 1

Question Number 153605 Answers: 1 Comments: 2

{ (((√x) +(√(y+z)) =5)),(((√y)+(√(z+x)) = 7)),(((√z)+(√(x+y)) = 7)) :}

$$\:\:\begin{cases}{\sqrt{\mathrm{x}}\:+\sqrt{\mathrm{y}+\mathrm{z}}\:=\mathrm{5}}\\{\sqrt{\mathrm{y}}+\sqrt{\mathrm{z}+\mathrm{x}}\:=\:\mathrm{7}}\\{\sqrt{\mathrm{z}}+\sqrt{\mathrm{x}+\mathrm{y}}\:=\:\mathrm{7}}\end{cases} \\ $$

Question Number 153598 Answers: 0 Comments: 3

lim_(x→∞) cos (nπ (e)^(1/(2n)) )=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}cos}\:\left({n}\pi\:\sqrt[{\mathrm{2}{n}}]{{e}}\:\right)=? \\ $$

Question Number 153736 Answers: 1 Comments: 0

Given that 7 cos 2θ+24 sin^2 θ=R cos(2θ−α), where R>0 and 0<α<(π/2), find the maximum value of 14 cos^2 θ+48 sin θ cos θ.

$$\mathrm{Given}\:\mathrm{that}\:\mathrm{7}\:\mathrm{cos}\:\mathrm{2}\theta+\mathrm{24}\:\mathrm{sin}^{\mathrm{2}} \theta={R}\:\mathrm{cos}\left(\mathrm{2}\theta−\alpha\right), \\ $$$$\mathrm{where}\:{R}>\mathrm{0}\:\mathrm{and}\:\mathrm{0}<\alpha<\frac{\pi}{\mathrm{2}},\:\mathrm{find}\:\mathrm{the}\:\mathrm{maximum} \\ $$$$\mathrm{value}\:\mathrm{of}\:\mathrm{14}\:\mathrm{cos}^{\mathrm{2}} \theta+\mathrm{48}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta. \\ $$

Question Number 153593 Answers: 1 Comments: 0

Solve : (sin(2x))! = 2

$$\boldsymbol{{Solve}}\::\:\left(\boldsymbol{{sin}}\left(\mathrm{2}\boldsymbol{{x}}\right)\right)!\:=\:\mathrm{2} \\ $$

Question Number 153611 Answers: 3 Comments: 0

Question Number 153596 Answers: 0 Comments: 5

Pg 557 Pg 558 Pg 559 Pg 560 Pg 561 Pg 562 Pg 563 Pg 564 Pg 565 Pg 566

Contact: info@tinkutara.com