Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 556

Question Number 162959 Answers: 1 Comments: 5

solve the differential equation y = x + p^3

$${solve}\:{the}\:{differential}\:{equation}\:{y}\:=\:{x}\:+\:{p}^{\mathrm{3}} \\ $$

Question Number 162952 Answers: 0 Comments: 0

Question Number 162951 Answers: 1 Comments: 2

4men clear a farm for 8 days and are paid 24$ How long will 6 men take to clear the same farm if they are paid 360$ ?

$$\mathrm{4men}\:\mathrm{clear}\:\mathrm{a}\:\mathrm{farm}\:\mathrm{for}\:\mathrm{8}\:\mathrm{days}\:\mathrm{and}\:\mathrm{are}\:\mathrm{paid}\:\mathrm{24\$} \\ $$$$\mathrm{How}\:\mathrm{long}\:\mathrm{will}\:\mathrm{6}\:\mathrm{men}\:\mathrm{take}\:\mathrm{to}\:\mathrm{clear}\:\mathrm{the}\:\mathrm{same}\:\mathrm{farm} \\ $$$$\mathrm{if}\:\mathrm{they}\:\mathrm{are}\:\mathrm{paid}\:\mathrm{360\$}\:? \\ $$

Question Number 162949 Answers: 3 Comments: 1

Question Number 162947 Answers: 0 Comments: 0

Question Number 162946 Answers: 1 Comments: 0

Question Number 162939 Answers: 0 Comments: 0

lim_( x→ 3) ( a ⌊x ⌋ + ⌊ −x⌋).tan(((πx)/2) )=−∞ a ∈ ?

$$ \\ $$$$\:\:{lim}_{\:{x}\rightarrow\:\mathrm{3}} \:\left(\:{a}\:\lfloor{x}\:\rfloor\:+\:\lfloor\:−{x}\rfloor\right).{tan}\left(\frac{\pi{x}}{\mathrm{2}}\:\right)=−\infty \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{a}\:\in\:? \\ $$$$ \\ $$

Question Number 162942 Answers: 2 Comments: 0

How many positive integers less than 500 can be formed using the numbers 1 , 2 , 3 and 5 for the digits?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{positive}\:\mathrm{integers}\:\mathrm{less}\:\mathrm{than} \\ $$$$\mathrm{500}\:\mathrm{can}\:\mathrm{be}\:\mathrm{formed}\:\mathrm{using}\:\mathrm{the}\:\mathrm{numbers} \\ $$$$\mathrm{1}\:,\:\mathrm{2}\:,\:\mathrm{3}\:\mathrm{and}\:\mathrm{5}\:\mathrm{for}\:\mathrm{the}\:\mathrm{digits}? \\ $$

Question Number 162941 Answers: 0 Comments: 0

Question Number 162926 Answers: 0 Comments: 0

lim_(x→0) (1/x^(n+1) )[(1+x+(x/2)+...+(x^n /n))^(1/(x+(x/2)+...+(x^n /n))) −(1+x+(x/2)+...+(x^(n+1) /(n+1)))^(1/(x+(x/2)+...+(x^(n+1) /(n+1)))) ]=?

$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{n}+\mathrm{1}} }\left[\left(\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}}{\mathrm{2}}+...+\frac{\mathrm{x}^{\mathrm{n}} }{\mathrm{n}}\right)^{\frac{\mathrm{1}}{\mathrm{x}+\frac{\mathrm{x}}{\mathrm{2}}+...+\frac{\mathrm{x}^{\mathrm{n}} }{\mathrm{n}}}} −\left(\mathrm{1}+\mathrm{x}+\frac{\mathrm{x}}{\mathrm{2}}+...+\frac{\mathrm{x}^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}\right)^{\frac{\mathrm{1}}{\mathrm{x}+\frac{\mathrm{x}}{\mathrm{2}}+...+\frac{\mathrm{x}^{\mathrm{n}+\mathrm{1}} }{\mathrm{n}+\mathrm{1}}}} \right]=? \\ $$

Question Number 162925 Answers: 1 Comments: 0

lim_(x→0) (1/x^4 )[(1+x+(x^2 /2)+(x^3 /3))^(1/(x+(x^2 /2)+(x^3 /3))) −(1+x+(x^2 /2)+(x^3 /3)+(x^4 /4))^(1/(x+(x^2 /2)+(x^3 /3)+(x^4 /4))) ]=?

Question Number 162924 Answers: 2 Comments: 0

𝛗 =∫_0 ^( ∞) (( e^( −x^( 2) ) .ln( x ))/( (√x))) dx=λ Γ((1/4)) λ=? ■

$$\: \\ $$$$\:\boldsymbol{\phi}\:=\int_{\mathrm{0}} ^{\:\infty} \frac{\:{e}^{\:−{x}^{\:\mathrm{2}} } .\mathrm{ln}\left(\:{x}\:\right)}{\:\sqrt{{x}}}\:{dx}=\lambda\:\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right) \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\lambda=?\:\:\:\:\:\:\:\:\:\:\:\:\:\blacksquare \\ $$$$ \\ $$

Question Number 162894 Answers: 1 Comments: 0

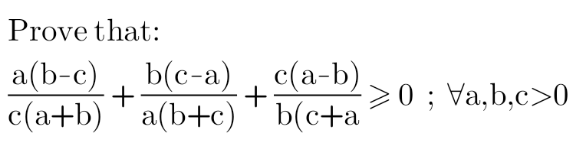
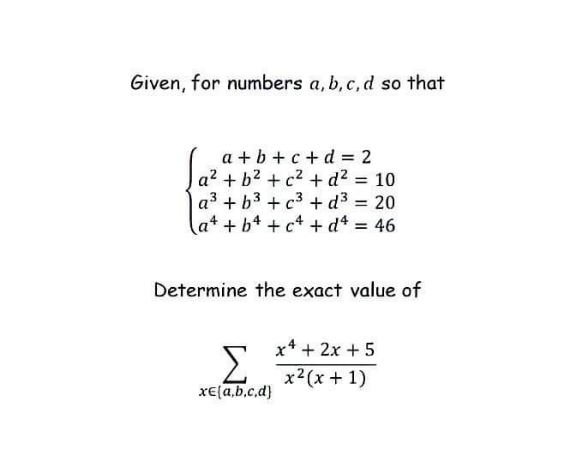
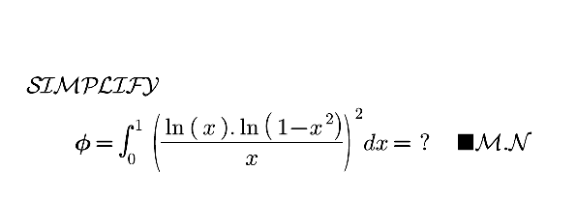
Question Number 162893 Answers: 2 Comments: 0

Ω=∫_0 ^( 1) ((( x^ )/(ln^ ( 1−x ))))^( 2) dx=^? ln ((( 27)/(16)) ) −−−−

$$ \\ $$$$\:\:\:\:\:\Omega=\int_{\mathrm{0}} ^{\:\mathrm{1}} \left(\frac{\:{x}^{\:} }{\mathrm{ln}^{\:} \left(\:\mathrm{1}−{x}\:\right)}\right)^{\:\mathrm{2}} {dx}\overset{?} {=}\:\mathrm{ln}\:\left(\frac{\:\mathrm{27}}{\mathrm{16}}\:\right) \\ $$$$\:\:\:\:\:\:\:\:−−−− \\ $$$$ \\ $$

Question Number 162877 Answers: 2 Comments: 0

Find: 𝛀 = ∫_( 0) ^( 1) (x^3 /(ln^2 (1 - x))) dx

$$\mathrm{Find}: \\ $$$$\boldsymbol{\Omega}\:\:=\:\underset{\:\mathrm{0}} {\overset{\:\mathrm{1}} {\int}}\:\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{ln}^{\mathrm{2}} \:\left(\mathrm{1}\:-\:\mathrm{x}\right)}\:\mathrm{dx} \\ $$

Question Number 162876 Answers: 0 Comments: 0

Prove that: ∫_( 0) ^( (𝛑/2)) (xcotx ∙ lncos^2 x + ln^2 cosx)dx = (π^3 /(24))

$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\underset{\:\mathrm{0}} {\overset{\:\frac{\boldsymbol{\pi}}{\mathrm{2}}} {\int}}\:\left(\mathrm{xcot}\boldsymbol{\mathrm{x}}\:\centerdot\:\mathrm{lncos}^{\mathrm{2}} \boldsymbol{\mathrm{x}}\:+\:\mathrm{ln}^{\mathrm{2}} \mathrm{cos}\boldsymbol{\mathrm{x}}\right)\mathrm{dx}\:=\:\frac{\pi^{\mathrm{3}} }{\mathrm{24}} \\ $$

Question Number 162872 Answers: 1 Comments: 1

Question Number 162866 Answers: 1 Comments: 0

Question Number 162865 Answers: 0 Comments: 4

Question Number 162864 Answers: 2 Comments: 0

Question Number 162860 Answers: 2 Comments: 0

Question Number 162859 Answers: 0 Comments: 0

Prove that: ∫_( 0) ^( (𝛑/2)) ((e^(cos 2x) ∙ sin(x + sin 2x))/(sin x)) dx = ((πe)/2)

$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\underset{\:\mathrm{0}} {\overset{\:\frac{\boldsymbol{\pi}}{\mathrm{2}}} {\int}}\:\frac{\mathrm{e}^{\boldsymbol{\mathrm{cos}}\:\mathrm{2}\boldsymbol{\mathrm{x}}} \:\centerdot\:\mathrm{sin}\left(\mathrm{x}\:+\:\mathrm{sin}\:\mathrm{2x}\right)}{\mathrm{sin}\:\mathrm{x}}\:\mathrm{dx}\:=\:\frac{\pi{e}}{\mathrm{2}} \\ $$

Question Number 162856 Answers: 0 Comments: 0

Question Number 162854 Answers: 0 Comments: 0

let a;b;c≥0 and a+b+c=3 prove that: ((a - 1)/( (√(b + 3)))) + ((b - 1)/( (√(c + 3)))) + ((c - 1)/( (√(a + 3)))) ≥ 0

$$\mathrm{let}\:\:\mathrm{a};\mathrm{b};\mathrm{c}\geqslant\mathrm{0}\:\:\mathrm{and}\:\:\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{3}\:\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\frac{\mathrm{a}\:-\:\mathrm{1}}{\:\sqrt{\mathrm{b}\:+\:\mathrm{3}}}\:+\:\frac{\mathrm{b}\:-\:\mathrm{1}}{\:\sqrt{\mathrm{c}\:+\:\mathrm{3}}}\:+\:\frac{\mathrm{c}\:-\:\mathrm{1}}{\:\sqrt{\mathrm{a}\:+\:\mathrm{3}}}\:\geqslant\:\mathrm{0} \\ $$

Question Number 162847 Answers: 2 Comments: 0

Question Number 162845 Answers: 1 Comments: 1

Pg 551 Pg 552 Pg 553 Pg 554 Pg 555 Pg 556 Pg 557 Pg 558 Pg 559 Pg 560

Contact: info@tinkutara.com